Find the equation of the hyperbola whose conjugate axis is 5 and the distance between the foci is 13.
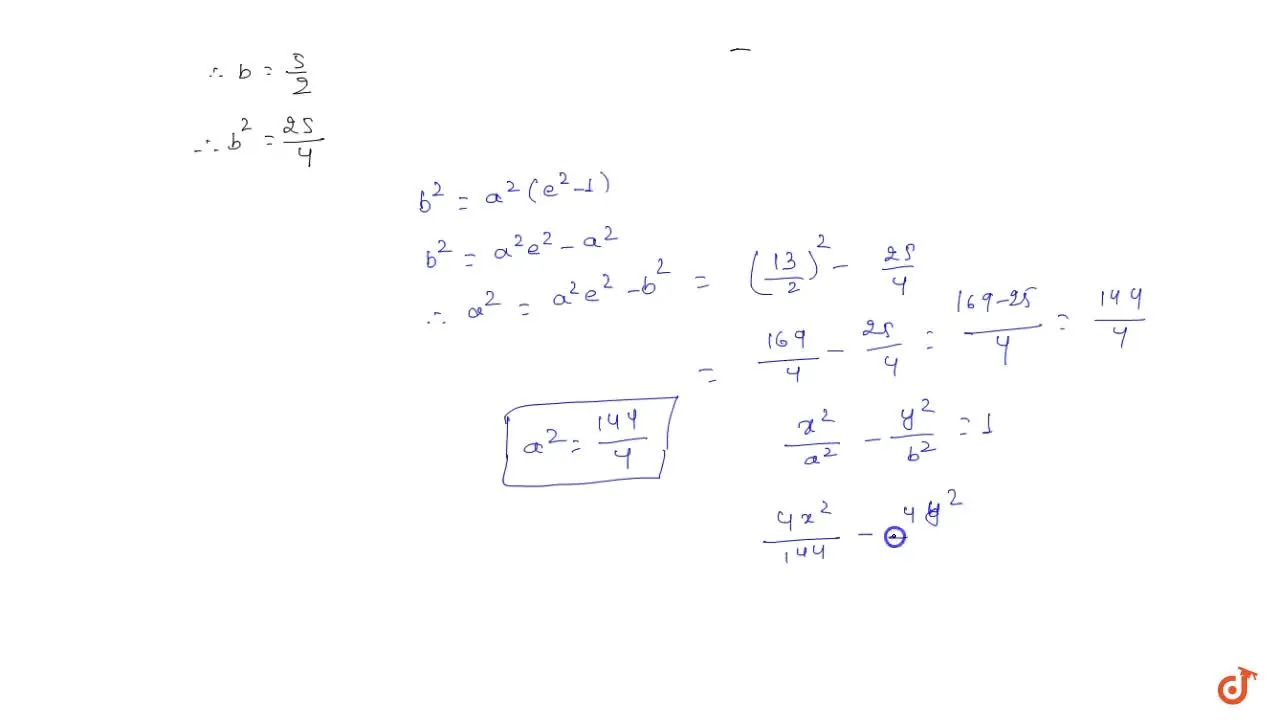
Length of congugate axis of hyperbola =5
⇒2b=5⇒b=54
⇒b2=254→()
Distance berween the foci =13
⇒2ae=13
Squaring both sides,
⇒4a2e2=132
⇒4a2(1+b2a2)=169
⇒a2+b2=1694
⇒a2+254=1694
⇒a2=1694−254=1444
⇒a2=36
Equation of a hyperbola is given by,
x2a2−y2b2=1
∴ Equation of the given hyperbola,
⇒x236−4y225=1
Related Playlists
Similar Questions
Find the equation of the hyperbola whose eccentricity is √2 and the distance between the foci is 16, taking transverse and conjugate axes of the hyperbola as xandy−axes respectively.
View SolutionFind the equation of the hyperbola in which the length of conjugate axis is 5 and the distance between foci is 13.
View SolutionFind the equation of hyperbola where, conjugate axis is 3 along Y-axis and distance between foci is 5.
View SolutionFind the equation of the hyperbola with eccentricity √2 and the distance between whose foci is 16.
View SolutionThe equation of the hyperbola whose eccentricity is √2 and the distance between the foci is 16, taking transverse and conjugate axes of the hyperbola as X and Y-axes respectively, is
View SolutionFind the equation of hyperbola whose conjugate axis = latus-rectum = 8 ,
View SolutionFind the equation of the hyperbola in standard form , if :
conjugata axis = 5 and distance between foci = 13View SolutionFind th equation of the hyperbola eccentricity is 2 and the distance between foci 16.
View Solution(i) Find the equation of hyperbola whose eccentricity is √1312 and the distance between foci is 26.
(ii) The foci of a hyperbola coincide of the ellipse 9x2+25y2=225. If the eccentricity of the hyperbola is 2, then find its equation.View SolutionThe equation to the hyperbola having its eccentricity 2 and the distance between its foci is 8 is
View Solution