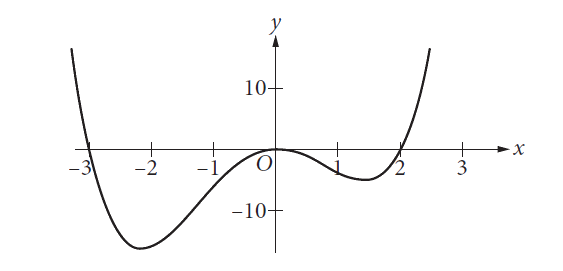
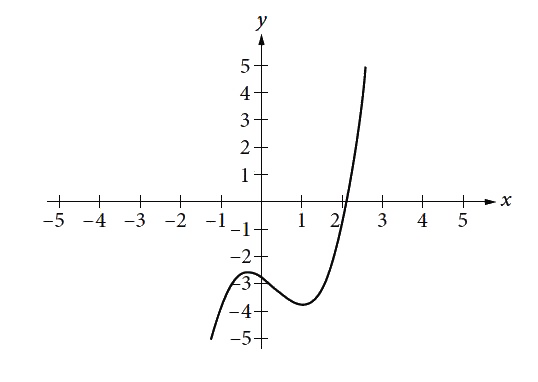If 8and−4 are the solutions for x, which of the following could be the equation?
x2−4x−32=0
x2−4x+32=0
x2+4x−12=0
x2+4x+32=0
The correct Answer is:A
To find the quadratic equation with the solutions 8 and −4, we can follow these steps:
Step 1: Write the factors based on the solutions
Since the solutions of the quadratic equation are x=8 and x=−4, we can express the factors of the equation as:
(x−8)and(x+4)
Step 2: Form the equation
To form the quadratic equation, we multiply the factors:
(x−8)(x+4)=0
Step 3: Expand the equation
Now, we will expand the product:
x2+4x−8x−32=0
Step 4: Combine like terms
Next, we combine the like terms:
x2+(4x−8x)−32=0
This simplifies to:
x2−4x−32=0
Conclusion
Thus, the quadratic equation that has 8 and −4 as solutions is:
x2−4x−32=0
Since the solutions of the quadratic equation are x=8 and x=−4, we can express the factors of the equation as:
(x−8)and(x+4)
To form the quadratic equation, we multiply the factors:
(x−8)(x+4)=0
Now, we will expand the product:
x2+4x−8x−32=0
Next, we combine the like terms:
x2+(4x−8x)−32=0
This simplifies to:
x2−4x−32=0
Thus, the quadratic equation that has 8 and −4 as solutions is:
x2−4x−32=0
Similar Questions
Knowledge Check
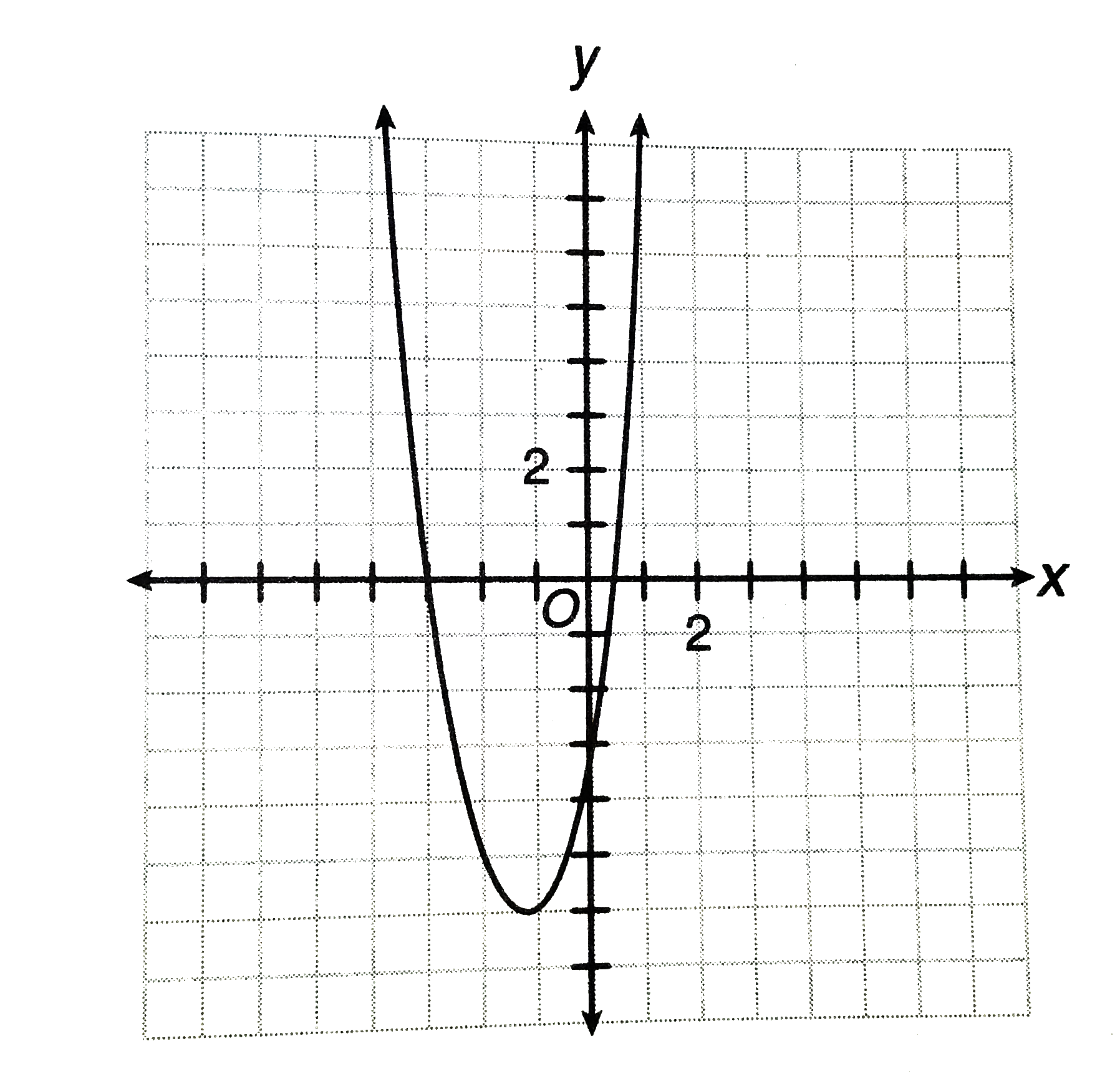
Which of the following could be the equation of the graph above?Ay=(x−3)(2x+1)By=(x+3)(2x−1)Cy=−(x−3)(1+2x)Dy=12(x+3)(x−1)2x2−4x=t
In the equation above, t is a constant. If the equation has no real solutions, which of the following could be the value of t ?A−3B−1C1D3
Two graphs f(x) and h(x) are shown above. IF f(x)=3x+4 and f(x) and h(x) are perpendicular, which of the following could be the equation of h(x)?Ah(x)=13x+9Bh(x)=−13x+9Ch(x)=3x+9Dh(x)=−3x+9
If one solution of x2−22x+d=0 is 6, which of the following could be the value of d ?
View Solution{(y=(1)/(2)x-2), (y=-x^(2)+1):}
If (a, b) is a solution to the system of equations above, which of the following could be the value of b?
View SolutionThree different chefs work together to prepare meals for 280 dinner guests. Each works at a different speed, and their combined output throughout the night is modeled by the equation 8x+4x+2x=280. If x is a positive integer, which of the following could 8x represent in the equation?
View Solution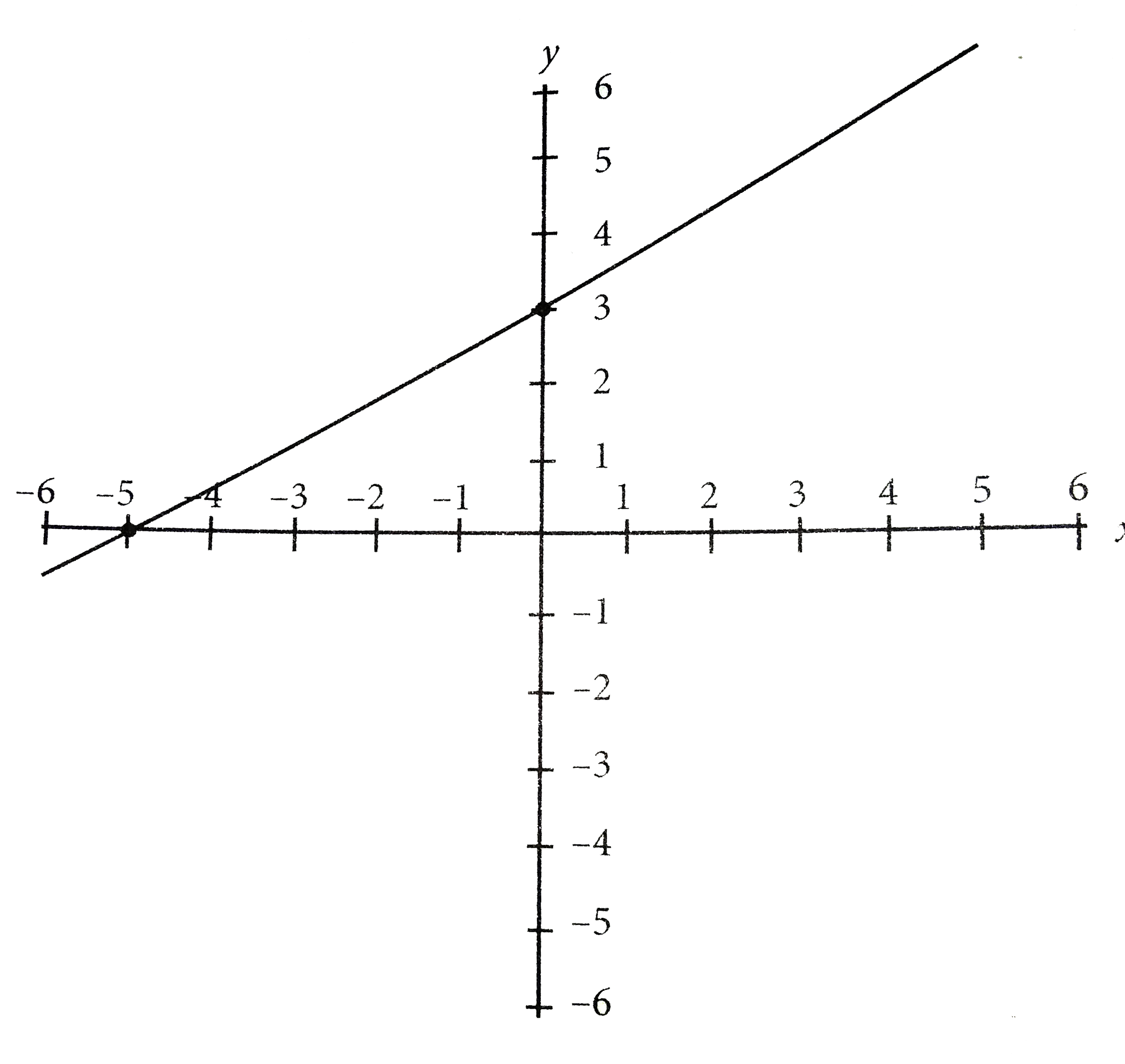
In the figure above, of y=f(x) is shown. Which of the following could be the equation of f(x)?
View SolutionThe center of circle O (not shown) falls on the point where the line y=43x+4 intersects the x-axis on the coordinate plane. The point (3, 8) lies on the circumference of the circle. Which of the following could be the equation for circle O?
View Solution