At a particular position the velocity of a particle in SHM with amplitude a is √3/2 that at its mean position. In this position, its displacement is :
a/2
√3a/2
a√2
√2a
The correct Answer is:A
To solve the problem, we need to find the displacement of a particle in Simple Harmonic Motion (SHM) when its velocity is given as √32 times the velocity at its mean position. Let's go through the solution step by step.
Step 1: Understand the equations of SHM
The general equation for displacement x in SHM is given by:
x=Asin(ωt)
where A is the amplitude and ω is the angular frequency.
Step 2: Find the expression for velocity
The velocity v in SHM can be derived by differentiating the displacement with respect to time:
v=dxdt=Aωcos(ωt)
Step 3: Determine the velocity at the mean position
At the mean position, the displacement x=0, which occurs when ωt=0. At this point, the velocity is maximized:
vmean=Aω
Step 4: Relate the given velocity to the mean position velocity
According to the problem, the velocity at a certain position is:
v=√32vmean=√32(Aω)
Step 5: Set up the equation
From the expression for velocity, we can set up the equation:
Aωcos(ωt)=√32(Aω)
Dividing both sides by Aω (assuming A≠0 and ω≠0):
cos(ωt)=√32
Step 6: Solve for ωt
The cosine function equals √32 at:
ωt=π6andωt=11π6(in the range (0,2π))
Step 7: Find the displacement at ωt=π6
Now we can find the displacement x at ωt=π6:
x=Asin(π6)
Since sin(π6)=12:
x=A⋅12=A2
Conclusion
Thus, the displacement of the particle at the given position is:
A2
The general equation for displacement x in SHM is given by:
x=Asin(ωt)
where A is the amplitude and ω is the angular frequency.
The velocity v in SHM can be derived by differentiating the displacement with respect to time:
v=dxdt=Aωcos(ωt)
At the mean position, the displacement x=0, which occurs when ωt=0. At this point, the velocity is maximized:
vmean=Aω
According to the problem, the velocity at a certain position is:
v=√32vmean=√32(Aω)
From the expression for velocity, we can set up the equation:
Aωcos(ωt)=√32(Aω)
Dividing both sides by Aω (assuming A≠0 and ω≠0):
cos(ωt)=√32
The cosine function equals √32 at:
ωt=π6andωt=11π6(in the range (0,2π))
Now we can find the displacement x at ωt=π6:
x=Asin(π6)
Since sin(π6)=12:
x=A⋅12=A2
Thus, the displacement of the particle at the given position is:
A2
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBook:MOTIONChapter:SIMPLE HARMONIC MOTIONExercise:EXERCISE -3 Section - A Previous Year Problems | NEET
Explore 24 Videos
- SIMPLE HARMONIC MOTIONBook:MOTIONChapter:SIMPLE HARMONIC MOTIONExercise:EXERCISE -3 Section - B Previous Year Problems | JEE MAIN
Explore 23 Videos
- SIMPLE HARMONIC MOTIONBook:MOTIONChapter:SIMPLE HARMONIC MOTIONExercise:EXERCISE -1 (Objective Problems | NEET) SECTION-E Simple Pendulum
Explore 6 Videos
- SEMI CONDUCTOR AND LOGIC GATESBook:MOTIONChapter:SEMI CONDUCTOR AND LOGIC GATESExercise:EXERCISE 3
Explore 34 Videos
- SIMPLE HARONIC MOTION Book:MOTIONChapter:SIMPLE HARONIC MOTION Exercise:EXERCISE -4 (Leve-II) [ JEE 2011]
Explore 11 Videos
Similar Questions
A particle executes simple harmonic motion with an amplitude of 4cm At the mean position the velocity of tge particle is 10 earth distance of the particle from the mean position when its speed 5 point is
View SolutionA particle performing SHM with a frequency of 5Hz and amplitude 2cm is initially at position exterme position. The equation for its displacement (in metre) is
View Solution
Knowledge Check
The velocity of a particle performing S.H.M. at mean position is
AmaximumBhalf of the maximumCminimumDzeroThe frequency of oscillation of a particle executing SHM with amplitude A and having velocity 'v' at the mean position is
Av2πABvACAvD2πAvThe velocity of a particle in simple harmonic motion at displacement y from mean position is
Aω√(a2+y2)Bω√(a2−y2)CwyDω2√(a2+y2)
A particle executes SHM of amplitude A. At what distance from the mean position is its KE equal to its PE ?
View SolutionIf the maximum velocity of a particle in SHM is v0 then its velocity at half the amplitude from position of rest will be :
View SolutionWhen a particle executing a linear S.H.M. moves from its extreme position to the mean position, its
View SolutionTwo particle undergoes SHM along parallel line with the same time period (T) and equal amplitude At a particular instant , one particle is at its extereme position while the other is at its mean position .They move in the same direction .They will cross each other after a further time
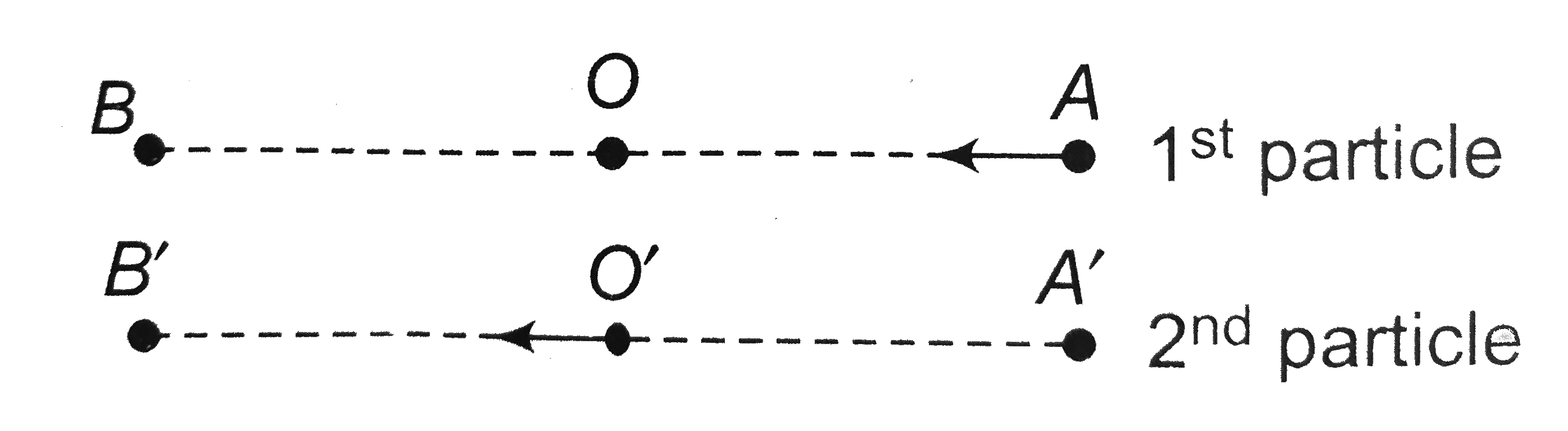
View SolutionThe velocity of particle undergoing SHM is v at the mean position. If only amplitude is doubled, the velocity at mean position
View Solution