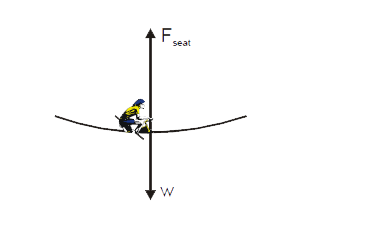
An aeroplane moves at 64 m/s in a vertical loop of radius 120 m, as shown in figure. Calculate the force of the plane’s seat on 72 kg pilot while passing through the bottom part of the loop.

Two forces acts on the pilot his downward weigth force w and the upward force of the aeroplane’s seat . Because the pilot moves in a circular path, these forces along the radial direction must, according to Newton’s seconds law ( F = ma), equal the pilot’s mass times his centripetal acceleration, where /r. We find that F
(in radial direction) =
Remember that force pointing towards the center of the circle () are positive & those pointing away from the center (w) are negative. Substituting = mg and rearranging, we find that the force of the aeroplane seat on the pilot is
= 72kg (34.1 m/ + 9.8 m/ ) = 3160.8 N
The pilot in this example feels very heavy. To keep him in the circular path, the seat must push the pilot upwards with a force of 3160 N, 4.5 times his normal weight. He experiences an acceleration of 4.5 g, that is, 4.5 times the acceleration of gravity.
Topper's Solved these Questions
- CIRCULAR MOTIONBook:MOTIONChapter:CIRCULAR MOTIONExercise:EXERCISE -1
Explore 41 Videos
- CIRCULAR MOTIONBook:MOTIONChapter:CIRCULAR MOTIONExercise:EXERCISE - 2
Explore 16 Videos
- CENTRE OF MASSBook:MOTIONChapter:CENTRE OF MASSExercise:Exercise - 4 Level-II
Explore 20 Videos
- COMMUNICATION SYSTEMBook:MOTIONChapter:COMMUNICATION SYSTEMExercise:EXERCISE - 3
Explore 6 Videos
Similar Questions
An aeroplane flying in the sky dives with a speed of 360km/h in a verticale circle of radius 200 m The weight of pilot sitting in it is 75 kg Calulate the force with which the pilot presses his seat when the aeroplane is (i) at the lowest position and (ii) at the highest position Take g=10m/s−2 .
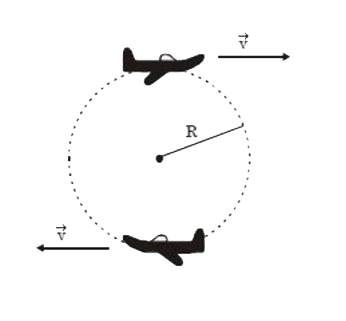
View SolutionA jet travelling at a constant speed of 1.20×102 m/s executes a vertical loop with a radius of 5.00×105 m.(See fig.) Find the magnitude of the force (in N) of the seat on a 70.0 kg pilot at the top of the loop. (Take: g=10m/s2)
View Solution
Knowledge Check
The moment of Inertia of an incomplete disc of mas M and radius R as shown in figure about the axis passing through the centre and perpendicular to the plane of the disc is:
 AMR22B7MR212C7MR26D5MR212
AMR22B7MR212C7MR26D5MR212An aeroplane flying in the sky with a uniform speed of 200m/s moves in a vertical circle of radius 400 m. The mass of the pilot is 70 kg. The force exerted by the pilot on the seat at the highest point of the circle will be [Take g=10m/s2]
A3000 NB6300 NC7700 ND630 NA current of 10A is passing through a long wire which has semicircular loop of the radius 20cm as shown in the figure. Magnetic field produced at the centre of the loop is
 A10πμTB5πμTC4πμTD2πμT
A10πμTB5πμTC4πμTD2πμT
An aeroplane flying in the sky dives with a speed of 360km/h in a verticale circle of radius 200 m The weight of pilot sitting in it is 75 kg Calulate the force with which the pilot presses his seat when the aeroplane is (i) at the lowest position and (ii) at the highest position Take g=10m/s−2 .
View SolutionA conducting square loop of side 10 cm is placed in a region of uniform magnetic field 0.5 T as shown in the figure. Calculate the work done in slowly moving the loop out of the field in 2 seconds with a speed of 0.05ms−1. The resistance of loop is 10 Ω.

View SolutionA particle of mass 100 g is moving in a vertical circle of radius 2 m The particle is just looping the loop . What is the speed of the particle and tension in the string at the highest point of the circular path ? . (g=10ms−2) .
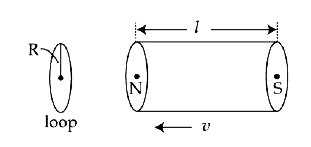
View SolutionA bar magnet is passing through a conducting loop of radius R with velocity v. The radius of the bar magnet is such that it just passes through the loop. The induced e.m.f. in the loop can be represented by the approximate curve :
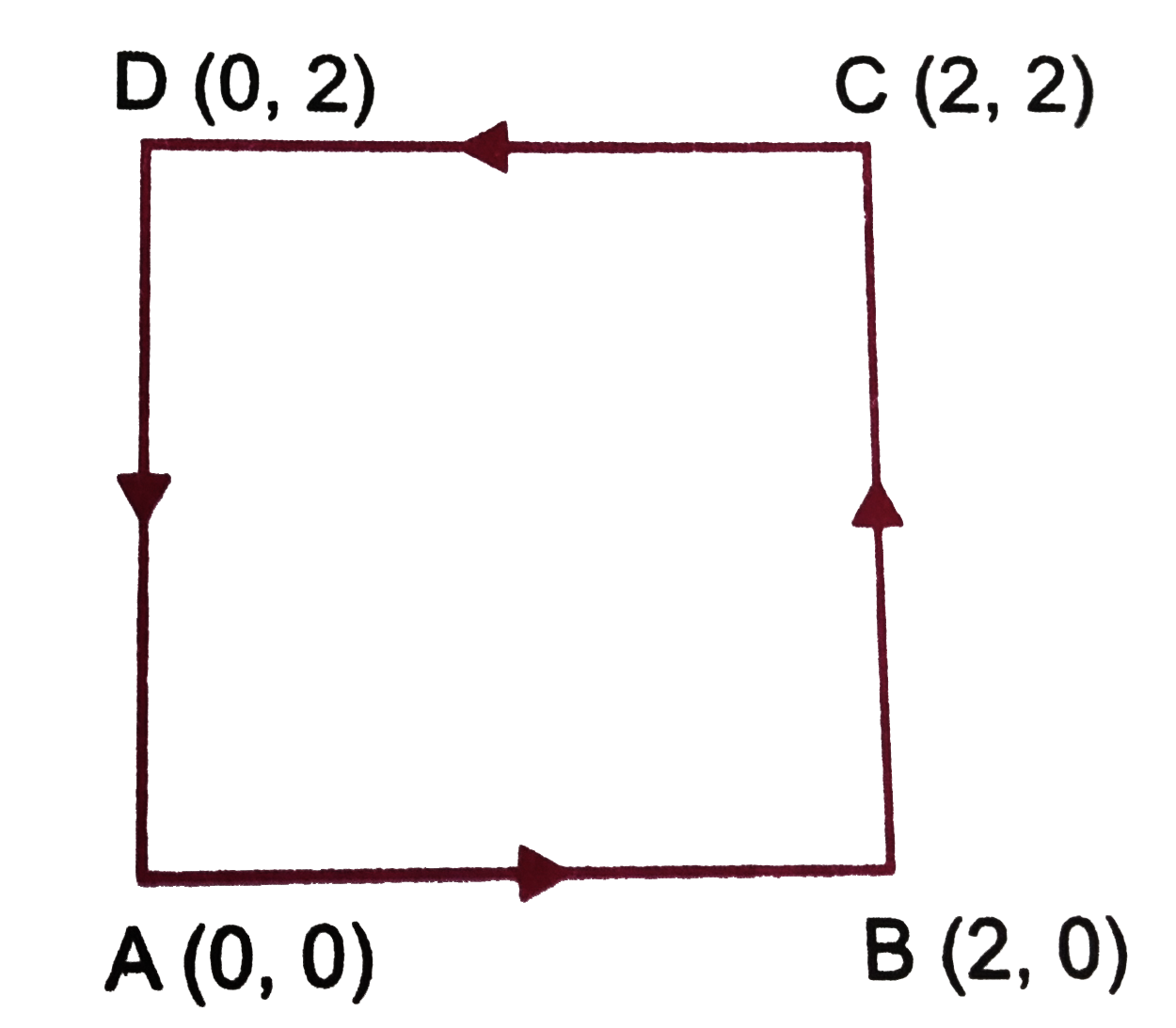
View SolutionA force →F=2xˆj newton acts in a region where a particle moves annticlockwise in a square loop of 2m in x−y plane as shown in figure . Calculate the total amount of work done. Is this force a conservative force or a non-conservative force?
View Solution