The velocity at which the mass of a particle becomes twice of its rest mass, will be -
2c3
c2
c√32
3c4
The correct Answer is:C
To find the velocity at which the mass of a particle becomes twice its rest mass, we can use the concept of relativistic mass. Here’s a step-by-step solution:
Step 1: Understand the relationship between moving mass and rest mass
The moving mass m of a particle is related to its rest mass m0 by the equation:
m=m0√1−v2c2
where:
- m is the moving mass,
- m0 is the rest mass,
- v is the velocity of the particle,
- c is the speed of light in vacuum.
Step 2: Set up the equation for the given condition
According to the problem, we want to find the velocity v at which the moving mass m becomes twice the rest mass m0:
m=2m0
Substituting this into the equation gives:
2m0=m0√1−v2c2
Step 3: Simplify the equation
We can cancel m0 from both sides (assuming m0≠0):
2=1√1−v2c2
Step 4: Square both sides
Squaring both sides of the equation results in:
4=11−v2c2
Step 5: Rearrange the equation
Rearranging gives:
1−v2c2=14
This simplifies to:
v2c2=1−14=34
Step 6: Solve for v
Taking the square root of both sides:
vc=√34=√32
Thus, we can express v as:
v=c⋅√32
Final Answer
The velocity at which the mass of a particle becomes twice its rest mass is:
v=√32c
---
The moving mass m of a particle is related to its rest mass m0 by the equation:
m=m0√1−v2c2
where:
- m is the moving mass,
- m0 is the rest mass,
- v is the velocity of the particle,
- c is the speed of light in vacuum.
According to the problem, we want to find the velocity v at which the moving mass m becomes twice the rest mass m0:
m=2m0
Substituting this into the equation gives:
2m0=m0√1−v2c2
We can cancel m0 from both sides (assuming m0≠0):
2=1√1−v2c2
Squaring both sides of the equation results in:
4=11−v2c2
Rearranging gives:
1−v2c2=14
This simplifies to:
v2c2=1−14=34
Taking the square root of both sides:
vc=√34=√32
Thus, we can express v as:
v=c⋅√32
The velocity at which the mass of a particle becomes twice its rest mass is:
v=√32c
Topper's Solved these Questions
- MATTER WAVEBook:MOTIONChapter:MATTER WAVEExercise:EXERCISE 3 (SECTION A)
Explore 17 Videos
- MATTER WAVEBook:MOTIONChapter:MATTER WAVEExercise:EXERCISE 3 (SECTION B)
Explore 7 Videos
- MATTER WAVEBook:MOTIONChapter:MATTER WAVEExercise:EXERCISE 1 (SECTION B : DAVISSION GERMER EXPERIMENT )
Explore 11 Videos
- MAGNETISM -1Book:MOTIONChapter:MAGNETISM -1Exercise:Exercise - 3 SECTION -A
Explore 56 Videos
- MODERN PHYSICSBook:MOTIONChapter:MODERN PHYSICSExercise:PHYSICS
Explore 73 Videos
Similar Questions
Knowledge Check
The velocity at which the mass of a particle becomes twice its rest mass, will be -
A2c3Bc2Cc√32D3c4As the speed of a particle increases, its rest mass
AincreasesBdecreasesCremains the sameDchangesWhen the mass of an electron becomes equal to thrice its rest mass, its speed is
A2√23cB23cC13cD14c
At what speed must a particle move for its rest mass to be trebled?
View SolutionIf the material particle is moving with velocity v and the velocity of light is c, then mass of particle is taken as (m0 is rest mass)
View SolutionEscape velocity of a body from the surface of earth is 11.2km/sec. from the earth surface. If the mass of earth becomes double of its present mass and radius becomes half of its present radius then escape velocity will become
View SolutionThe velocity of a particle at which the kinetic energy is eqyal to its rest mass energy is
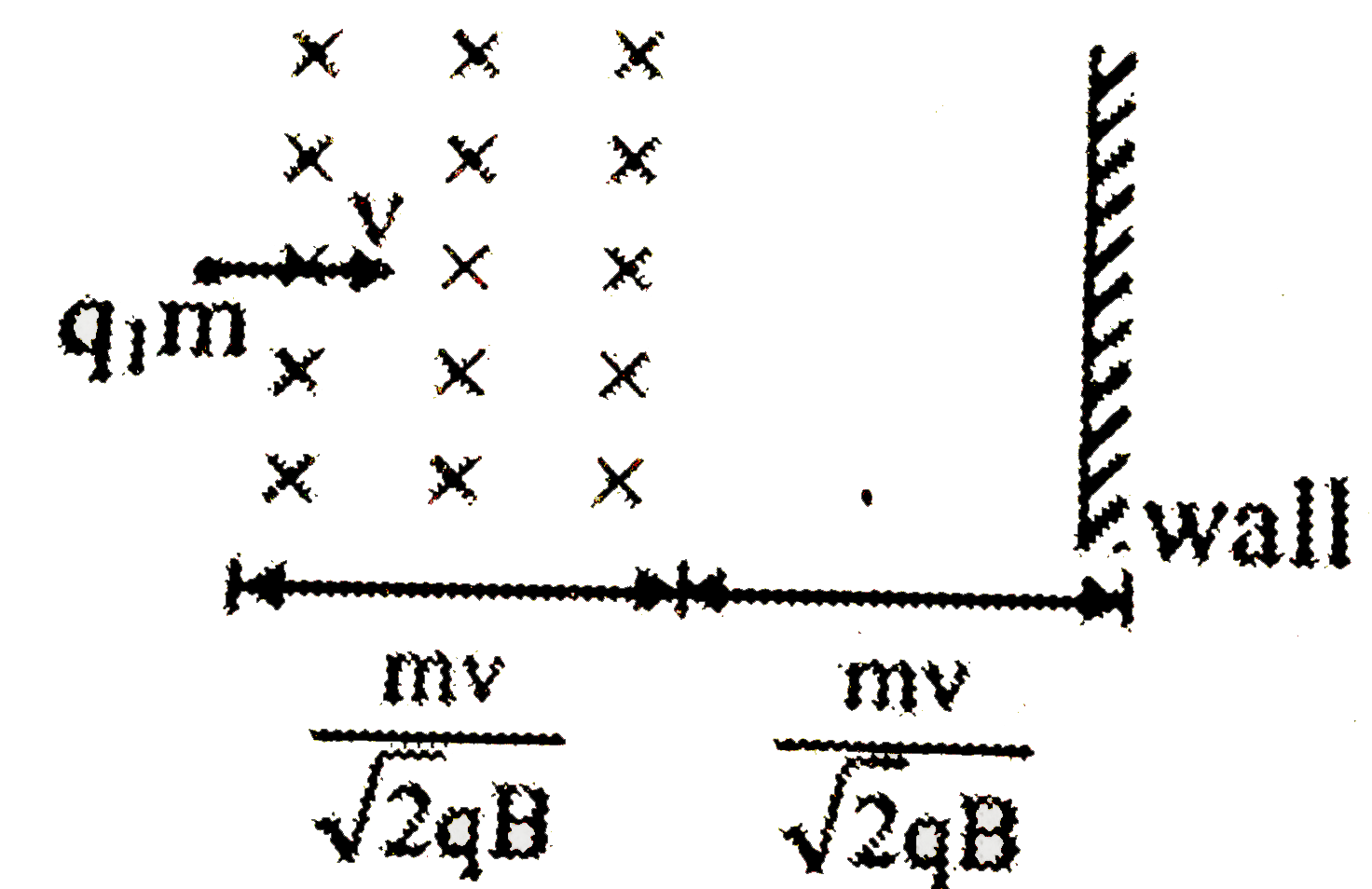
View SolutionA particle of mass m and charge q enters a region of magnetic field (as shown) with speed v at t = 0. There is a region in which the magnetic field is absent as shown. The particle after entering the region collide elastically with a rigid wall. Time t after which the velocity of particle become antiparallel to its initial velocity is -
View Solution