A cylinder is filled with non viscous liquid of density d to a hegiht h0 and a hole is made at a height h1 from the bottom of the cylinder. The velocity of liquid issuing out of the hole is
√(2gh0)
√2g(h0−h1)
√dgh1
√dgh0
The correct Answer is:B
To find the velocity of the liquid issuing out of the hole in the cylinder, we can use Bernoulli's equation. Here’s a step-by-step solution:
Step 1: Understand the Setup
We have a cylinder filled with a non-viscous liquid of density d to a height h0. A hole is made at a height h1 from the bottom of the cylinder. We need to determine the velocity of the liquid as it exits through the hole.
Step 2: Identify Points for Bernoulli's Equation
We will apply Bernoulli's equation between two points:
- Point 1: At the free surface of the liquid (height h0)
- Point 2: At the hole (height h1)
Step 3: Write Bernoulli's Equation
Bernoulli's equation states that:
P1+12ρV21+ρgh1=P2+12ρV22+ρgh2
Where:
- P1 and P2 are the pressures at points 1 and 2 respectively.
- V1 and V2 are the velocities at points 1 and 2 respectively.
- h1 and h2 are the heights at points 1 and 2 respectively.
Step 4: Assign Values
- At point 1 (free surface), the pressure P1 is atmospheric pressure P0, and since the liquid is at rest, V1≈0.
- At point 2 (the hole), the pressure P2 is also atmospheric pressure P0.
Thus, we can simplify the equation:
P0+0+ρgh0=P0+12ρV22+ρgh1
Step 5: Cancel Out Terms
Since P0 appears on both sides, we can cancel it out:
ρgh0=12ρV22+ρgh1
Step 6: Rearrange the Equation
Rearranging gives:
ρgh0−ρgh1=12ρV22
Factoring out ρg:
ρg(h0−h1)=12ρV22
Step 7: Cancel ρ
We can cancel ρ from both sides (since the liquid is non-viscous and incompressible):
g(h0−h1)=12V22
Step 8: Solve for V2
Multiplying both sides by 2:
2g(h0−h1)=V22
Taking the square root:
V2=√2g(h0−h1)
Final Answer
The velocity of the liquid issuing out of the hole is:
V=√2g(h0−h1)
---
We have a cylinder filled with a non-viscous liquid of density d to a height h0. A hole is made at a height h1 from the bottom of the cylinder. We need to determine the velocity of the liquid as it exits through the hole.
We will apply Bernoulli's equation between two points:
- Point 1: At the free surface of the liquid (height h0)
- Point 2: At the hole (height h1)
Bernoulli's equation states that:
P1+12ρV21+ρgh1=P2+12ρV22+ρgh2
Where:
- P1 and P2 are the pressures at points 1 and 2 respectively.
- V1 and V2 are the velocities at points 1 and 2 respectively.
- h1 and h2 are the heights at points 1 and 2 respectively.
- At point 1 (free surface), the pressure P1 is atmospheric pressure P0, and since the liquid is at rest, V1≈0.
- At point 2 (the hole), the pressure P2 is also atmospheric pressure P0.
P0+0+ρgh0=P0+12ρV22+ρgh1
Since P0 appears on both sides, we can cancel it out:
ρgh0=12ρV22+ρgh1
Rearranging gives:
ρgh0−ρgh1=12ρV22
Factoring out ρg:
ρg(h0−h1)=12ρV22
We can cancel ρ from both sides (since the liquid is non-viscous and incompressible):
g(h0−h1)=12V22
Multiplying both sides by 2:
2g(h0−h1)=V22
Taking the square root:
V2=√2g(h0−h1)
The velocity of the liquid issuing out of the hole is:
V=√2g(h0−h1)
Topper's Solved these Questions
- HYDROSTATIC, FLUID MECHANICS & VISCOSITYBOOK - MOTIONCHAPTER - HYDROSTATIC, FLUID MECHANICS & VISCOSITYEXERCISE - EXERCISE -3 (SECTION-B) PREVIOUS YEAR PROBLEM
7 Videos
- HYDROSTATIC, FLUID MECHANICS & VISCOSITYBOOK - MOTIONCHAPTER - HYDROSTATIC, FLUID MECHANICS & VISCOSITYEXERCISE - EXERCISE-2 (ASSERTION AND REASON)
28 Videos
- HEAT-2 BOOK - MOTIONCHAPTER - HEAT-2 EXERCISE - EXERCISE-4 (LEVEL-II)
30 Videos
- KINEMATICSBOOK - MOTIONCHAPTER - KINEMATICSEXERCISE - EXERCISE 4 PREVIOUS YEAR (LEVEL 2)
6 Videos
Similar Questions
A large vessel of height H is filled with a liquid of density ρ upto the brim. A small hole of radius r is made at the side vertical face, close to the base. The horizontal force is required to stop the gushing of liquid is
View SolutionA liquid of density ρ is filled in a vessel up to height H and a hole of cross section area A is made at a depth h below the free surface of liquid. The speed of liquid coming out of the hole is independent of
View Solution
Knowledge Check
In a container, filled with water upto a height h, a hole is made in the bottom. The velocity of water flowing out of the hole is
Aproportional to hBproportional to h1/2Cproportional to h2Dindependent of hA wide vessel with a small hole at the bottom is filled with two liquids. The density and height of one liquid are ρ1 and h1 and that of the other are ρ2 and h2(ρ1>ρ2). The velocity of liquid coming out of the hole is:
Av=√2g(h1+h2)Bv=√2(h1ρ1+h2ρ2)/(ρ1+ρ2)Cv=√2g(h1+h2ρ2ρ1)Dv=√2g(h1ρ1ρ2+h2)A cylinder is fill ed with a liquid of density p upto a height h. What is the hydrostatic pressure exerted by the liquid on the bottom of the cylinder, if the cylinder is kept in a lifi moving upwa rds with an acceleration a ?
AρghBρ(g+a)Cρ(g−a)Dρgha
A hole is made in the bottom of a container having water filled up to a height h. The velocity of water flowing out of the hole is :
View SolutionA cylindrical vessel of radius r is filled with a homogeneous liquid upto a height h. It is found that the force exerted by the liquid column on the bottom of the cylinder is equal to the force exerted by the liquid on the sides of the cylinder. What is the relation between r and h?
View SolutionA cylindrical vessel is filled with a liquid up to a height H. A small hole is made in the vessel at a distance y below the liquid surface as shown in figure. The liquid emerging from the hole strike the ground at distance x
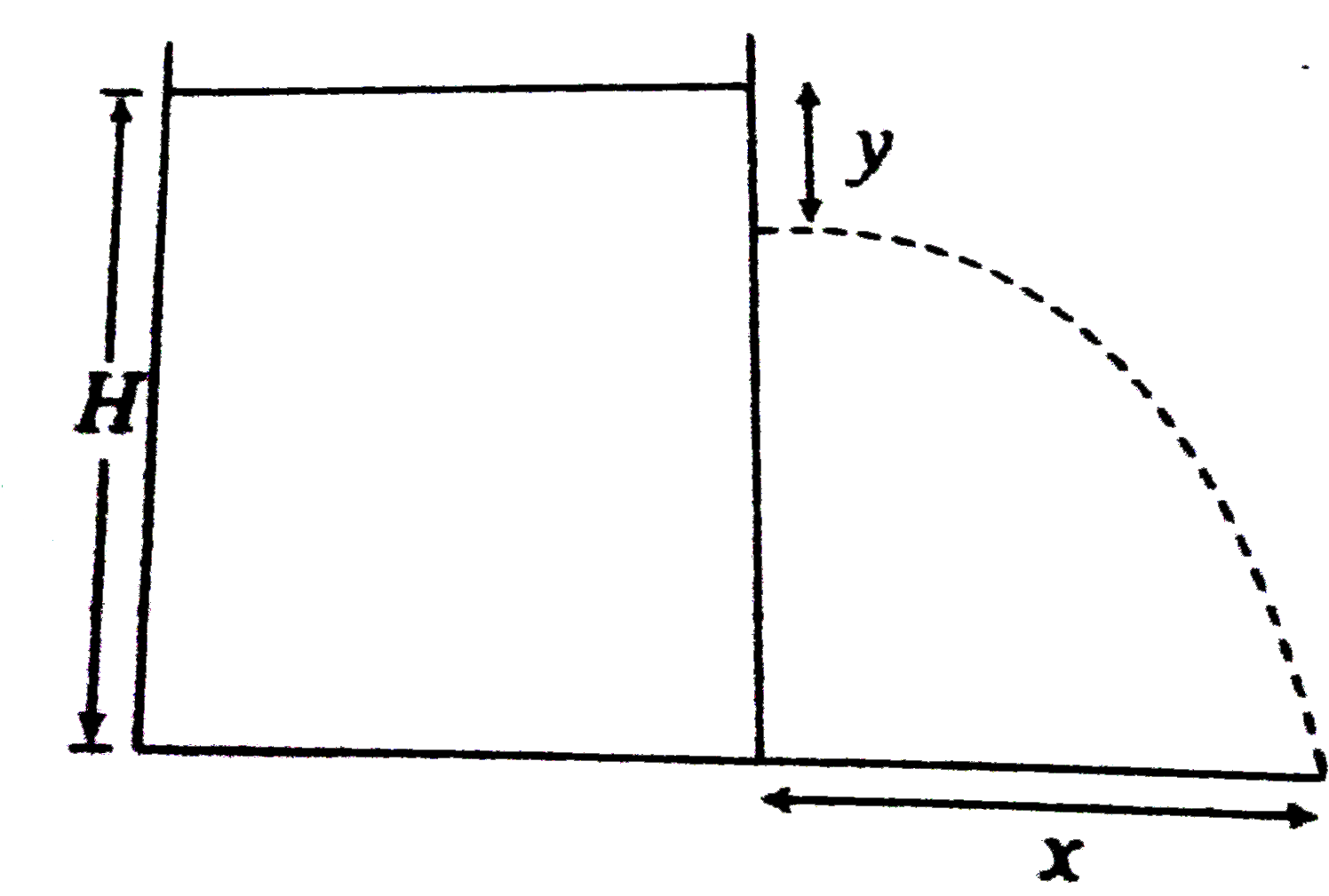
View SolutionWater is filled to height h in a fixed vertical cylinder placed on horizontal surface. At time t=0 a small hole a drilled at a height h/4 from bottom of cylinder as shown. The cross section area of hole is a and the cross-section area of cylinder is A such that A>>a.

The duration of time for which water flows out of hole is.
View SolutionWater is filled to height h in a fixed vertical cylinder placed on horizontal surface. At time t=0 a small hole a drilled at a height h/4 from bottom of cylinder as shown. The cross section area of hole is a and the cross-section area of cylinder is A such that A>>a.
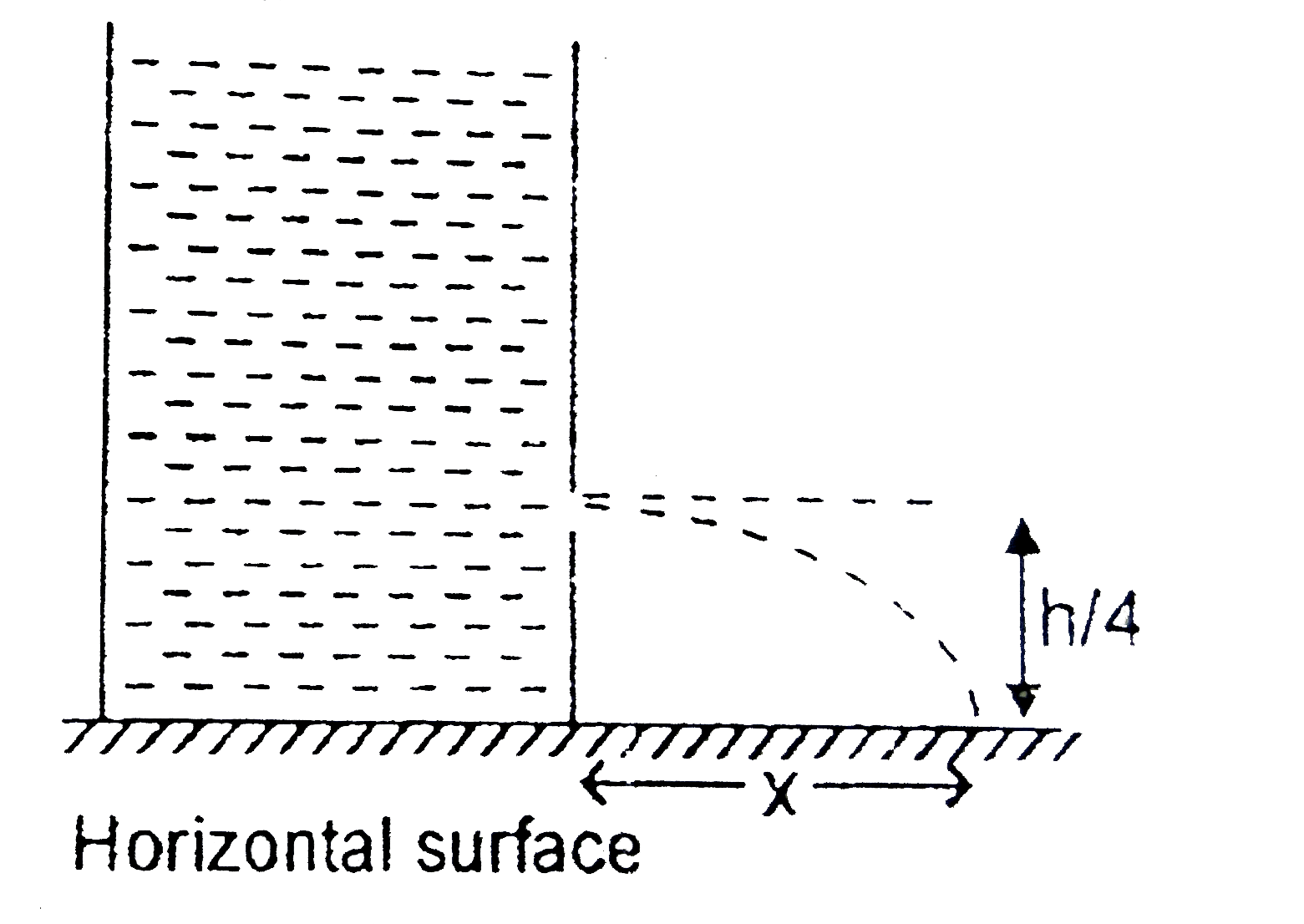
Let the value of horizontal distance of point where the4 water fall on horizontal surface from bottom of cylinder be x as shown. Then from time t=0 till water comes out of hole, pick the correct statement:
View Solution
