Find out the minimum force that must be applied on the block vertically downwards so that the block doesn’t move.
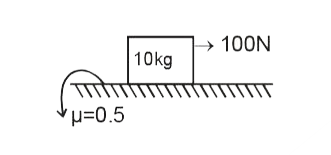
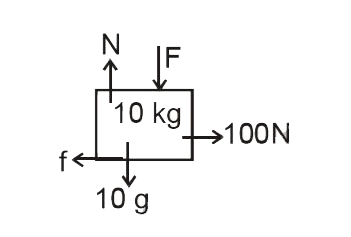
100−fs=0
∴fs=100……….(1)
F+10g=N⇒N=100+F…………(2)
Now 0≤fs≤μN
100≤0.5N
100≤0.5[100+F]
200≤100+F
F≤100N
∴ Minimum F = 100 N
Topper's Solved these Questions
- NLM & FRICTIONBOOK - MOTIONCHAPTER - NLM & FRICTIONEXERCISE - EXAMPLE
47 Videos
- NLM & FRICTIONBOOK - MOTIONCHAPTER - NLM & FRICTIONEXERCISE - SOLVED EXAMPLE
16 Videos
- NEWTON'S LAWS OF MOTION & FRICTION BOOK - MOTIONCHAPTER - NEWTON'S LAWS OF MOTION & FRICTION EXERCISE - Exercise - 3 Section-B
12 Videos
- ONE DIMENSION MOTIONBOOK - MOTIONCHAPTER - ONE DIMENSION MOTIONEXERCISE - Exercise - 3 |Section - B Previous Year Problems | JEE MAIN
12 Videos
Similar Questions
Find the minimum force F0 that should be applied to the block of mass m = 0.5 kg so that the block stays in equilibrium with the rough vertical wall having friction coefficient μ=0.2 (Take g=10ms2)
View SolutionBlock B of mass m has been placed on block A of mass 3 m as shown. Block A rests on a smooth horizontal table. F1 is the maximum horizontal force that can be applied on the block A such that there is no slipping between the blocks. Similarly, F2 is the maximum horizontal force that can be applied on the block B so that the two blocks move together without slipping on each other. When F1andF2 both are applied together as shown in figure.
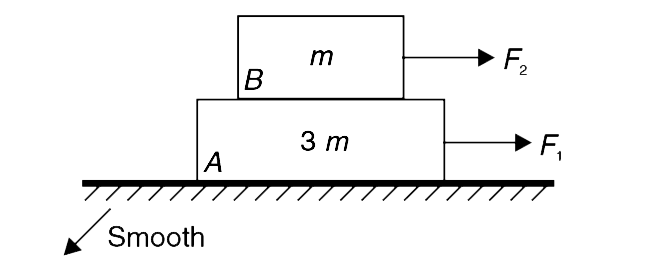
(a) Find the friction force acting between the blocks.
(b) Acceleration of the two blocks.
(c) If F2 is decreased a little, what will be direction of friction acting on B.
View Solution
Knowledge Check
In the figure shown, the minimum force F to be applied perpendicular to the incline so that the block does not slide is
 A200NB40NC120NDnone
A200NB40NC120NDnoneA 4.0 kg block is put on top of a 5.0kg block. In order to cause the top block to slip on the bottom one, a horizontal force of 12N must be applied to the top block. Assuming a friction less table the maximum horizontal force F which can be applied to the lower block so that the blocks, will move together should be
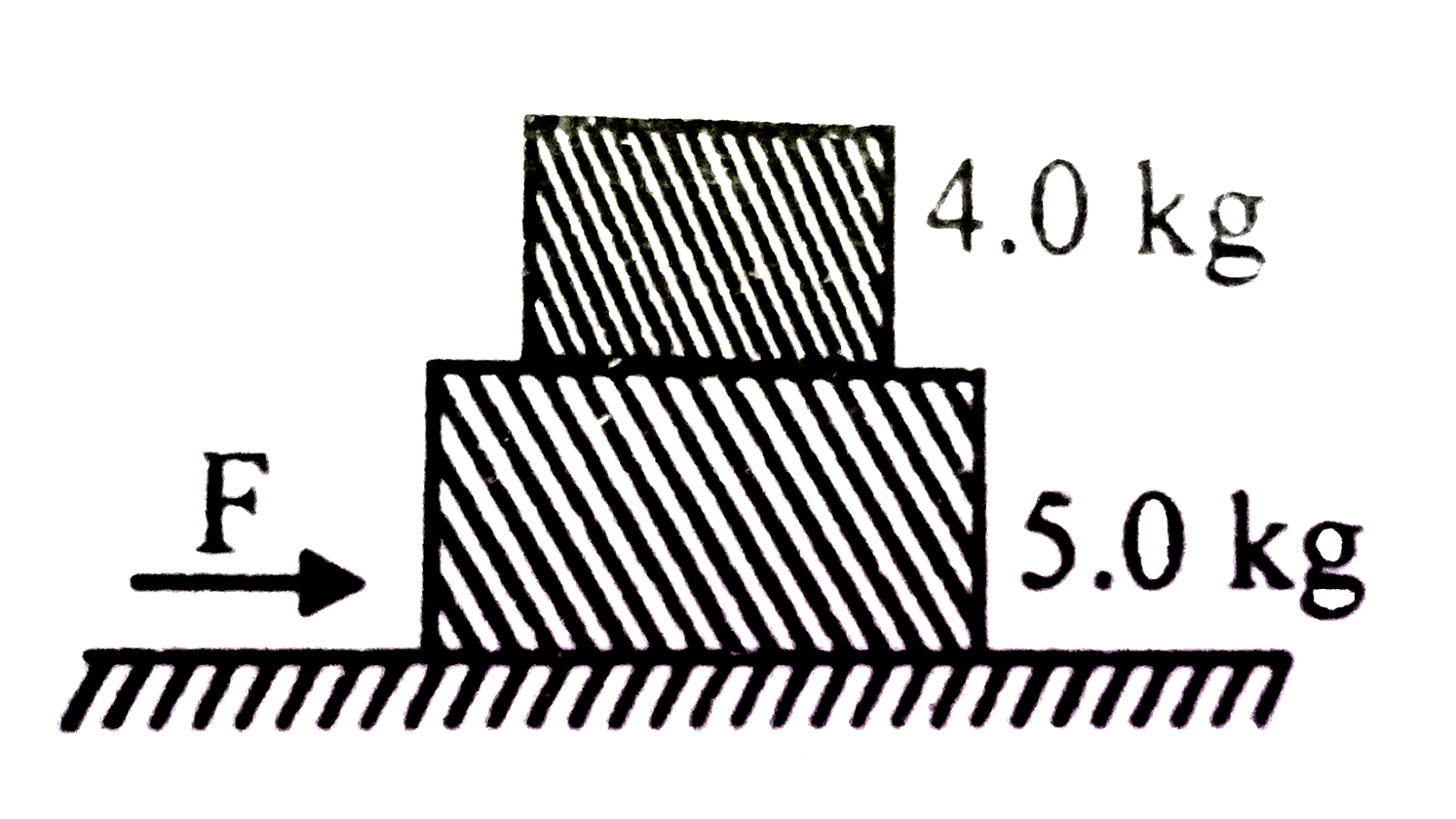 A<30NB<27NC<15ND<9N
A<30NB<27NC<15ND<9NThe minimum acceleration that must be impprted to the cart in the figure so that the block A will not fall (given μ is the coefficient if friction between the surface of block and cart) is given by:
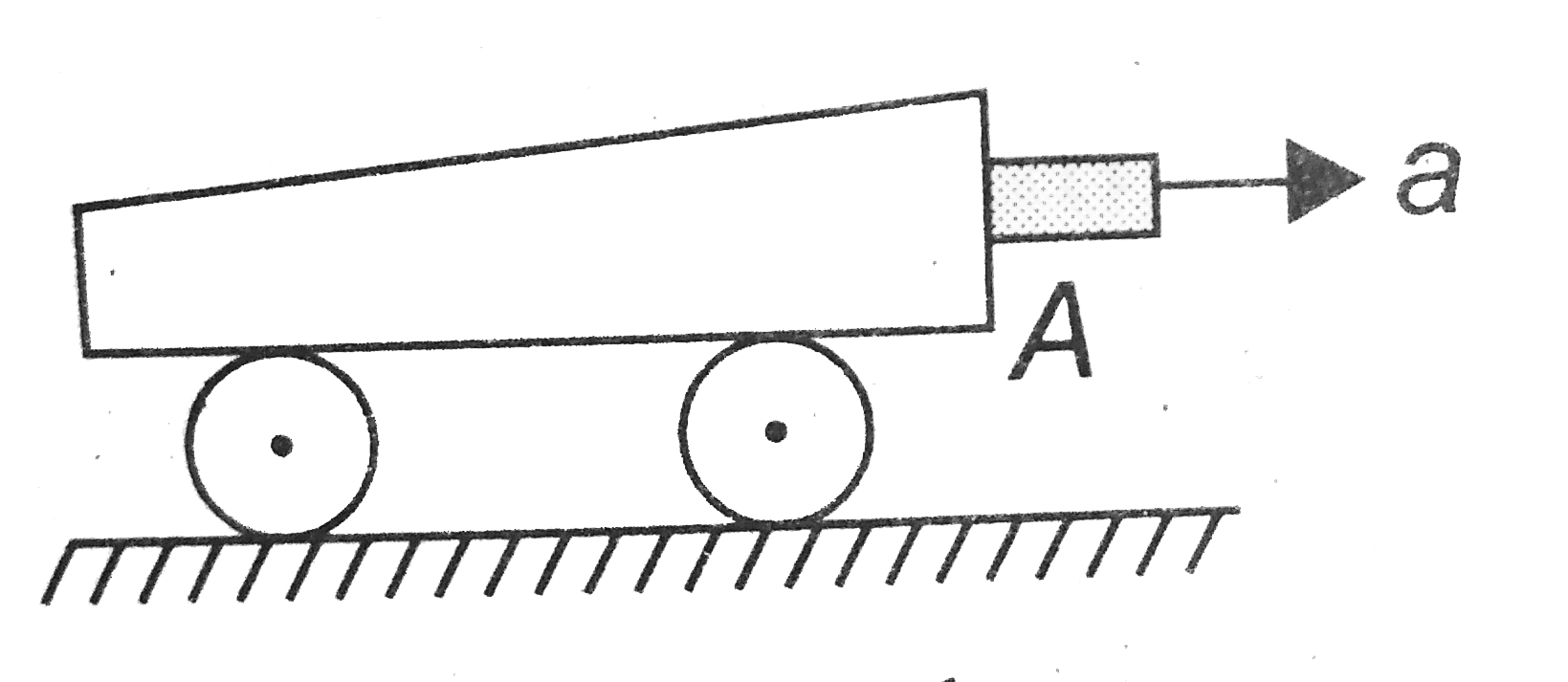 AμgBg/μCg√μDμg
AμgBg/μCg√μDμg
In the figure shown, the minimum force F to be applied perpendicular to the incline so that the block does not slide is

View SolutionWhat should be the minimum force P to be applied to the string so that block of mass m just begins to move up the frictionless plane?

View SolutionIn the above problem what is the minimum force by which 75 N force should be replaced with so that the block does not move.
View SolutionThe coefficient of static friction between the two blocks shown in figure is μ and the table is smooth. What maximum horizontal forced F can be applied to he block of mass M so that the block move together?

View SolutionFind the minimum value of force (in N) man should apply so that block can move
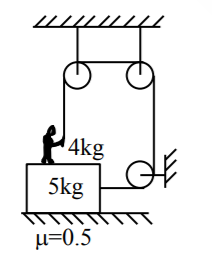
View Solution
