The circle $C_{1} : x^{2} + y 6 (2) = 3$ , with cenre at O, intersects the parabola $x^{2} = 2 y$ at the point P in the first quadrant. Let the tangent to the circle $C_{1}$ at P touches other two circles $C_{2}$ and $C_{3}$ at $R_{2}$ and $R_{3}$ respectively. Suppose $C_{2}$ and $C_{3}$ have equal radii $2 \sqrt{3}$ and centres $Q_{2}$ and $Q_{3}$ respectively. If $Q_{2}$ and $Q_{3}$ lie on the y-axis, then $Q_{2} Q_{3} =$

$Q_{2} Q_{3} = 12$

$R_{2} R_{3} = 4 \sqrt{6}$

area of the triangle $O R_{2} R_{3}$ is $6 \sqrt{2}$

area of the triangle $P Q_{2} Q_{3}$ is $4 \sqrt{2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, B, C

Updated on:21/07/2023

Answer

Step by step video, text & image solution for The circle C_(1) : x^(2)+y6(2)=3, with cenre at O, intersects the parabola x^(2)=2y at the point P in the first quadrant. Let the tangent to the circle C_(1) at P touches other two circles C_(2) and C_(3) at R_(2) and R_(3) respectively. Suppose C_(2) and C_(3) have equal radii 2sqrt(3) and centres Q_(2) and Q_(3) respectively. If Q_(2) and Q_(3) lie on the y-axis, then Q_(2)Q_(3)= by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS PARABOLA

Topper's Solved these Questions

Knowledge Check

The circle $C_{1} : x^{2} + y^{2} = 3$ , with centre at O, intersects the parabola $x^{2} = 2 y$ at the point P in the quadrant. Let the tangent to the circle $C_{1}$ at P touches other tqo circles $C_{2} and C_{3}$ at $R_{2} and R_{3}$ , respectively. Suppose $C_{2} and C_{3}$ have equal radii $2 \sqrt{3}$ and centres $Q_{2} and Q_{3}$ . respectively. If $Q_{2} and Q_{3}$ lie on the y-axis, then
A $Q_{2} Q_{3} = 12$
B $R_{2} R_{3} = 4 \sqrt{6}$
Carea of the triangle $O R_{2} R_{3} is 6 \sqrt{2}$
Darea of the triangle $P Q_{2} Q_{3} is 4 \sqrt{2}$
In, the charges on $C_{1}, C_{2}$ , and $C_{3}$ , are $Q_{1}, Q_{2}$ , and $Q_{3}$ , respectively.
.
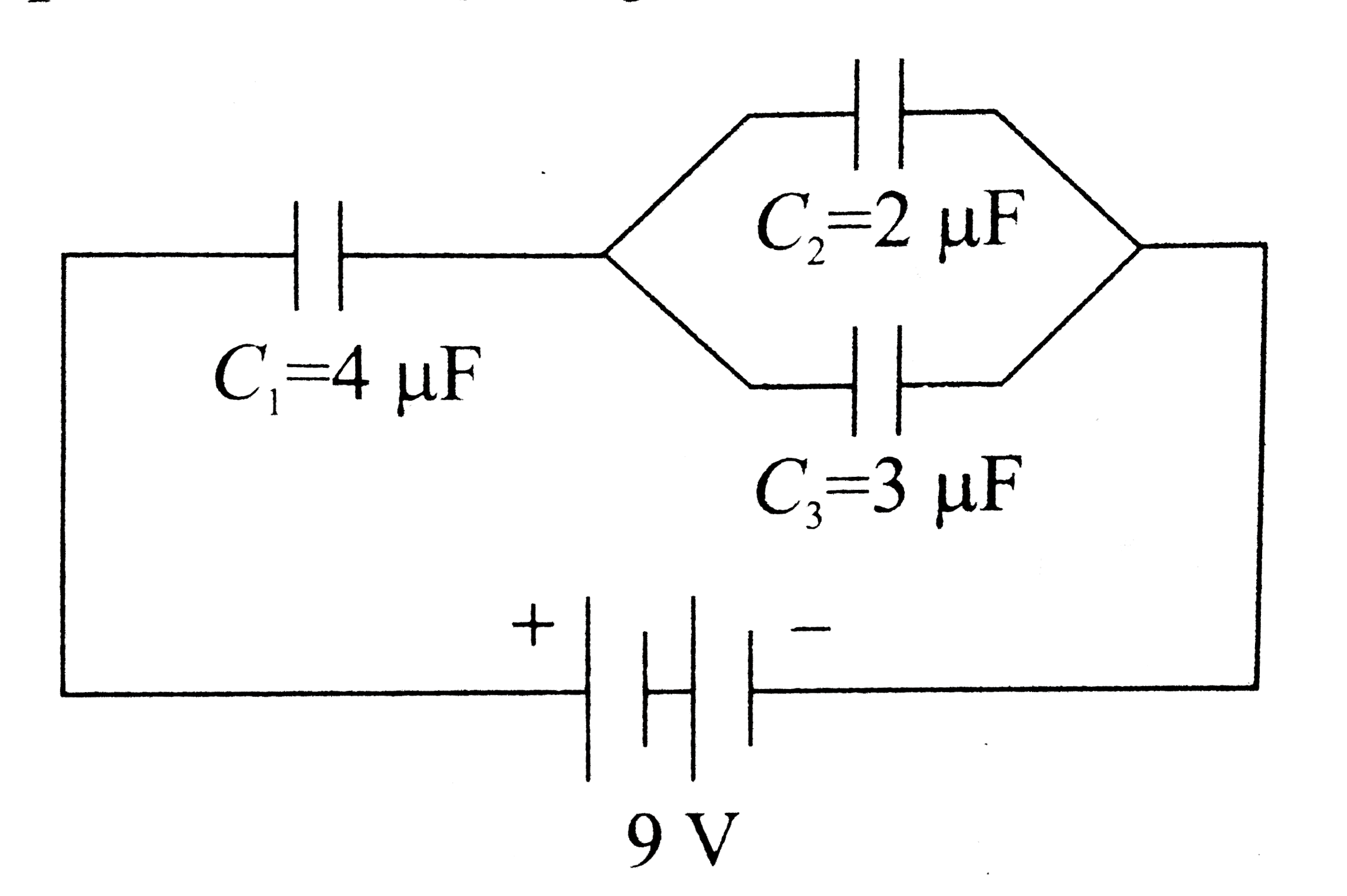A $Q_{2} = 8 μ C$
B $Q_{3} = 12 μ C$
C $Q_{1} = 20 μ C$
D $Q_{2} = 12 μ C$
Let $C_{1}, C_{2}$ be two circles touching each other externally at the point A and let AB be the diameter of circle $C_{1}$ . Draw a secant $B A_{3}$ to circle $C_{2}$ , intersecting circle $C_{1}$ at a point $A_{1} (\neq A),$ and circle $C_{2}$ at points $A_{2}$ and $A_{3}$ . If $B A_{1} = 2$ , $B A_{2} = 3$ and $B A_{3} = 4$ , then the radii of circles $C_{1}$ and $C_{2}$ are respectively
A $\frac{\sqrt{30}}{5}, \frac{3 \sqrt{30}}{10}$
B $\frac{\sqrt{5}}{2}, \frac{7 \sqrt{5}}{10}$
C $\frac{\sqrt{6}}{2}, \frac{\sqrt{6}}{2}$
D $\frac{\sqrt{10}}{3}, \frac{17 \sqrt{10}}{30}$

MOTION-PARABOLA-EXERCISE - IV

Home
Ask Doubt
Profile

The correct Answer is:A, B, C

Topper's Solved these Questions

Similar Questions

Knowledge Check

In, the charges on C1,C2, and C3, are Q1,Q2, and Q3, respectively. .

MOTION-PARABOLA-EXERCISE - IV

In, the charges on $C_{1}, C_{2}$ , and $C_{3}$ , are $Q_{1}, Q_{2}$ , and $Q_{3}$ , respectively.
.