(1−1n+1)+(1−2n+1)+(1−3n+1)+…….+(1−nn+1) का योग ज्ञात करें।
n
12n
(n+1)
12(n+1)
The correct Answer is:B
(1−1n+1)+(1−2n+1)+
=(1−3n+1)+………….+(1−n(n+1))
=n−1+2+3……….nn+1
=n−n(n+1)2(n+1)
{1+2+3………….n=n(n+1)2}
=n=n2=n2
Topper's Solved these Questions
Similar Questions
What sum of money must be given at simple interest for six months at 4% per annum in order to earnn 150 interest(रु 150 बियाज प्राप्त करने के लिए कितने रूपए को 6 महीने करने के लिए 4 % बियाज दर से साधारण बियाज पर देना होगा )
04:25View SolutionA motorist and a scooterist made a journey of 120 km at the same time and from the same place. The graph shows the progress of the journey made by each person. Study the graph and answer the question.
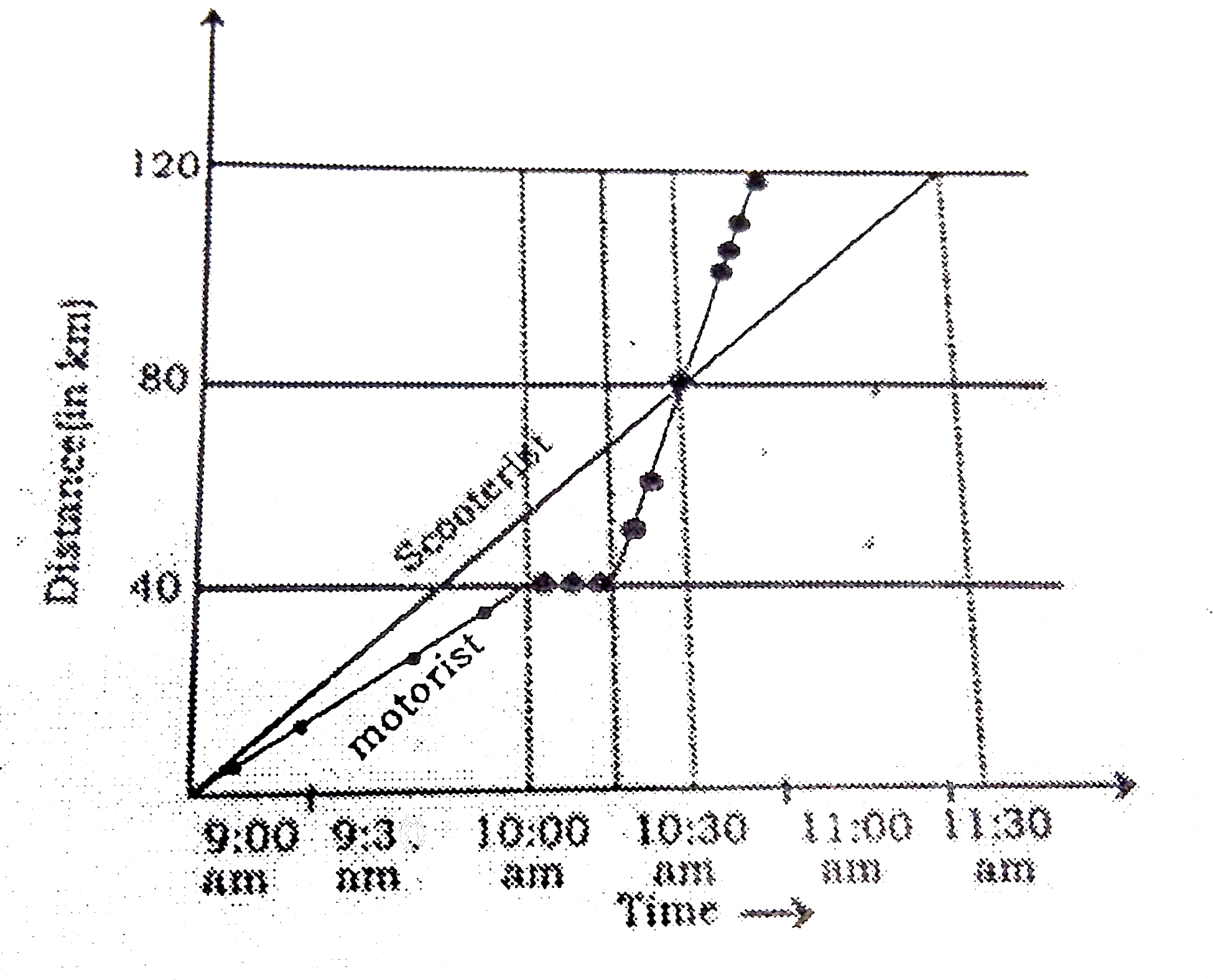
किस समय पर मोटर साइकिल वाला स्कूटर चालक से मिला ?03:17View SolutionIf A=⎡⎢⎣111111111⎤⎥⎦, prove that An=⎡⎢ ⎢⎣3n−13n−13n−13n−13n−13n−13n−13n−13n−1⎤⎥ ⎥⎦,n∈N.
02:32View SolutionIf A,=[[1,1,11,1,11,1,1]]A^(n)=,[[3^(n-1),1]]3^(n-1),3^(n-1),3^(n-1)3^(n-1),3^(n-1),3^(n-1)]]
04:51View SolutionThe sequence where a_(n)=(1)/(n+1)+(1)/(n+2)+(1)/(n+3)+......+(1)/(n+n) is
00:48View SolutionThe sum of the series 11!(n−1)!+13!(n−3)!+15!(n−5)!+…..+1(n−1)!1! is = (A) 1n!2n (B) 2nn! (C) 2n−1n! (D) 1n!2n−1
03:25View Solution((n+2)!+(n+1)(n-1)!)/((n+1)(n-1)!)=
07:07View SolutionSimplify: (3^(n+1))/(3^(n(n-1)))-:(9^(n+1))/((3^(n+1))^((n-1)))
05:17View Solutionयदि A = ⎡⎢⎣111111111⎤⎥⎦, तो सिद्ध कीजिए कि An=⎡⎢ ⎢⎣3n−13n−13n−13n−13n−13n−13n−13n−13n−1⎤⎥ ⎥⎦ , n∈N
02:24View SolutionIf 1⋅1!+2⋅2!+3⋅3!+...+n⋅n!=(n+1)!−1 then show that, 1⋅1!+2⋅2!+3⋅3!+...+n⋅n!+(n+1)(n+1)!=(n+2)!−1
05:08View SolutionLet Sn=1+12+13+….+1n Show that nsn=n+(n−11+n−22+………+2n−2+1n−1).
08:27View Solutionनिम्नलिखित श्रेणियों का n पदों तक योग ज्ञात कीजिए :
11.2.3+12.3.4+13.4.5+…..............05:10View SolutionEvaluate :
limn→∞[(1+1n)(1+2n)(1+3n)…(1+nn)]1n04:36View Solution121.2+12+222.3!+12+22+323.4!+….+12+22+…+n2n.(n+1)!+…∞ का योग होगा।
04:21View SolutionIf A=⎡⎢⎣111111111⎤⎥⎦, prove that An=⎡⎢ ⎢⎣3n−13n−13n−13n−13n−13n−13n−13n−13n−1⎤⎥ ⎥⎦,n∈N.
09:25View Solution

