If a,b,c are in G.P. and a1/x=b1/y=c1/z, then xyz are in AP (b) GP (c) HP (d) none of these
Video Solution
Text Solution
Let a1x=b1y=c1z=p
a=px
b=py
c=pz
b2=ac (since a,b,c in GP)
(py)2=pxpz
p2y=px+z
Equating powers
2y=x+z
So x,y,z are in AP.
thus,(1) is the answer.
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
If a, b c are in GP and a1x=b1y=c1z, then x, y, z are in
AAPBGPCHPDNone of theseIf a, b c are in GP and a1x=b1y=c1z, then x, y, z are in
AAPBGPCHPDNone of theseIf x,y and z are in G.P. and ax=by=cz then
Alogba=logacBlogcb=logacClogba=logcbDnone of these
If x,y,z are in GP andax=by=cz then
View SolutionIf a, b, c are in G.P. and a1x=b1y=c1z,prove that x, y, z are in A.P.
View Solution29. If a,b,c are in G.P. and a1x=b1y=c1z prove that x,y,z are in A.P.
View Solution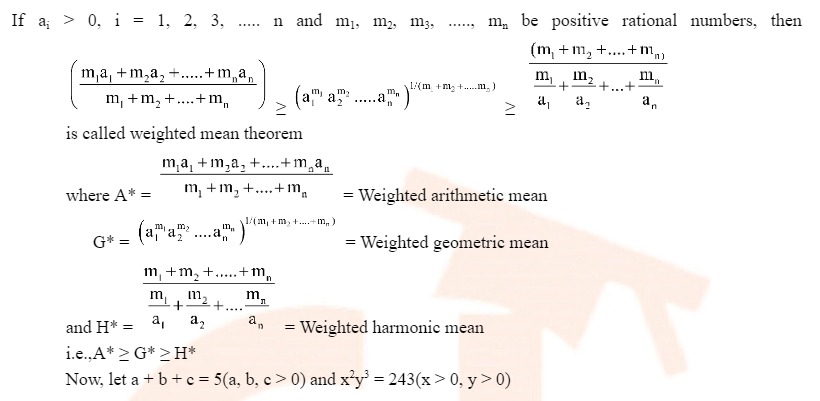
1. The greatest value of ab3c is -
(a) 3
(b) 9
(c) 27
(d) 81
2. Which statement is correct -
(a) 15≥11a+3b+1c
(b) 125≥11a+9b+1c
(c) 15≥11a+9b+1c
(d) 125≥11a+6b+1c
3. The least value of x2+3y+1 is -
(a) 15
(b) greater than 15
(c) 3
(d) less than 15
View SolutionIf x, y ,z are in G.P. and ax=by=cz , then :
View Solution