A straight wire carrying a current of 12A is bent into a semicircular arc of radius 2⋅0cm as shown in figure. Consider the magnetic field →B at the centre of arc.
(a) What is the magnetic field due to the staight segments?
(b) In what way the contribution to →B from the semicircle differs from that of a circular loop and in what way does it resemble?
(c) Would your answer be different if the wire were bent into a semicircle arc of the same radius but in the opposite way as shown in figure
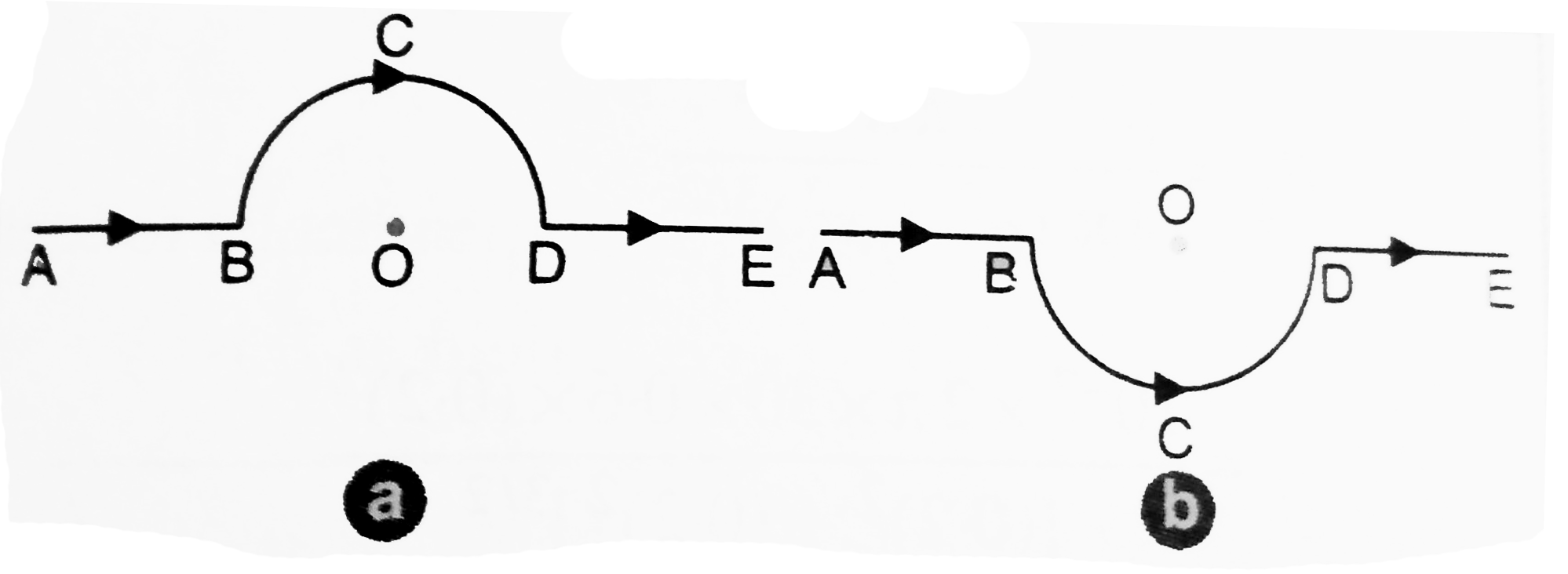
(a) dl and r for each element of the straight segments are parallel. Therefore, dl×r=0. Straight segments do not contribute to |B|.
(b) For all segments of the semicircular arc, dlx×r are all parallel to each other (into the plane of the paper). All such contributions add up in magnitude. Hence direction of B for a semicircular arc is given by the right-hand rule and magnitude is half that of a circular loop. Thus B is 1.9×10–4T normal to the plane of the paper going into it. (c) Same magnitude of B but opposite in direction to that in (b).
Topper's Solved these Questions
Similar Questions
A straight wire carrying a current of 13 A is bent into a semi-circular arc of radius 2 cm as shown in figure. The magnetic field is 1.5×10−4T at the centre of arc, then the magnetic field due to straight segment is

View SolutionA straight wire carrying a current of 5 A is bent into a semicircular arc of radius 2 cm as shown in the fig. Find the magnitude and direction of the magnetic field at the centre of the arc.

View Solution
Knowledge Check
A straight wire carrying a current 10A is bent into a semicircular arc of radius 5cm. The magnitude of magnetic field at the center is
A1.5×10−5TB3.14×10−5TC6.28×10−5TD19.6×10−5TA part of a long wire carrying a current i is bent into a circle of radius r as shown in figure. The net magnetic field at the centre O of the circular loop is
 Aμ0i4rBμ0i2rCμ0i2πr(π+1)Dμ0i2πr(π−1)
Aμ0i4rBμ0i2rCμ0i2πr(π+1)Dμ0i2πr(π−1)What is the magnetic field at the centre of arc in the figure below?
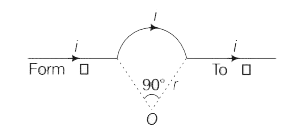 Aμ04π⋅2ir[√2+π]Bμ04π⋅2ir[√2+π4]Cμ04π⋅ir[√2+π]Dμ04π⋅ir[√2+π4]
Aμ04π⋅2ir[√2+π]Bμ04π⋅2ir[√2+π4]Cμ04π⋅ir[√2+π]Dμ04π⋅ir[√2+π4]
Magnetic field at the centre of a circular loop & arc
View SolutionA straight wire carrying a current of 12 A is bent into a semi-circular are of radius of2.0 cm as shown in the adjoining figure (a). Consider the magnetic field B at the centre of the arc. Would your answer be different if the wire were bent into a semi-circular arc of the same radius but in the opposite way a shown in figure (b)?

View SolutionA piece of wire carrying a current of 6.00 A is bent in the form of a circular arc of radius 10.0 cm, and it subtends an angle of 120∘ at the centre. Find the magnetic field B due to this piece of wire at the centre.
View SolutionA current of 10A is passing through a long wire which has semicircular loop of the radius 20cm as shown in the figure. Magnetic field produced at the centre of the loop is

View SolutionA long straight conductor carrying a current i is bent to form an almost complete circular loop of radius r on it. The magnetic field at the centre of the loop
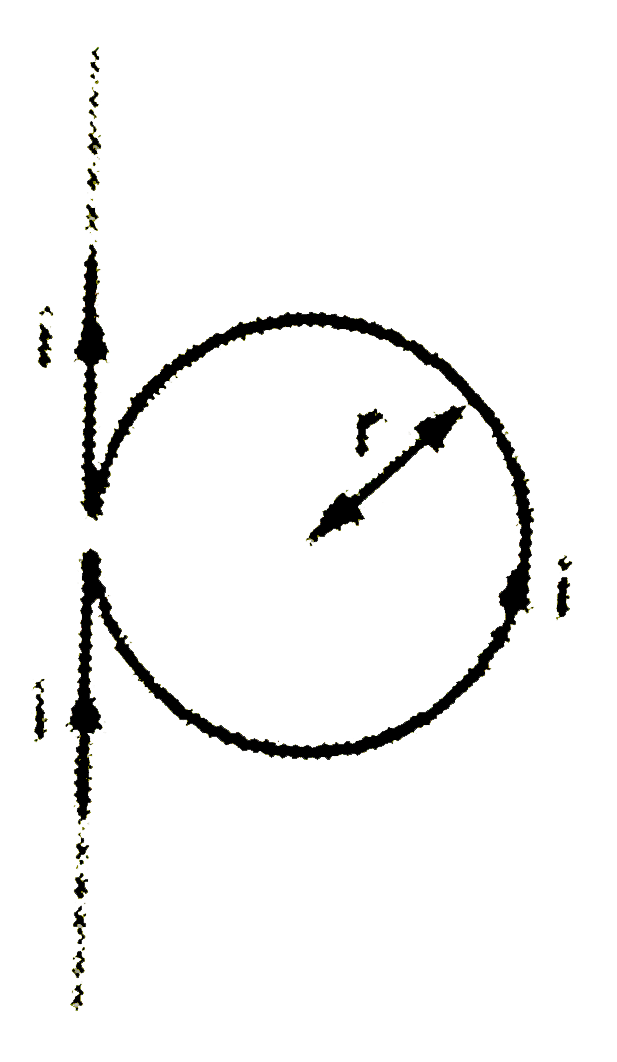
View Solution
