A cyclist starts from the center O of a circular park of radius 1km, reaches the edge P of the park, then cycles along the PQ circumference and returns to the center along OQ as shown in fig. If the round trip taken ten minute, the (a) net displacement, (b) average velocity and (c) average speed of the cyclists (in kilometer and kinetic per hour) is
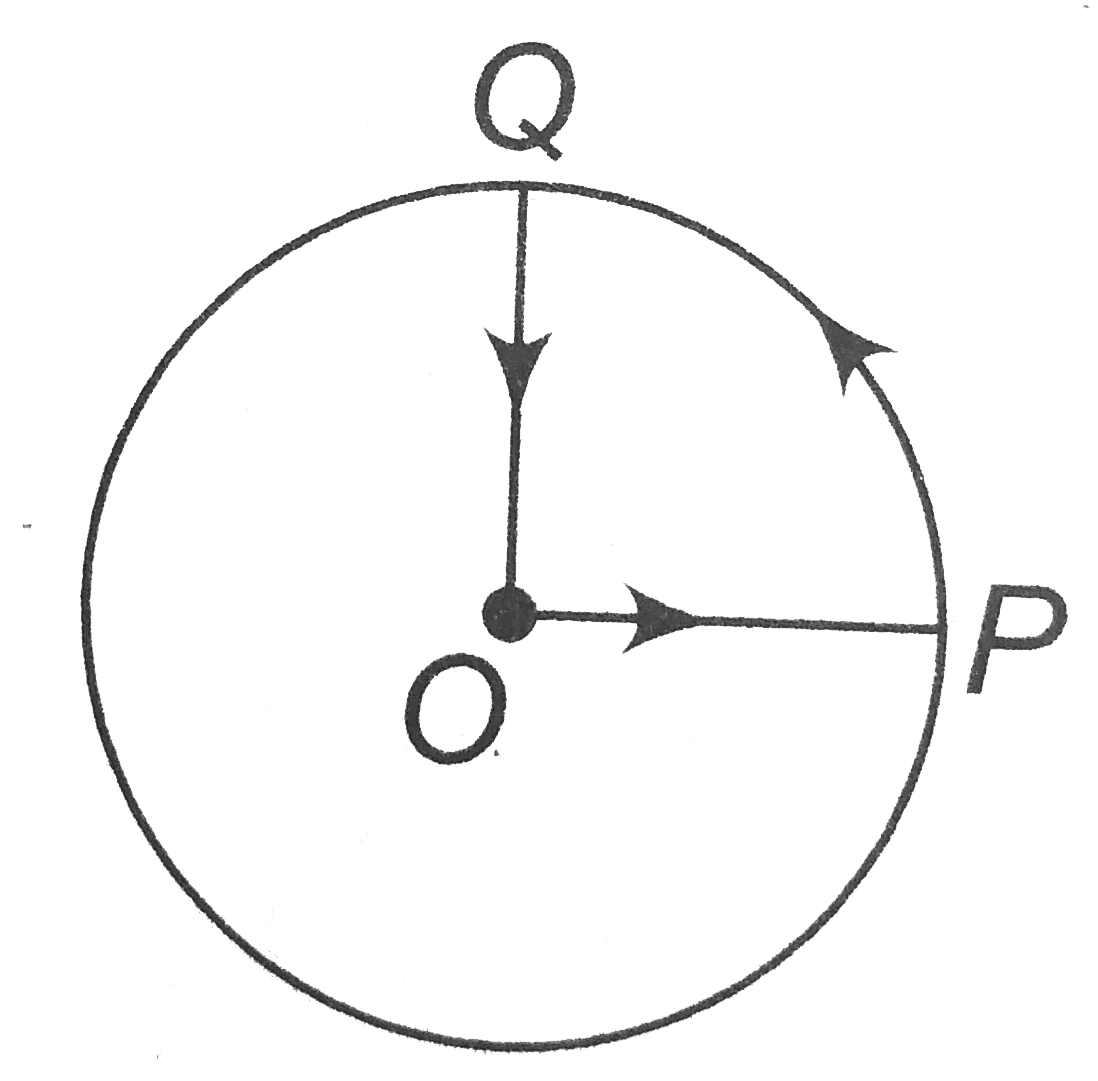
a) Since both the initial and final positions are the same therefore the net displacement is zero.
b) Average velocity is the ratio of net displacement and total time taken. Since the net displacement is zero therefore the average velocity is also zero.
c) Average speed = distance coveredtime taken
=(OP+Actual distance PQ + OQ)/(10minutes)
1km+14×2π×1km+1km10/60h
6(2+2214)kmh−1 = 6×5014kmh−1
=21.43kmh−1
Topper's Solved these Questions
Similar Questions
A cyclist starts from the centre O of a circular park of radius 1km , reaches the edge P of the park , then cycles along the circumference and returns to the centre along QO as shown in figure . If the round trip takes 10 min. what is the (a) net displacement . (b) average velocity and (c) average speed of the cyclist ?
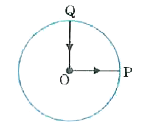
View SolutionA cyclist travels from centre O of a circular park of radius 1 km and reaches point P. After cycling along 1/4 th of the circumference along PQ, he returns to the centre of the park along QO. If the total time taken is 10 minutes, calculate (i) net displacement (ii) average velocity and (ii) average speed of the cyclist.
View Solution
Knowledge Check
A cyclist starts from the centre O of a circular park of radius 1km, reaches the edge P of the park, then cycles along the PQ cicumference and returns to the centre along OQ as shown in fig. If the round trip taken ten minute, the net displacement and average speed of the cylists (in kilometer and kinetic per hour) is
 A0,2(π+4)Bπ+42,0C21.4,π+42D0,3(π+4)
A0,2(π+4)Bπ+42,0C21.4,π+42D0,3(π+4)A cyclist starts from the centre O of circular path of radius 10 m, covers the radius of circular path and reaches at point X on circumference, then cycles along the semi-circular path and reaches the point Y. If he takes 10 minutes to go from O to Y via X, then the net displacement and average speed of the cyclist would be
AZero,10ms−1B10m,(1+π60)ms−1C10m,(1+π6)ms−1D10m,(1−π60)ms−1One end of a rod of length L=1 m is fixed to a point on the circumference of a wheel of radius R=1/√3 m. The other end is sliding freely along a straight channel passing through the center O of the wheel as shown in the figure below. The wheel is rotating with a constant angular velocity ω about O.

The speed of the sliding end P when θ=60∘ isA2ω3Bω3C2ω√3Dω√3
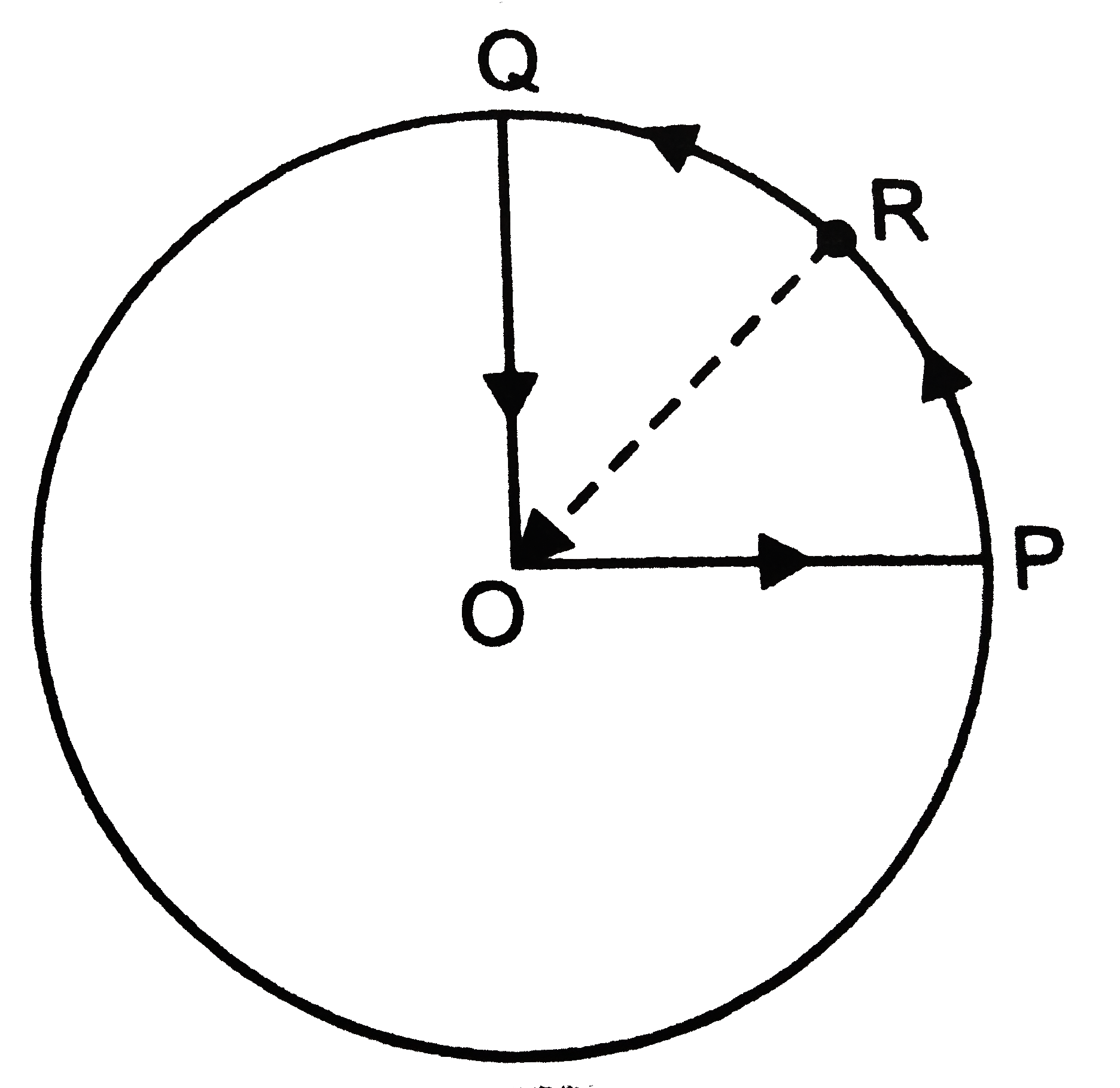
A cyclist starts form centre O of a circular park of radius 1km and moves along the path OPRQO as shown Fig. 2 (EP).15. If he maintains constant speed of 10ms−1, what is his acceleration at point (R )in magnitude and direction ?
 .
.
View SolutionA cyclist starts form centre O of a circular park oa radius 1km and moves along the path OPRQO as whown Fig. 2 (EP).15. If he maintais constant speed of 10ms−1, what is his acceleration at point (R )in magnitude and direction ?
 .
.
View SolutionA cyclist moves along a circular path of radius 70 m. If he completes one round in 11 s, calculate (i) total length of path, (ii) magnitude of the displacement, (iii) average speed, and (iv) magnitude of average velocity.
View SolutionA girl is jogging along a circular path of radius. 25. 0 m. In 1 110.0 second she jogs a. quarter ofa circle starting from point1 P. (a) Compute her displacement and average velocity, and (b) Compute the magnirude of the runner's average velocity and her average speed.
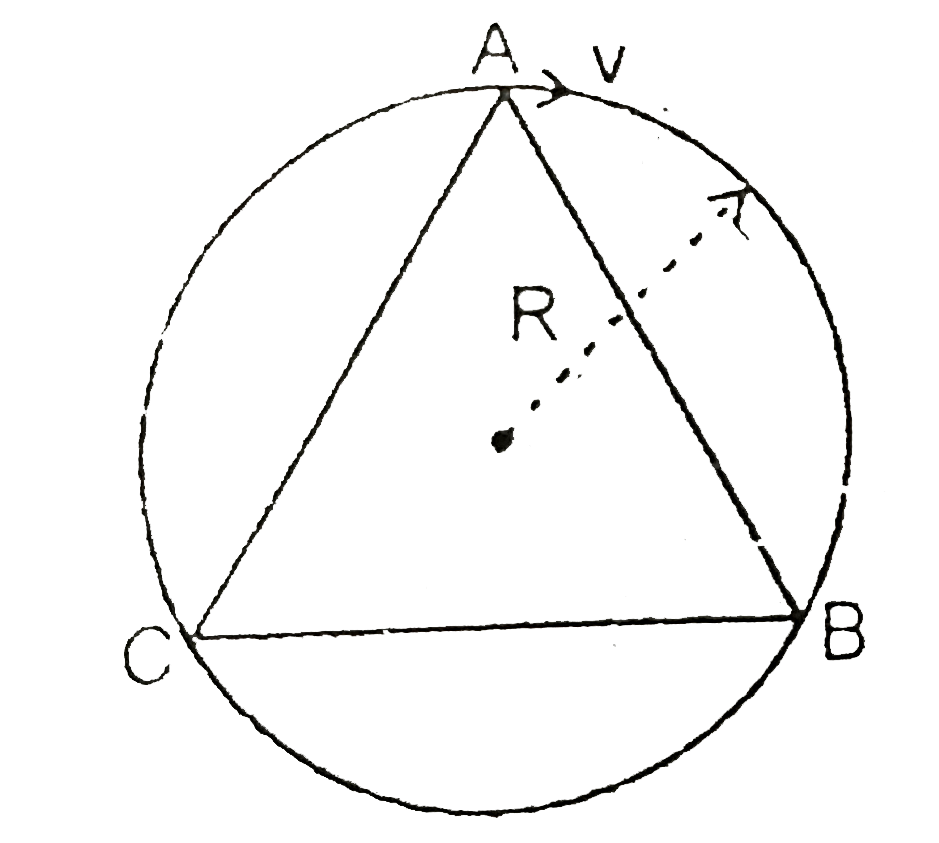
View SolutionA particle is moving with constant speed V m/s along the circumference of a circle of radius R meters as shown. A, B and C are three points on periphery of the circle and ΔABC is equilateral. The magnitude of average velocity of particle, as it moves from A to C in clockwise sence, will be :
View Solution
