An inductor of inductance L = 5 H is connected to an
AC source having voltage v = 10sin(10t+π6)
Find
Intstantatious current (I(t))
I(t)=15sin(10t+π6−π2).
Topper's Solved these Questions
- ALTERNATING CURRENTBOOK - MOTIONCHAPTER - ALTERNATING CURRENTEXERCISE - EXERCISE - 1
20 Videos
- ALTERNATING CURRENTBOOK - MOTIONCHAPTER - ALTERNATING CURRENTEXERCISE - EXERCISE - 2 (LEVEL - I)
19 Videos
- 10 ROTATIONALBOOK - MOTIONCHAPTER - 10 ROTATIONALEXERCISE - Exercise - 4 (Level - II)
49 Videos
- ATOMIC STRUCTURE & X-RAY BOOK - MOTIONCHAPTER - ATOMIC STRUCTURE & X-RAY EXERCISE - Exercise - 2
31 Videos
Similar Questions
Knowledge Check
A coil of inductance 5.0 mH and negligible resistance is connected to an alternating voltage V=10sin(100t). The peak current in the circuit will be:
A2ampB1ampC10ampD20ampA coil of inductance 5.0 mH and negligible resistance is connected to an alternating voltage V=10sin(100t). The peak current in the circuit will be:
A2 ampB1 ampC10 ampD20 ampAn inductor has a resistance R inductance L. It is connected to an A.C source of e.m.f. EV and angular frequency ω then the current Iv in the circuit is
AEVωLBEVRCEV√R2+ω2L2D√(EVR)2+(EVωL)2
An inductor of inductance L = 5 H is connected to an
AC source having voltage v = 10sin(10t+π6)
Find
Peak & Rms voltage (V0 & Vrms)
View SolutionAn ideal inductor of inductance 50μH is connected to an AC source of 220V, 50 Hz. Find the inductive reactance.
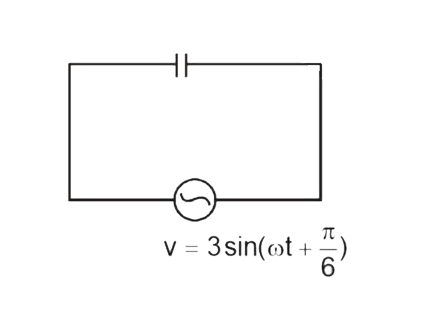
View SolutionA capacitor of capacitive reactance 5ω is connected with A.C. source having voltage V=3sin(ωt+π/6). Find rms and Peak voltage rms and peak current and instantaneous current
View SolutionAn inductor of inductance L Henry is connected to an AC supply of 12 V . The frequency of source is 50 Hz and current in the inductor is 0.5 A. When the same inductor is connected across a direct current supply of 12 V it draws the current of 1.5 A . Find the value of L .
View SolutionAn inductor of inductance 10 mH and a resistance of 5 is connected to a battery of 20 V at t = 0. Find the ratio of current in circuit at t = to current at t = 40 sec
View Solution