In a thermally isolated container, material A of mass m is placed against material B, also of mass m but at higher temperature. When thermal equilibrium is reached, the temperature changes of A and B are recorded. Then the experiment is repeated, using A with other materials. All of the same mass m. The results are given in the table. Rank the four materials according to their specific heats, greatest first.
The correct Answer is:
Step by step video, text & image solution for In a thermally isolated container, material A of mass m is placed against material B, also of mass m but at higher temperature. When thermal equilibrium is reached, the temperature changes Delta T_A and Delta T_B of A and B are recorded. Then the experiment is repeated, using A with other materials. All of the same mass m. The results are given in the table. Rank the four materials according to their specific heats, greatest first. {:("Experiment", "Temperature","Changes"),(1., DeltaT_A=+50 C^@,DeltaT_B=-50 C^@),(2.,Delta T_A=+10 C^@,Delta T_C=-20 C^@),(3.,Delta T_A=+2 C^@,Delta T_D=-40 C^@):} by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- HEAT - 1Book:MOTIONChapter:HEAT - 1Exercise:EXERCISE -3 (Level - II) Subjective | JEE Advanced
Explore 11 Videos
- HEAT - 1Book:MOTIONChapter:HEAT - 1Exercise:EXERCISE -4 (Level - I) Previous Year | JEE Main
Explore 10 Videos
- HEAT - 1Book:MOTIONChapter:HEAT - 1Exercise:EXERCISE -2 (Level - II) Multiple Correct | JEE Advanced
Explore 16 Videos
- GRAVITATIONBook:MOTIONChapter:GRAVITATIONExercise:Exercise - 4 Section - B Previous Years Problems
Explore 15 Videos
- HEAT TRANSFER & THERMAL EXPANSION Book:MOTIONChapter:HEAT TRANSFER & THERMAL EXPANSION Exercise:Exercise - 3 Section-B
Explore 19 Videos
Similar Questions
The figure shows the face and interface temperatures of a composite slab consisting of four materials, of identical thickness, through which the heat transfer is steady. Rank the materials according to their thermal conductivities, greatest first.

View SolutionMaterials A, B and C are solids that are at their melting temperatures. Material A requires 200 J to melt 4 kg, material B requires 300 J to melt 5 kg, and material C requires 300 J to melt 6 kg. Rank the materials according to their heats of fusion, greatest first.
View Solution
Knowledge Check
When a ferromagnetic material is heated above its Curie temperature , the material
ABecomes permanently magnetizedBBecomes diamagneticCBecomes paramagneticDRemains ferromagneticThe magnetic susceptibility (x) of a paramagnetic material changes with absolute temperature (T) as
Ax∝TBx∝1TCx∝T2Dx∝e−TThe magnetic susceptibility of any paramagnetic material changes with absolute temperature T as
Adirectly proportional to TBRemains constantCInversely proportional to TDExponentially decaying with T
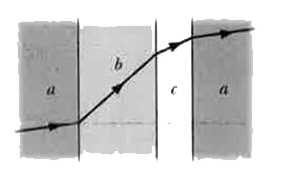
The figure shows a monochromatic ray of light traveling across parallel interfaces, from an original material a, through layers of materials b and c, and then back into material a. Rank the materials according to the speed of light in them, greatest first.
View SolutionThe magnetic susceptibility of ant paramagnetic material changes with absolute temperature T as:
View SolutionThe magnetic susceptibility of ant paramagnetic material changes with absolute temperature T as:
View SolutionD.M.T. the raw material of terylene is called
View SolutionWhen a ferromagnetic material is heated to temperature above its Curie tamperature, the material
View Solution