In example 3,K1=0.125W/m−.∘C,K2=5K1=0.625W/m−.∘C and thermal conductivity of the unknown material is K=0.25W/m∘CL1=4cm,L2=5L1=20cm. If the house consists of a single room of total wall area of 100m2. then find the power of the electric heater being used in the room.
R1=R2=(4×102m)(0.125w/m−∘C)(100m2)
=32×10−4∘C/w
R=(10×10−2m)(0.25W/m−∘C)(100m2)=40×10−4∘C/w
the equivalent thermal resistance of the entire wall
=R1+R2+2R=144×10−4∘C/W
∴ Net heat current, i.e. amount of heat flowing out of the house per second =TH−TCR
=25∘C−(−20∘C)144×10−4∘C/w=45×104144 watt
Hence the heater must supply 3.12 kW to compensate for the outflow of heat.
Topper's Solved these Questions
- HEAT - 1Book:MOTIONChapter:HEAT - 1Exercise:EXERCISE - 1 Objective Problems | JEE Main
Explore 39 Videos
- HEAT - 1Book:MOTIONChapter:HEAT - 1Exercise:EXERCISE -2 (Level - I) Objective Problems | JEE Main
Explore 24 Videos
- GRAVITATIONBook:MOTIONChapter:GRAVITATIONExercise:Exercise - 4 Section - B Previous Years Problems
Explore 15 Videos
- HEAT TRANSFER & THERMAL EXPANSION Book:MOTIONChapter:HEAT TRANSFER & THERMAL EXPANSION Exercise:Exercise - 3 Section-B
Explore 19 Videos
Similar Questions
Knowledge Check
Figure shows in cross section a wall consisting of four layers with thermal conductivities K1=0.06W/mK,K3=0.04W/mKandK4=0.10W/mK. The layer thickness are L1=1.5cm,L3=2.8cmandL4=3.5cm. The temperature of interfaces is as shown in figure. energy transfer through the wall is in steady state. the temperature of the interface between layer 3and4 is:
 A−1∘CB−3∘CC2∘CD0∘C
A−1∘CB−3∘CC2∘CD0∘CConductivity k, is equal to ………….
1) 1R.lA
2) GA
3) Λm
4) lAA1 & 2B2 & 3C3 &4D1 & 4If layer thickness L2 is 1.4cm, then its thermal conductivity K2 will have value (in W/mK)
A2×10−2B2×10−3C4×10−2D4×10−3
The thermal conductivity of brick is 1.7Wm−1K−1, and that of cement is 2.9Wm−1K−1.What thickness of cement will have same insulation as the brick of thickness 20cm.
View SolutionFigure shows the cross section of a wall made of three layers. The layer thicknesses are L1,L2=0.750L1, and L3=0.350L1. The thermal conductivities are k1,k2=0.900k1, and k3=0.800k1. The temperature at the left side and right side of the wall are TH=30.0∘C and TC=−15.0∘C, respectively. Thermal conduction is steady . (a) What is the temperature difference ΔT2 across layer 2 ( between the left and right sides of the layer ) ? If k2 were, instead, equal to 1.1k1, (b) would the reate at which energy is conducted through the wall be greater than, less than, or the same as proviously, and (c ) what would be the value of ΔT2 ?
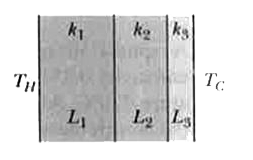
View SolutionThe molar conductivity of 0.05 M BaCl2 solution at 25∘C is 223Ω−1cm2mol−1. What is its conductivity?
View SolutionFind the centroid of tetrahedron with vertices K(5, -7, 0), L(1, 5, 3), M(4, -6, 3), N(6, -4, 2) ?
View SolutionIf the length (l) (in m) and width (w) (in m) of a room are related by the expresion w=12l+1, then the width of the room is
View Solution