A ball is dropped from a height h on to a floor. If in each collision its speed becomes e times of its striking value (a) find the time taken by ball to stop rebounding (b) find the total change in momentum in this time (c) find the average force exerted by the ball on the floor using results of part (a) and (b).

Video Solution

Text Solution

Verified by Experts

(a) When the ball is dropped from a height h, time taken by it to reach the ground will be
$t_{0} = \sqrt{\frac{2 h}{g}}$ and its speed $v_{0} = \sqrt{2 g h}$

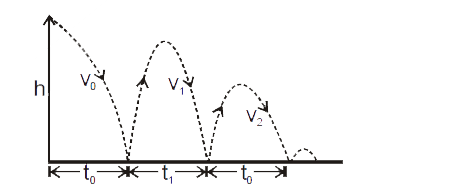
Now after collision its speed will becomes e times, i.e., $v_{1} = e v_{0} = e \sqrt{2 g h}$ and so, it will take time to go up till its speed becomes zero $= (v_{1} / g)$ . The same time it will take to come down. So total time between I and II collision will be $t_{1} = 2 v_{1} / g .$ Similarly, total time between II and III collision $t_{2} = 2 v_{2} / g$ .
So total time of motion
$Δ T = t_{0} + t_{1} + t_{2} +$ .......
or $Δ T = t_{0} + \frac{2 v_{1}}{g} + \frac{2 v_{2}}{g}$ .....
or $Δ T = t_{0} + \frac{2 e v_{0}}{g} + \frac{2 e^{2} v_{0}}{g}$ .......
[as $v_{2} = e v_{1} = e^{2} v_{0}$ ]
or $Δ T = \sqrt{\frac{2 h}{g}} [1 + 2 e (1 + e + e^{2} + ... . .)]$
$= \sqrt{\frac{2 h}{g}} [1 + 2 e (\frac{1}{1 - e})] = \sqrt{\frac{2 h}{g}} [\frac{1 + e}{1 - e}]$
(b) Change in momentum in I collision
$= m v_{1} - (- m v_{0}) = m (v_{1} + v_{0})$
Change in momentum in II collision = $m (v_{2} + v_{1})$
Change in momentum in nth collision $= m (v_{n} + v_{n - 1})$
Adding these all total change in momentum
$Δ p = m [v_{0} + 2 v_{1} + ... . . + 2 v_{n - 1} + v_{n}]$
or $Δ p = m v_{0} [1 + 2 e + e^{2} + ... ...]$
or $Δ p = m v_{0} [1 + 2 e (\frac{1}{1 - e})] = m \sqrt{2 g h} [\frac{1 + e}{1 - e}]$
....(2)
(c) Now as $\to F = \frac{d \to p}{d t}$ so, $F_{a v} = \frac{Δ p}{Δ T}$
Substituting the value of $Δ T$ and $Δ p$ from Eqns. (1) and (2)
$F_{a v} = m \sqrt{2 g h} [\frac{1 + e}{1 - e}] \times \sqrt{\frac{g}{2 h}} [\frac{1 - e}{1 + e}] = m g$ ...(3)

Updated on:21/07/2023

A ball is dropped from a height 'h' on to a floor of coefficient of restitution 'e'. The total distance covered by the ball just before second hit is
A $h (1 - 2 e^{2})$
B $h (1 + 2 e^{2})$
C $h (1 + e^{2})$
D $h e^{2}$
If a ball is dropped from height 2 metre on a smooth eleastic floor, then the time period of oscillation is
A $\frac{2}{g}$
B $\frac{2}{\sqrt{g}}$
C $\frac{4}{g}$
D $\frac{4}{\sqrt{g}}$
A ball of mass m is dropped from height h on a horizontal floor which collides with it with speed u. If coefficient of restitution in floor is e, then impulse imparted to ball by the floor on its second rebounce is
A $m e u$
B $m e u (e + 1)$
C $m e^{2} u (e + 1)$
D $m e^{2} u (e - 1)$