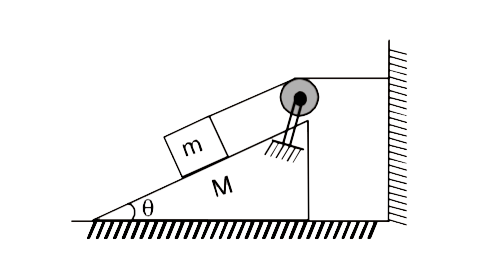
Find the acceleration of rod A and wedge B in the arrangement shown in fig if the ratio of the mass of wedge to that of the rod equals η and the friction between the contact surfaces are negligible.
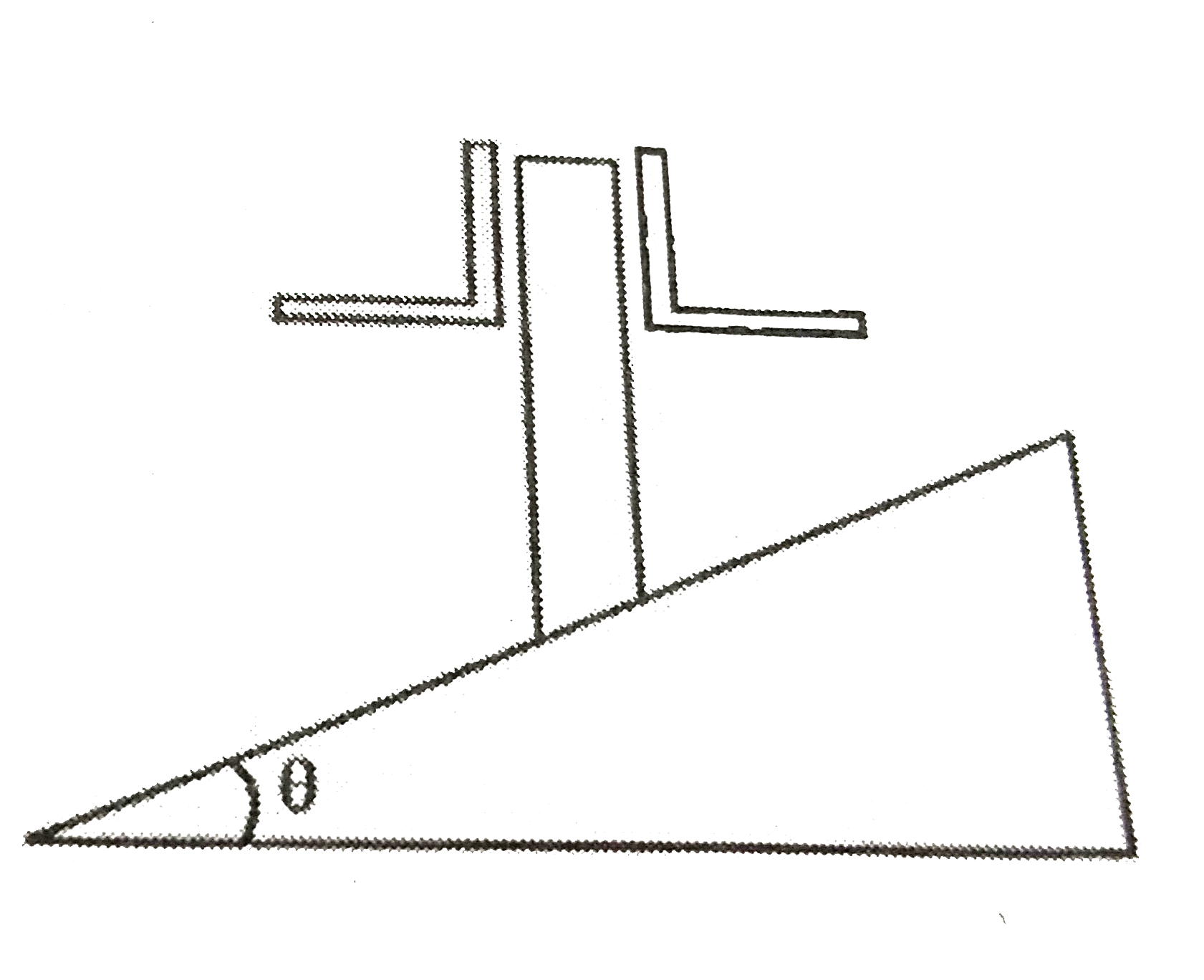

Let in any time the rod displaces down by y, the corresponding horizontal displacement of wedge will be x from the figure we have
y=xtanθ …(i)
Differentiating both sides of Eq. (i) we get
dydt=dxdttanθ
or vrod=vwedgetanθ ...(ii)
and arod=awedgetanθ ...(iii)
Let mass of the rod is m, then mass of wedge M=ηm
By newton second law
For vertical motion of rod
mg−Ncosθ=marod ....(iv)
For horizontal motion of wedge,
Nsinθ=Mawedge
=M(arodtanθ)....(v)
Solving Eqns (iv) and (v) we get
arod=g[1+Mmcot2θ]
=g[1+ηcot2θ]
and awedge=arodtanθ=g[tanθ+ηcotθ]
Topper's Solved these Questions
- FORCE AND NEWTONS LAWS OF MOTIONBOOK - GRB PUBLICATIONCHAPTER - FORCE AND NEWTONS LAWS OF MOTIONEXERCISE - Objective Questions
134 Videos
- FORCE AND NEWTONS LAWS OF MOTIONBOOK - GRB PUBLICATIONCHAPTER - FORCE AND NEWTONS LAWS OF MOTIONEXERCISE - More than one choice is correct
15 Videos
- FORCE AND NEWTONS LAWS OF MOTIONBOOK - GRB PUBLICATIONCHAPTER - FORCE AND NEWTONS LAWS OF MOTIONEXERCISE - Problem
33 Videos
- BASIC MATHEMATICSBOOK - GRB PUBLICATIONCHAPTER - BASIC MATHEMATICSEXERCISE - Problems For Practice
35 Videos
- FRICTION AND CIRCULAR MOTION BOOK - GRB PUBLICATIONCHAPTER - FRICTION AND CIRCULAR MOTION EXERCISE - Comprehension type
11 Videos
Similar Questions
Find the accelerations of rod A and wedge B in the arrangement shown in figure if the ratio of the mass of the wedge to that of the rod equals η, and the friction between all contact surfaces is negligible.

View SolutionFind the acceleration of rod A and wedge B in the arrangement shown in figure if the mass of rod equal that of the wedge and the friction between all contact surfaces is negligible Take angle of wedge as 45∘&g=9.8m/s2

View Solution
Knowledge Check
In the arrangement shown in fig. the block of mass m=2kg lies on the wedge of mass M=8kg. The initial acceleration of the wedge, if the surfaces are smooth, is
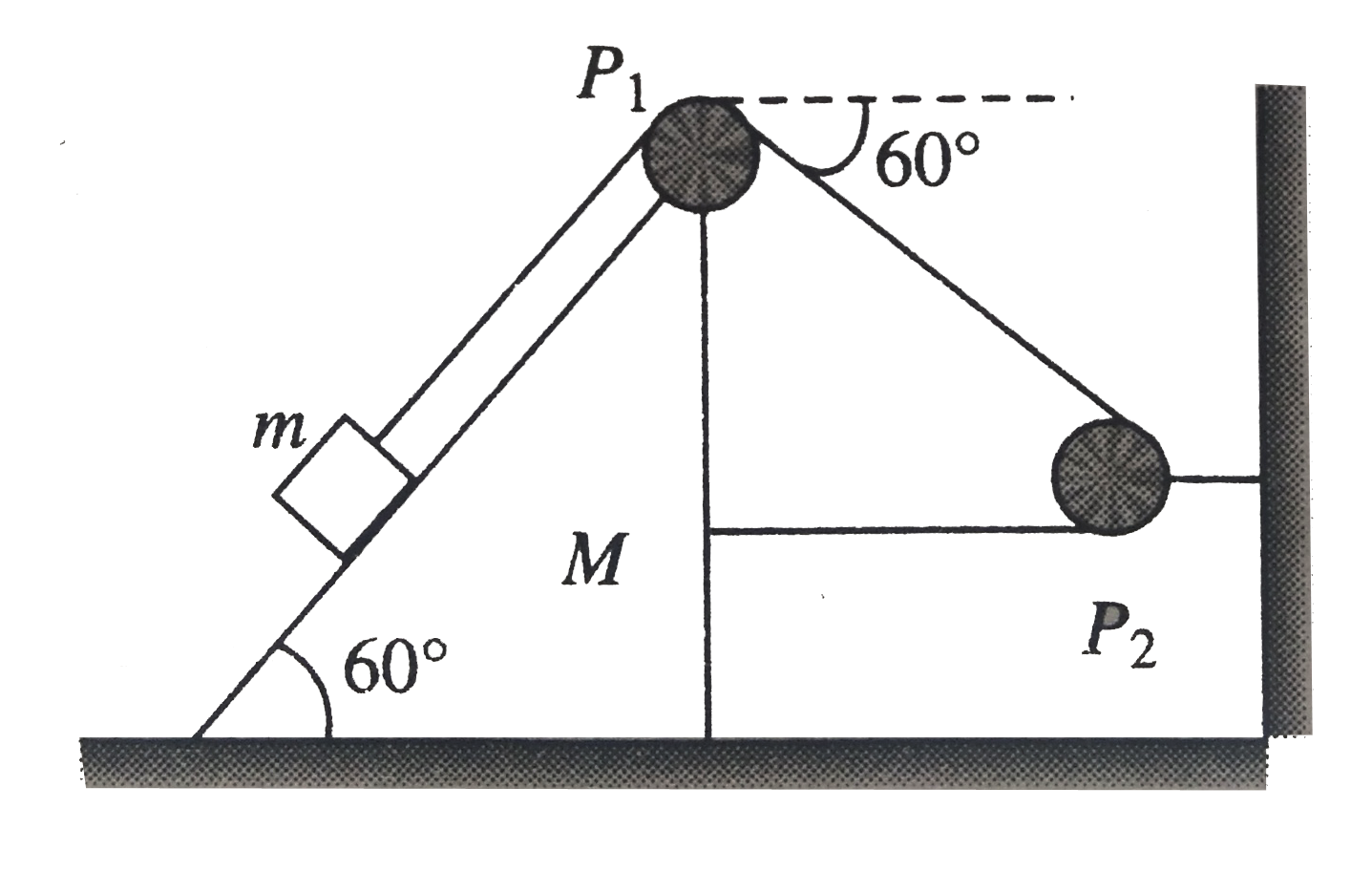 A√3g23ms−2B3√3g23ms−2C3g23ms−2Dg23ms−2
A√3g23ms−2B3√3g23ms−2C3g23ms−2Dg23ms−2In the system shown, the initial acceleration of the wedge of mass 5M is (there is no friction anywhere)
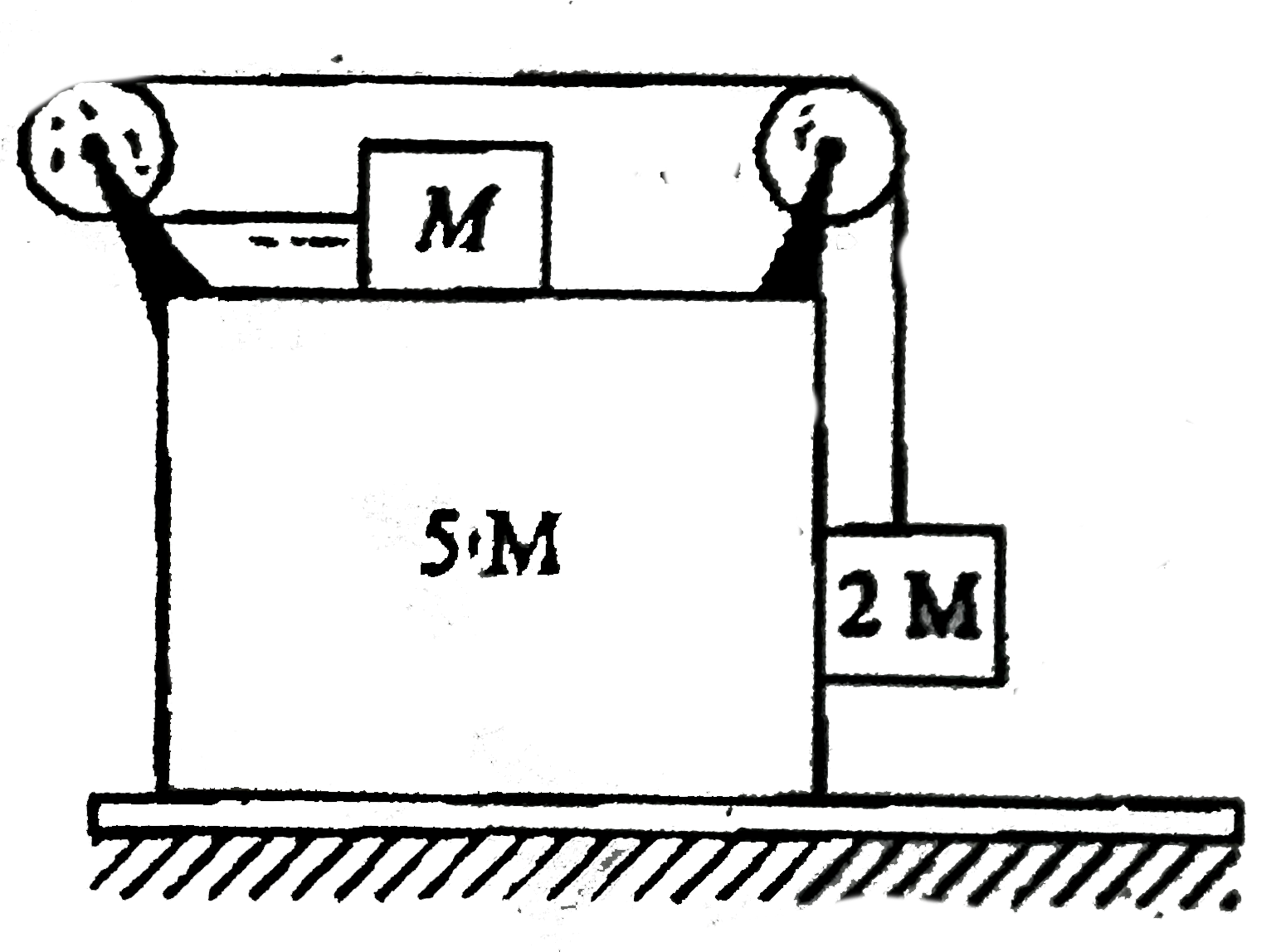 AZeroB2g/23C3g/23DNone
AZeroB2g/23C3g/23DNone
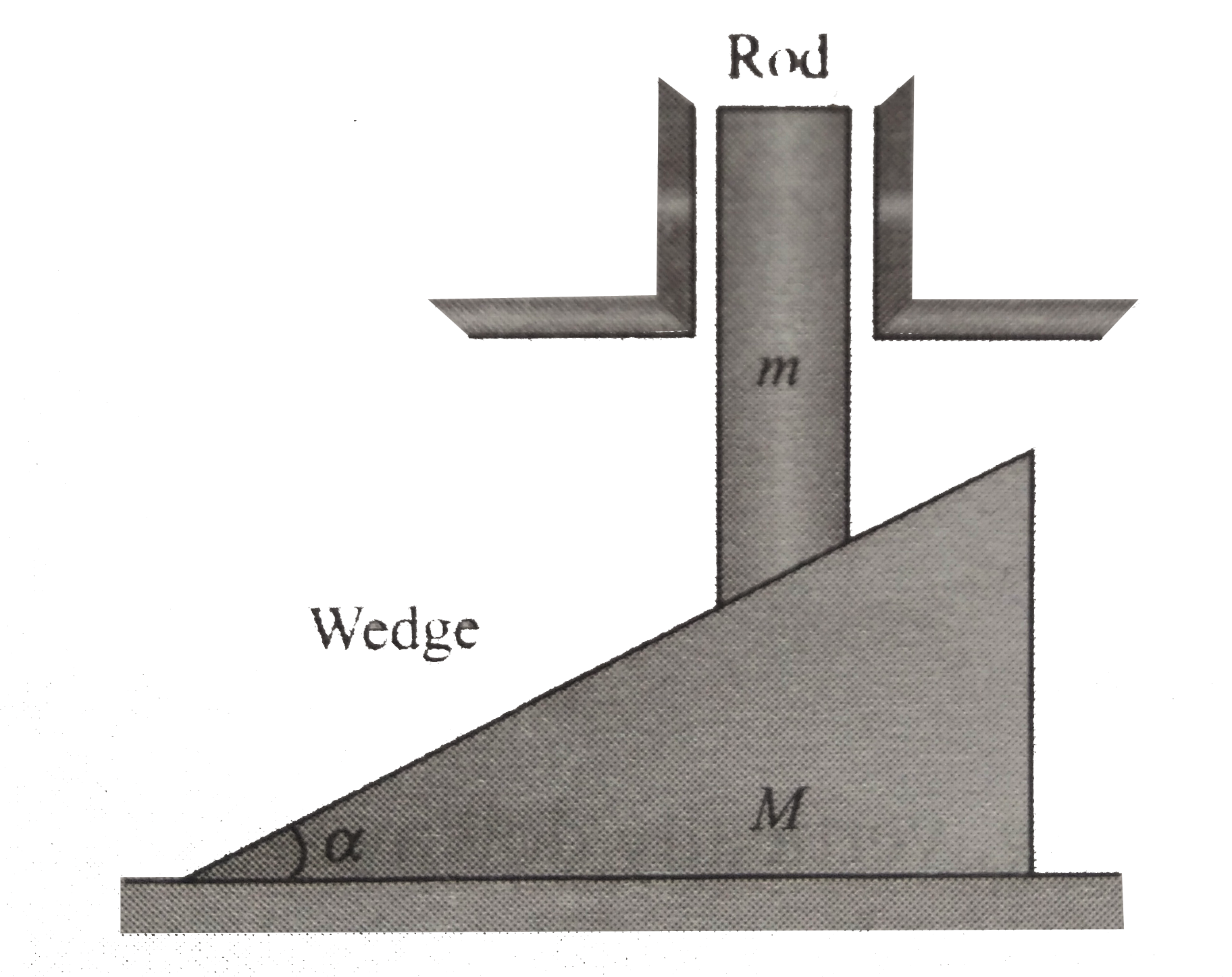
A rod of mass m is supported on a wedge of mass M shown in fig. Find the accelerations of rod a and wedge A in the arrangment. The friction between all contact surfaces in negligible.
View SolutionFind the acceleration of the block of mass m in the arrangement shown in the following figures. The masses of the pulleys and the threads, as well as the friction are assumed to be negligible.

View SolutionThe coefficient of friction between the wedge and the table is μ, the friction between the rod and the wedge being negligible.
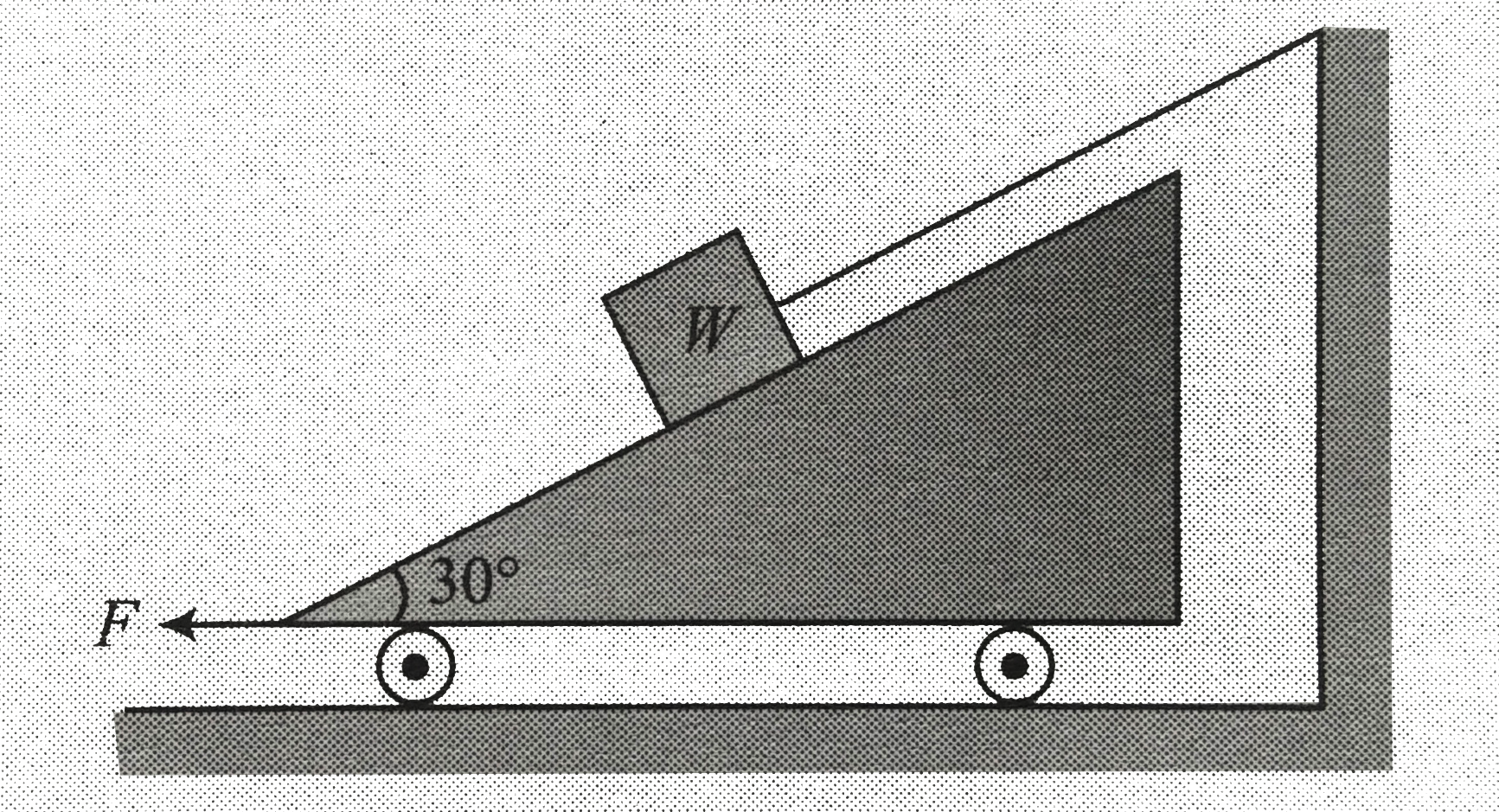
View SolutionA block of weight W is placed on a wedge and arranged as shown in fig. find the force F needed to hold the cart equilibrium if there is no friction.
View SolutionFor the arrangement shown in figure when the system is released, find the acceleration of wedge. Pulley and string are ideal and friction is absent.
View SolutionIn the arrangement shown in figure the mass of the ball is η times as that of the rod. The length of the rod is L the masses of the pulleys and the threats as well as the friction, are negligible. The ball is set on the same level as the lower and of the rod and then released. How soon will the ball be opposite the upper and of the rod?
View Solution
