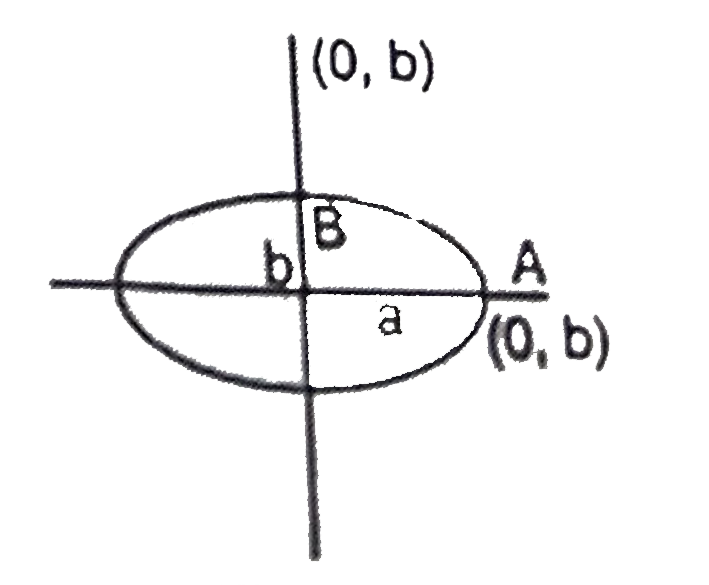
The coordinate of a particle moving in a plane are given by $x (t) = a cos (p t) and y (t) = b sin (p t)$ where $a, b (< a)$ and $P$ are positive constants of appropriate dimensions . Then

A

The path of the particle is an ellipse

B

The velocity and acceleration of the particles are normal to each toerh at t= $π / 2 p$ .

C

The acceleration of the particle is always directed towards a forucs

D

The distance travelled by the particle in time interval $t = 0$ to $t = π / 2 p$ is a.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:a,b

$x = α cos (p t) : y = b sin (p t)$
Equation of path in x-y plane is
${(\frac{x}{a})}^{2} + {(\frac{y}{b})}^{2} =$
i.e., the path of the particle is an elipse.
Position vector of a point P is
$\to r = a cos p t ˆ i + b sin p t ˆ j$
$\Rightarrow \to v = P (- a sin p t ˆ i + b cos p t ˆ j)$
and $\to a = - p^{2} (- a cos p t ˆ i + b sin p t ˆ j) = - p^{2} \to r$
Acceleration of directed towards the centre.
Also, $\to v, \to a = 0$ at $t = π / 2 p$
At $t = 0, \to r = a ˆ i$
At $t = π / 2 p, \to r = b ˆ j$
So, in time t=0 to $t = π / 2 p$ , particle goes from A to B travelling a distance more than a.

|

Updated on:21/07/2023

Class 11 PHYSICS PROJECTILE MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One or More
The coordinates of a particle moving in a plane are given by $x (t) = a cos (p t) and y (t) = b sin (p t) where a, b (< a) and p$ are positive constants of appropriate dimensions. Then
Athe path of the particle is an ellipse.
Bthe velocity and acceleration of the particle are normal to each other at $t = π / (2 p)$ .
Cthe acceleration of the particle is always directed toward a focus.
Dthe distance traveled by the particle in time interval t = 0 to $t = π / (2 p)$ is a.
Question 2 - Select One
The coordinates of a moving particle at any time t are given by x = ct and y = bt. The speed of the particle at time t is given by
A2t(c-b)
B $\sqrt{(c^{2} + b^{2})}$
C $2 t \sqrt{(c^{2} + b^{2})}$
D $2 t \sqrt{(c^{2} + b^{2})}$
Question 3 - Select One
The coordinates of a moving particle at any time t are given by $x = c t$ and $y = b t^{2}$ . The speed of the particle is given by
A $2 t \sqrt{b^{2} - c^{2}}$
B $\sqrt{4 b^{2} t^{2} + c^{2}}$
C $2 t (b + c)$
D $2 t (b - c)$

GRB PUBLICATION-MOTION IN TWO AND THREE DIMENSIONS-All Questions

Home
Ask Doubt
Profile