When a proton is released from rest in a room, it starts with an initial acceleration $a_{0}$ towards west. When it is projected towards north with a speed $v_{0}$ it moves with an initial acceleration $3 a_{0}$ towards west. The electric and magnetic fields in the room are:

A

$\frac{m a_{0}}{e} east, \frac{3 m a_{0}}{e v_{9}} down$

B

$\frac{m a_{0}}{e} west, \frac{2 m a_{0}}{e v_{0}} up$

C

$\frac{m a_{0}}{e} west, \frac{2 m a_{0}}{e v_{0}} down$

D

$\frac{m a_{0}}{e} east, \frac{3 m a_{0}}{e v_{0}} up$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:C

To solve the problem, we need to analyze the forces acting on the proton in two different scenarios: when it is released from rest and when it is projected towards the north with a speed $v_{0}$ .
Step 1: Analyze the first scenario (proton released from rest)
When the proton is released from rest, it experiences an initial acceleration $a_{0}$ towards the west. This indicates that there is an electric force acting on the proton in the westward direction.
- Force due to Electric Field:
$F_{e} = q E$
where $q$ is the charge of the proton and $E$ is the electric field. Since the proton is positively charged, the direction of the electric force is the same as the direction of the electric field.
- Acceleration:
$F = m a ⟹ q E = m a_{0} ⟹ E = \frac{m a_{0}}{q}$
Step 2: Analyze the second scenario (proton projected towards north)
In the second scenario, when the proton is projected towards the north with a speed $v_{0}$ , it experiences an initial acceleration of $3 a_{0}$ towards the west.
- Total Force: The total force acting on the proton is the sum of the electric force and the magnetic force. The magnetic force is given by:
$F_{m} = q (v \times B)$
Here, $v$ is the velocity of the proton (which is towards the north), and $B$ is the magnetic field.
- Direction of Forces:
Since the proton is moving north and the acceleration is towards the west, the magnetic force must also act towards the west to account for the increased acceleration.
Step 3: Set up the equations
From the second scenario, we can express the net force acting on the proton:
$F_{n e t} = F_{e} + F_{m}$
Given that $F_{e} = q E$ and $F_{m} = q (v_{0} \times B)$ , we can write:
$m a_{n e t} = q E + q (v_{0} \times B)$
Substituting the known values:
$m (3 a_{0}) = q E + q (v_{0} \times B)$
Step 4: Relate the two scenarios
From the first scenario, we know:
$E = \frac{m a_{0}}{q}$
Substituting this into the second scenario:
$m (3 a_{0}) = q (\frac{m a_{0}}{q}) + q (v_{0} \times B)$
This simplifies to:
$3 m a_{0} = m a_{0} + q (v_{0} \times B)$
Rearranging gives:
$2 m a_{0} = q (v_{0} \times B)$
Step 5: Solve for the magnetic field $B$
We can solve for $B$ :
$B = \frac{2 m a_{0}}{q v_{0}}$
Conclusion
The electric field $E$ is directed towards the west, and the magnetic field $B$ is perpendicular to the velocity of the proton (which is north). Thus, the electric field is responsible for the initial acceleration $a_{0}$ towards the west, and the magnetic field contributes to the increased acceleration $3 a_{0}$ when the proton is moving north.

|

Updated on:7/8/2024

Class 12 PHYSICS JEE MAIN REVISION TEST - 28

Similar Questions

Knowledge Check

Question 1 - Select One
When a proton is released from rest in a room, it starts with an initial acceleration $a_{0}$ towards west. When it is projected towards north with a speed $v_{0}$ it moves with an initial accelaration $3 a_{0}$ towards west. The electric and the maximum possible magnetic field in the room
(i) $\frac{m a_{0}}{e}$ , towards west
(ii) $\frac{2 m a_{0}}{e v_{0}}$ , downward
(iii) $\frac{m a_{0}}{e}$ , towards east
(iv) $\frac{2 m a_{0}}{e v_{0}}$ , upward
A(i), (ii)
B(ii), (iv)
C(ii), (iii)
D(i), (iv)
Question 2 - Select One or More
A particle is projected horizontally from a tower with velocity u towards east. Wind provides constant horizontal acceleration $\frac{g}{2}$ towards west. If particle strikes the ground vertically the then :

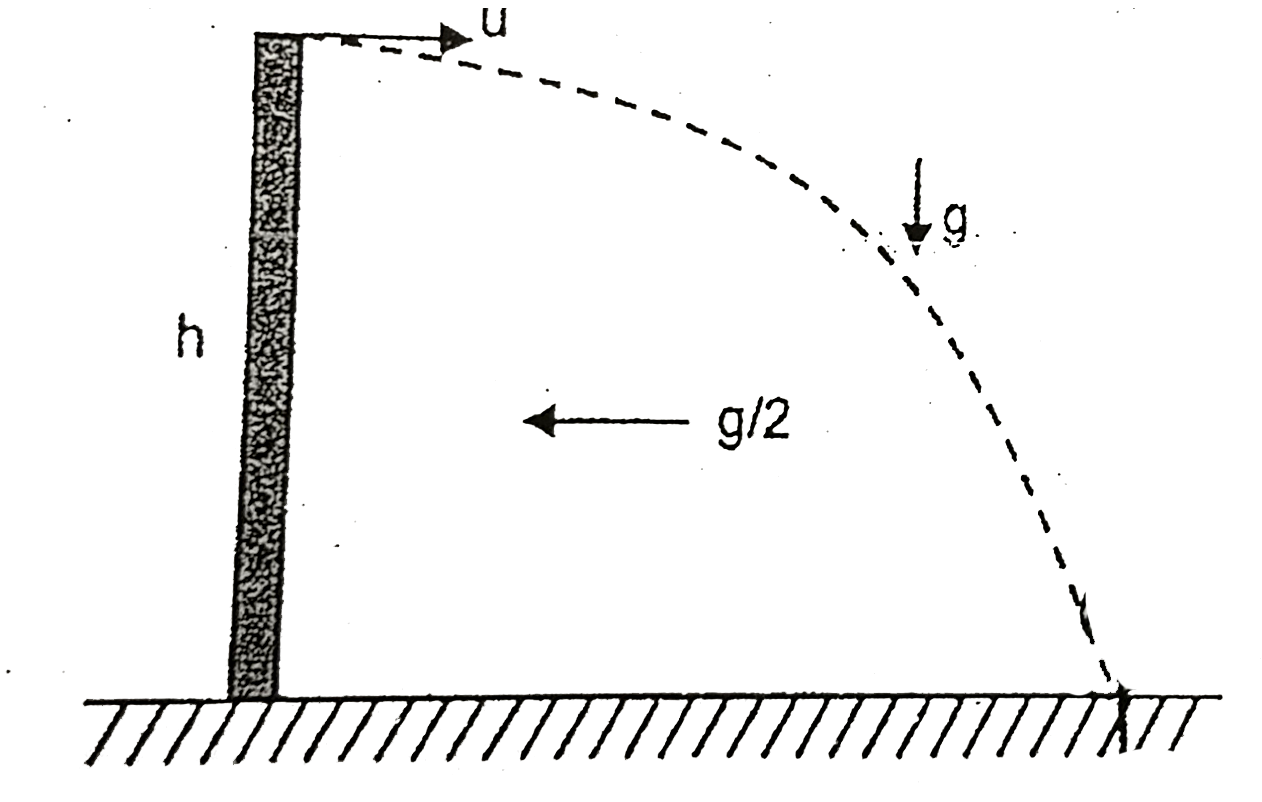AHeight of toward is $\frac{2 u^{2}}{g}$
BThe speed with which particle strikes ground is $2 u$
CWork done by wind force $\frac{1}{2} μ^{2}$
DParticle3 strikes the ground after time $t = 2 u / g$
Question 3 - Select One
A positively charged particle projected towards west is deflected towards north by a magnetic field. The direction of the magnetic field is
Atowards south
Btowards east
Cin downward direction
Din upward direction

Recommended Questions

Home
Ask Doubt
Profile