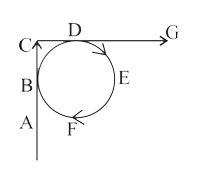
A very long wire ABCDEFBDG is shown in figure carrying current I. AC and CG parts are straight, long and at right angle. At D wire forms a circular turn DEFBD of radius R. AC, CG parts are tangential to circular turn at B and D. Magnetic field at the centre of circle is:
μ0IR
μ0I2πR(π+1)
μ0I2πR(π+1√2+1)
μ0I2πR(π−1√2)
The correct Answer is:C
We say we have 3 parts
B=BA+BB_+BC
=μ0I4πR(sin90∘+sin45∘)+μoI2R+μ0I4πR(sin45∘+sin90∘) =μ0I2πR(π+1√2+1)
Similar Questions
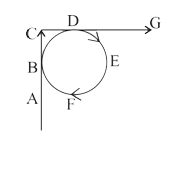
A very long wire ABDMNDC is shown in figure carrying current I. AB and BC parts are straight, long and at right angle. At D wire forms a circular turn DMND of radius R. AB, BC parts are tangential to circular turn at N and D. Magnetic field at the centre of circle is :
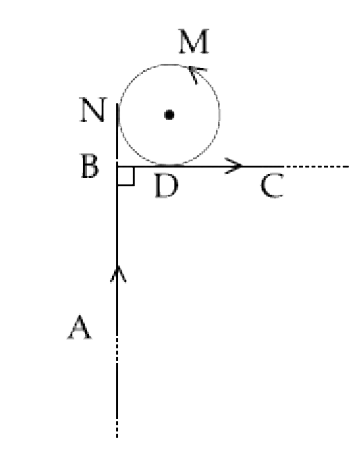
View SolutionIn the figure, the current l enters the circular loop of uniform wire of radius r at A and leaves it at B. The magnetic field of the centre of circular loop is
View Solution
Knowledge Check

A conducting wire carrying a current I is bent into the shape as shown. The net magnetic field at the centre O of the circular arc of radius RAμ0l2R(1+3ππ)Bμ0l4R(1+3ππ)Cμ0l8R(1+3ππ)Dμ0l8R(2+3ππ)A long wire bent as shown in fig. carries current I. If the radius of the semicircular portion is a, the magnetic field at centre C is
 Aμ0I4aBμ0I4πa√π2+4Cμ0I4a+μ0I4πaDμ0I4πa√(π2−4)
Aμ0I4aBμ0I4πa√π2+4Cμ0I4a+μ0I4πaDμ0I4πa√(π2−4)A circular coil of n turns and radius r carries a current I. The magnetic field at the center is
Aμ0nIrBμ0nI2rC2μ0nIrDμ0nI4r
A long wire having a semi-circular loop of radius r carries a current I, as shown in Fig. Find the magnetic field due to entire wire.

View SolutionTwo long thin wires ABC and DEF are arranged as shown in the figure. They carry currents I. The magnitude of the magnetic field at O is
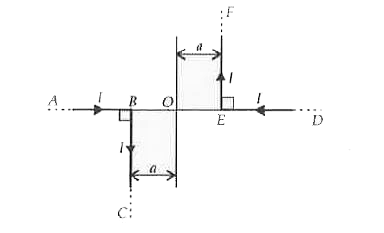
View SolutionA current carrying wire AB of the length L is turned along a circle, as shown in figure. The magnetic field at the centre O.

View SolutionA long straight conductor carrying a current i is bent to form an almost complete circular loop of radius r on it. The magnetic field at the centre of the loop
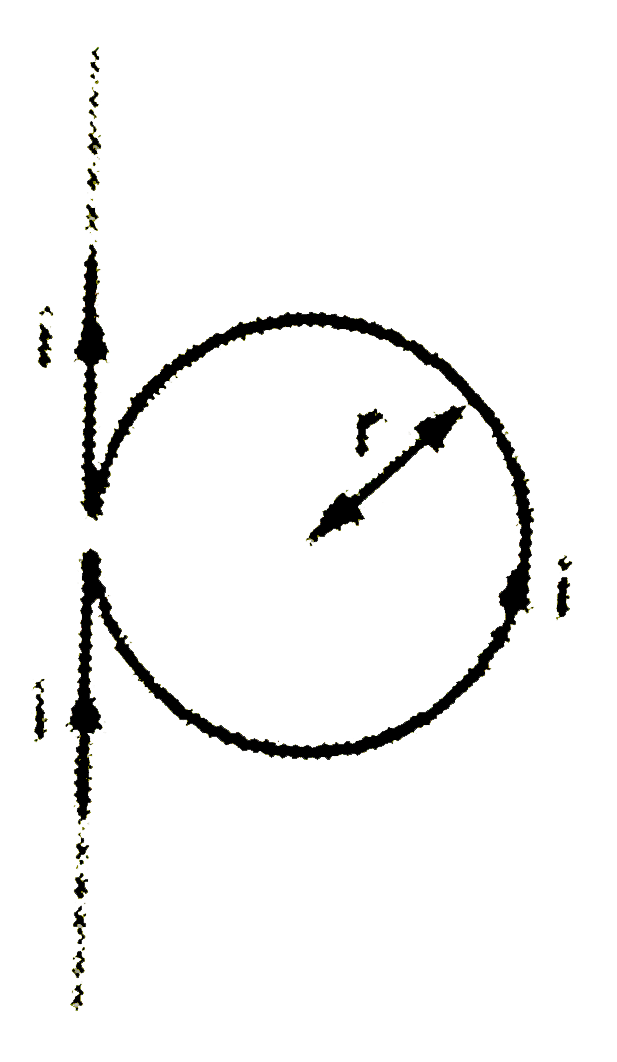
View SolutionA part of a long wire carrying a current i is bent into a circle of radius r as shown in figure. The net magnetic field at the centre O of the circular loop is

View Solution
