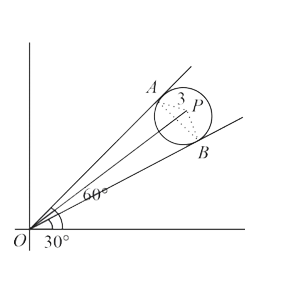
If a circle of radius $3$ units is touching the lines $\sqrt{3} y - 4 x y + \sqrt{3} x^{2} = 0$ in the first quadrant then the length of chord of contact to this circle, is:

$\frac{\sqrt{3} + 1}{2}$

$\frac{\sqrt{3} + 1}{\sqrt{2}}$

$3 (\frac{\sqrt{3} + 1}{\sqrt{2}})$

$\frac{3 (\sqrt{3} + 1)}{2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Updated on:21/07/2023

If a circle of radius 3 units is touching the lines $\sqrt{3} y^{2} - 4 x y + \sqrt{3} x^{2} = 0$ in the first quadrant then length of chord of contact to this circle is :
A $\frac{\sqrt{3} + 1}{2}$
B $\frac{\sqrt{3} + 1}{\sqrt{2}}$
C $3 (\frac{\sqrt{3} + 1}{\sqrt{2}})$
D $\frac{3 (\sqrt{3} + 1)}{2}$
If a circle of radius b units with centre at $(0, b)$ touches the line $y = x - \sqrt{2}$ , then what is the value of b ?
A $2 + \sqrt{2}$
B $2 - \sqrt{2}$
C $2 \sqrt{2}$
D $\sqrt{2}$
If a circle of radius b units with centre at $(0, b)$ touches the line $y = x - \sqrt{2}$ , then what is the value of b ?
A $2 + \sqrt{2}$
B $2 - \sqrt{2}$
C $2 \sqrt{2}$
D $\sqrt{2}$