A point P moves on line $2 x - 3 y + 4 = 0$ If $Q (1, 4)$ and $R (3, - 2)$ are fixed points, then the locus of the centroid of $△ P Q R$ is a line: (a) with slope $\frac{3}{2}$ (b) parallel to y-axis (c) with slope $\frac{2}{3}$ (d) parallel to x-axis

A

parallel to x-axis

B

with slope $\frac{3}{2}$

C

parallel to y-axis

D

with slope $\frac{2}{3}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

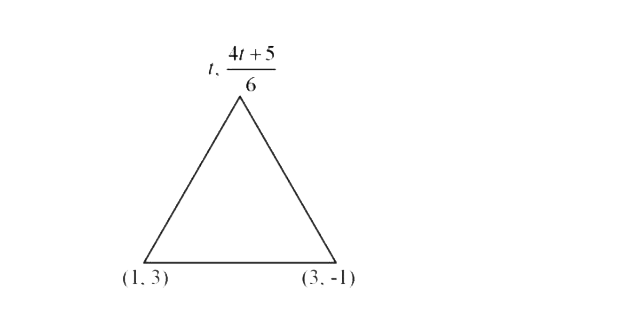
$4 x - 6 y + 5 = 0$
$Q (1, 3), P (3, - 1)$
Let (h,k) be centroid
$h = \frac{t + 1 + 3}{3} = \frac{t + 4}{3}$
$k = \frac{\frac{4 t + 5}{6} + 3 - 1}{3} = \frac{\frac{4 t + 5 + 12}{6}}{3} = \frac{4 t + 17}{18}$
$t = 3 h - 4$
$18 k - 17 = 4 t$
$18 k = 17 = 4 (3 h - 4)$
$18 k - 17 = 12 h - 16$
$12 h - 18 k + 1 = 0$
$12 x - 18 y + 1 = 0$
Slope $= (\frac{12}{18}) = \frac{2}{3}$

|

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Similar Questions

Knowledge Check

Question 1 - Select One
The vertex C of a triangle ABC moves on the line $L \equiv 3 x + 4 y + 5 = 0$ . The co-ordinates of the points A and B are $(2, 7)$ and $(5, 8)$ The locus of centroid of $Δ A B C$ is a line parallel to:
AAB
BBC
CCA
DL
Question 2 - Select One
The vertex C of a triangle ABC moves on the line $L \equiv 3 x + 4 y + 5 = 0$ . The co-ordinates of the points A and B are $(2, 7)$ and $(5, 8)$ The locus of centroid of $Δ A B C$ is a line parallel to:
AAB
BBC
CCA
DL
Question 3 - Select One
Let P is a point on the line $y + 2 x = 2$ and Q and R are two points on the line $3 y + 6 x = 3$ . If the triangle PQR is an equilateral triangle, then its area (in sq. units) is equal to
A $\frac{1}{15}$
B $\frac{1}{5 \sqrt{3}}$
C $\frac{1}{3 \sqrt{5}}$
D $\frac{1}{2 \sqrt{15}}$

Recommended Questions

Home
Ask Doubt
Profile