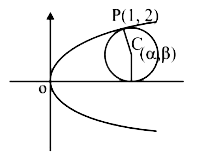
The area (in sq. units) of the smaller of the two circles that touch the parabola, y2=4x at the point (1, 2) and the x-axis is:
A
4π(2−√2)
B
8π(2−√2)
C
8π(3−2√2)
D
4π(3+√2)
Video Solution
Text Solution
The correct Answer is:C
y2=4x2yy'=4y'=2y∣−1=β−2α−1−α+1=β2β=3−α
Slope of normal =−22=−1
(α−1)2+(β−2)2=β2, α2+β2−2α=4β+5=β2
α2−2α−4β+5=0
α2−2α−4β+5=0
α2−2α−12+4α+5=0
α2+2α−7=0
(α+1)2=8, α+1=±2√2
α=−1−2√2,−1+2√2 ∴β=3−(−1+2√2)
Radius β=4−2√2
Area =π(β2)=π(4−2√2)2=4π(2−√2)2=4π(4+2−4√2)=8π(3−2√2)
|
Similar Questions
Knowledge Check
The area ( in sq, units ) of the part of the circle x2+y2=36 , which is outside the parabola y2=9x, is
A24π+3√3B12π−3√3C24π−3√3D12π+3√3The area (in sq units) in the first quadrant bounded by the parabola, y=x2+1, the tangent to it at the point (2, 5) and the coordinate axes is
A143B18724C83D3724The area (in sq. units) in the first quadrant bounded by the parabola y=x2+1, the tangent to it at the point (2, 5) and the coordinate axes is
A143B18724C3724D83
Radius of smaller circle that touches the line y=x at (1,1) and also touches the x-axis is:
View SolutionThe area (in sq units) of the circle which touches the line 4x+3y=15 and 4x+3y=15
View SolutionThe area (in sq. units) bounded by the parabola y=x2−1, the tangent at the point (2,3) to it and the y-axis is
View SolutionThe area (in sq. units) of the region in the first quadrant bounded by y=x2,y=2x+3 and the y - axis is
View SolutionThe area (in sq. units) of the region bounded by the X-axis and the curve y=1−x−6x2 is
View Solution