The sum of the squares of the length of the chords intercepted on the circle x2+y2=16, by the lines x +y = n , n∈N, where N is the set of all natural numbers, is
A
160
B
320
C
105
D
210
Video Solution
Text Solution
The correct Answer is:D
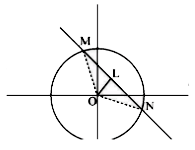
x+y=n will be intersecting
x2+y2=16 if n=1,2,3,4,5(n ∈N)
Length OL=n√2 (Distance of from origin)
OM=4 (radius)
MN=2√16−n22
(MN)2=4(16−n22)
5∑n=14(16−n22)=4[16×5−5×6×112×6]=320−110=210
|
Similar Questions
Knowledge Check
The mapping f : N→N given by f(n)=1+n2,n∈N, where N is the set of natural numbers, is
Aone-one and ontoBonto but not one-oneCone-one but not ontoDNeither one-one nor ontoThe mapping f : N→Ngiven by f(n)=1+n2,n∈N where, N is the set of natural numbers is
Aone-one and ontoBonto but not one -oneCone-one but not ontoDneither one-one nor ontoThe number of elements of the set {x:X∈N,x2=1} where N is the set of all natural numbers is :
A2B1C0D3
Find the length of the chord intercepted by the circle x2+y2−8x−2y−8=0 on the line x+y+1=0
View Solutionthe length of the chord intercepted by the circle x2+y2=r2 on the line xa+yb=1
View SolutionSum of square of m natural numbers
View SolutionSum of the square of the length of the chord intersected by the line x+y=n,n∈N on circle x2+y2=4 is: (A) 11 (B) 22 (C) 33 (D) none of these
View SolutionFind the middle point of the chord intercepted on line lx+my+n=0 by circle x2+y2=a2.
View Solution
