A rocket has to be launched from earh in such a way that it never returns. If E is the minimum energy delivered by the rocket launcher what should be the minimum energy that the launcher should have if the same rocket has launcher from the surface of the moon ? Assume that the density of the earth and the moob are equal and that the earth's volume is $64$ times the volume of the moon

$\frac{E}{64}$

$\frac{E}{32}$

$\frac{E}{16}$

$\frac{E}{4}$

Video Solution

Text Solution

Verified by Experts

$V_{E} = 64 V_{m} \Rightarrow R_{E} = 4 R_{M}$
$g_{E} = G . ρ \frac{4 π}{3} R_{E} \Rightarrow \frac{g_{M}}{g_{E}} = \frac{R_{M}}{R_{E}} \Rightarrow g_{M} = \frac{g_{E}}{4}$
$E \propto v_{e}^{2} \Rightarrow E \propto g R$
$\frac{E_{M}}{E_{E}} = \frac{g_{M}}{g_{E}} \frac{R_{M}}{R_{E}} \Rightarrow \frac{E_{M}}{E} = \frac{1}{4} \times \frac{1}{4} \Rightarrow E_{M} = \frac{E}{16}$

Updated on:21/07/2023

The escape velocity of a rocket launched from the surface of the earth
ADoes not depend on the mass of the rocket
BDoes not depend on the mass of the earth
CDepends on the mass of the planet towards which it is moving
DDepends on the mass of the rocket
The acceleration due to gravity on the moon is $\frac{1^{t h}}{6}$ that on the earth. If the earth and the moon are assumed to have the same density, then the radius of the moon is
A $6 R_{e}$
B $\frac{1}{6} R_{e}$
C $\frac{1}{3} R_{e}$
D $\frac{1}{4} R_{e}$
A rocket is launched upward from the earth surface whose velocity time graph shown in figure. Then maximum height attained by the rocket is :-

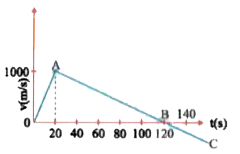A1 km
B10 km
C100 km
D70 km