Let I=∫ba(x4−2x2)dx. If I is minimum then the ordered pair (a,b) is (−√k,√l). The value of (k+l) is _________.
Video Solution
Text Solution
The correct Answer is:4
I=∫ba(x4−2x2)dx
Consider f(x)=x4−2x2=x2(x2−2)
Obviously ∫ba(x4−2x2)dx is least when a=−√2 and b=√2
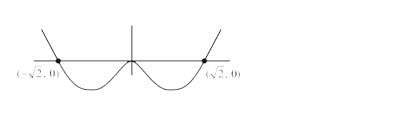
|
Similar Questions
Knowledge Check
Let I=∫31√x4+x2, dx then
AI>6√10BI<2√2C2√2<I<6√10DI<1If a<∫2π0)110+3cosxdx<b. Then the ordered pair (a,b) is
A(2π7,2π3)B(2π13,2π7)C(π10,2π13)Dnone of theseLet f(a,b)=∫ba(x2−4x+3)dx,(b>0) then
Af(a,3) is least when a=1Bf(a,b) is an increasing function ∀b≥4Cf(0,b) is least for b=2Dmin{f(a,b)}=−43