A rod, of length L at room tempera ture and uniform area of cross section A, is made of a metal having coefficient of linear expansion α/∘C. It is observed that an external compressive force F, is applied on each its ends, prevents any change in the length of the rod, when its temperature rises by ΔTK. Young's modulus, Y, for this metal is :
F2AαΔT
FAα(ΔT−273)
FAαΔT
2FAαΔT
The correct Answer is:C
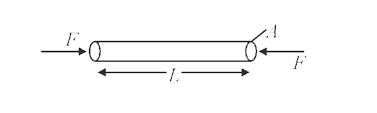
For no change in length ΔL+(−Δl)=0
Δl= increase in length due to change in temperature.
−Δl= decrease in length due to compressive stress.
⇒LαΔT=FLYA⇒Y=FAαΔT
Similar Questions
Knowledge Check
A metal rod of length 'L', cross-sectional area 'A', Young's modulus 'Y' and coefficient of linear expansion 'α' is heated to 't'∘C. The work that can be perfomred by the rod when heated is
AYAαLt22BYAα2Lt22CYAα2L2t22DYAαLT2A metal rod of length L cross sectional area A young modulus Y and coefficient of linear expansion α is heated to t∘C The work that can be performed by the rod when heated is
AYaαLt22BYaα2Lt22CYaα2L2t22DYaαLt2A rod of length l and coefficient of linear expansion α. Find increase in length when temperature changes from T to T+ ΔT
AlαΔTBl(1+αΔT)Cl(1+2αΔT)Dnone of these
The density of a uniform rod with cross-section A is d, its specific heat capacity is c and the coefficient of its linear expansion is α. Calculate the amount of heat that should be added in order to increase the length of the rod by l.
View SolutionA metal rod of length 'L', cross-sectional area 'A', Young's modulus 'Y' and coefficient of linear expansion 'α' is heated to 't'∘C. The work that can be perfomred by the rod when heated is
View SolutionA metallic rod of length 'L' and cross-section 'A' has Young's modulus 'Y' and coefficent of linear expansion 'α'. If the rod is heated to a temperature. 'T' the energy stored per unit volume is:
View SolutionA metal rof having coefficient of linear expansion α and Young's modulus Y is heated to raise its temperature by Δθ. The stress exerted by the rod is
View Solution
The variation of length of two metal rods A and B with change in temperature is shown in Fig. the coefficient of linear expansion αA for the metal A and the temperature T will be
View Solution
