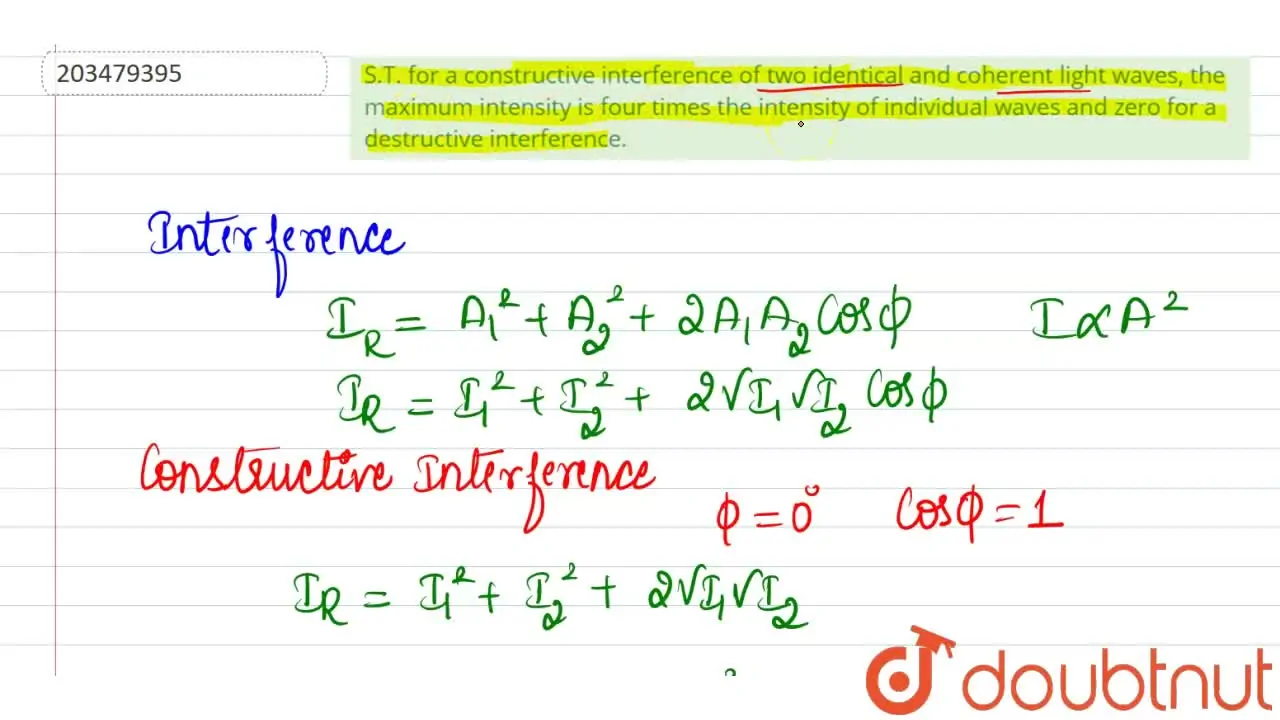
S.T. for a constructive interference of two identical and coherent light waves, the maximum intensity is four times the intensity of individual waves and zero for a destructive interference.Solution in Kannada

Video Solution

Text Solution

Verified by Experts

For an interference of two identical waves
$R = \sqrt{{(A)}^{2} + {(A)}^{2} + 2 (A) (A) cos (ϕ_{1} - ϕ_{2})}$
i.e., $R = \sqrt{2 A^{2} (1 + cos (ϕ_{1} - ϕ_{2}))}$
or $R = \sqrt{2} A (\sqrt{1 + cos ϕ})$ where $ϕ_{1} = ϕ_{1} - ϕ_{2}$
or $R = \sqrt{2} (A \sqrt{2 {cos}^{2} (\frac{ϕ}{2})})$
or $R = 2 A \frac{cos (ϕ)}{2}$
so that intensity $I = 4 A^{2} {cos}^{2} . \frac{ϕ}{2}$
For $C . I . {cos}^{2} . \frac{ϕ}{2} = 1 \Rightarrow \frac{ϕ}{2} = 0, \pm π, \pm 2 π, ... ... ... ... ... ... . . \pm n π$
or $ϕ = 0, \pm 2 π, \pm 4 π, ... ... ... ... ... ... ., \pm 2 n π$ , where $n = 0, 1, 2, 3$ .
i.e., $I_{max} = 4 A_{2} = 4 I_{0}$ where
$I_{0}$ is the maximum intensity of individual waves.
For destructive interference $I = 0 \Rightarrow {cos}^{2} . \frac{ϕ}{2} = 0$
i.e.., $ϕ = \pm 1 π, \pm 3 π, \pm 5 π, ... ... ... ... ... ... \pm (12 n + 1) π,$
where, $n = 0, 1, 2 .$
Note : if the phase difference b/w the two waves is not constant then the average intensity $= 4 I_{0} < {cos}^{2} . \frac{ϕ}{2} >$
i.e., $< I > = 2 I_{0}$ at all points.

Updated on:21/07/2023

|

Class 12 PHYSICS WAVE OPTICS

Topper's Solved these Questions

Similar Questions

SUBHASH PUBLICATION-WAVE OPTICS-FIVE MARKS QUESTIONS WITH ANSWERS

Home
Ask Doubt
Profile