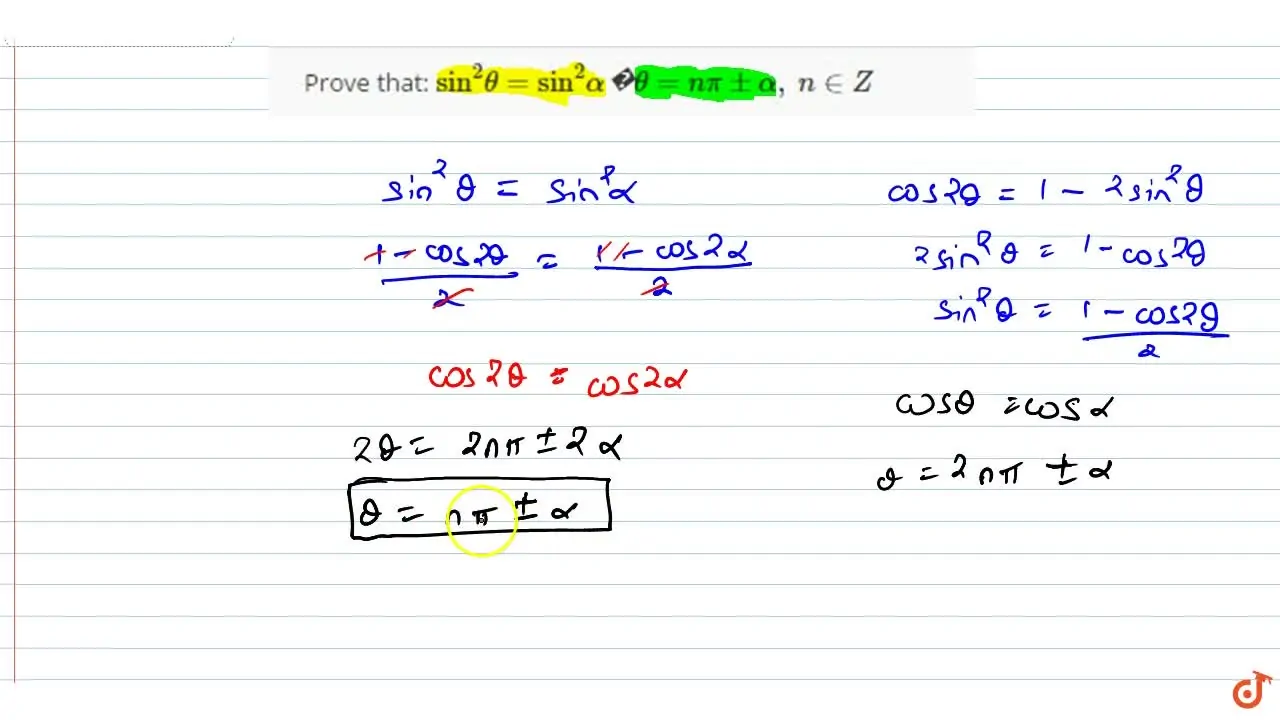
Prove that: ${sin}^{2} θ = {sin}^{2} α,$ then $θ = n π \pm α, n \in Z$

Video Solution

Text Solution

Verified by Experts

Given:
${sin}^{2} θ = {sin}^{2} α$
Using Identity
$cos 2 θ = 1 - 2 {sin}^{2} θ$
$2 {sin}^{2} θ = 1 - cos 2 θ$
${sin}^{2} θ = \frac{1 - cos 2 θ}{2}$
$\frac{1 - cos 2 θ}{2} = \frac{1 - cos 2 α}{2}$
$cos 2 θ = cos 2 α$
$2 θ = 2 n π \pm 2 α$
$θ = n π \pm α$

|

Updated on:21/07/2023

Class 11 MATHS TRIGONOMETRIC EQUATIONS

Topper's Solved these Questions

BOOK - RD SHARMACHAPTER - TRANSFORMATION FORMULAEEXERCISE - Solved Examples And Exercises
145videos
TRANSFORMATION FORMULAE
BOOK - RD SHARMACHAPTER - TRIGONOMETRIC FUNCTIONSEXERCISE - Solved Examples And Exercises
167videos
TRIGONOMETRIC FUNCTIONS

Similar Questions

Knowledge Check

Question 1 - Select One
A line makes the same angle $α$ with each of the x and y axes. If the angle $θ$ , which it makes with the z-axis, is such that ${sin}^{2} θ = 2 {sin}^{2} α$ , then what is the value of $α$ ?
A $π / 4$
B $π / 6$
C $π / 3$
D $π / 2$
Question 2 - Select One
If $∣ ∣ cos θ {sin θ + \sqrt{{sin}^{2} θ + {sin}^{2} α}} ∣ ∣ \leq k$ , then the value of k
A $\sqrt{1 + {cos}^{2} α}$
B $\sqrt{1 + {sin}^{2} α}$
C $\sqrt{2 + {sin}^{2} α}$
D $\sqrt{2 + {cos}^{2} α}$

RD SHARMA-TRIGONOMETRIC EQUATIONS-Solved Examples And Exercises

Home
Ask Doubt
Profile