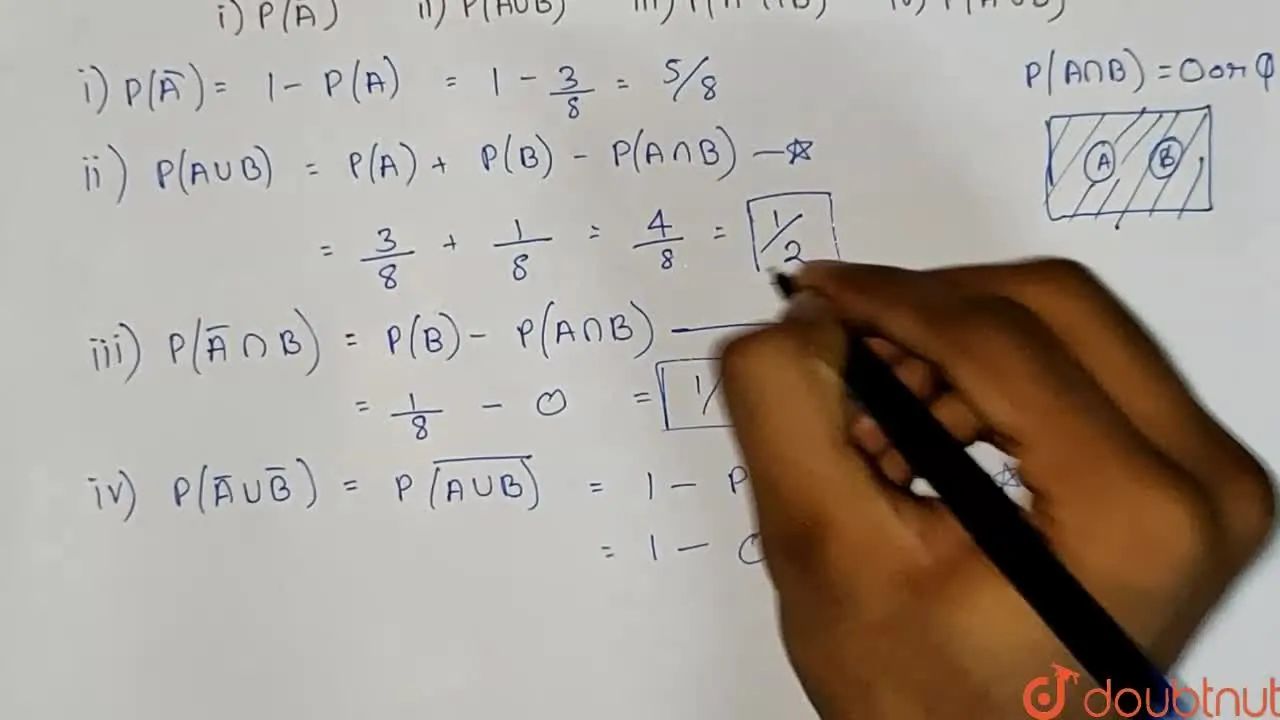If A and B are mutually exclusive events P(A)=38andP(B)=18, then find
(i)P(¯¯¯A) (ii)P(A∪B) (iii)P(¯¯¯A∩B) (iv)P(¯¯¯A∪¯¯¯B)Solution in Tamil
Step by step video & image solution for If A and B are mutually exclusive events P(A) = 3/8 and P(B) = 1/8, then find ("i") P(barA)" "("ii") P(A uu B)" "("iii")P(barA nn B)" "("iv")P(barA uu barB) by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
- BOOK - SURA PUBLICATIONCHAPTER - INTRODUCTION OF PROBABILITY THEOREMEXERCISE - EXERCISE 12.312videos
INTRODUCTION OF PROBABILITY THEOREM
- BOOK - SURA PUBLICATIONCHAPTER - INTRODUCTION OF PROBABILITY THEOREMEXERCISE - EXERCISE 12.45videos
INTRODUCTION OF PROBABILITY THEOREM
- BOOK - SURA PUBLICATIONCHAPTER - INTRODUCTION OF PROBABILITY THEOREMEXERCISE - ADDITIONAL PROBLEMS SECTION - A14videos
INTRODUCTION OF PROBABILITY THEOREM
- BOOK - SURA PUBLICATIONCHAPTER - INTEGRAL CALCULUSEXERCISE - Section - D (5 marks)3videos
INTEGRAL CALCULUS
- BOOK - SURA PUBLICATIONCHAPTER - MATRICES AND DETERMINANTS EXERCISE - ADDITIONAL PROBLEMS SECTION -D (5 MARK )3videos
MATRICES AND DETERMINANTS
Similar Questions
Can two events be mutually exclusive and independent simultaneously ?
02:07View SolutionA factory has two Machines - I and II. Machine-I produced 60% if items and Machine - II produces 40% of the items of the total output. Further 2% of the items produced by Machine - I are defective whereas 4% produced by Machine - II are defective. If an item is drawn at random what is the probability that it is defective ?
02:01View SolutionA and B are two events such that P(A) = 0.25 P(B) = 0.50. The probability of both happening together is 0.14. The probability of both A and B not happening is
03:36View SolutionEvaluate ∫dxtanx+cotx+secx+cosecx
01:52View SolutionUsing factor theorem, show that ∣∣ ∣∣−2aa+bc+aa+b−2ab+cc+ac+b−2c∣∣ ∣∣=4(a+b)(b+c)(c+a)
02:31View SolutionFor two events A and B P(A)=0.7,(B)=0.5 and P(A∩B)=0.3. Find the values of following:
(i) P(A∪B) (ii) P(A/B)
(iii) P(¯¯¯A/¯¯¯B) (iv) P(¯¯¯B/¯¯¯A)02:21View SolutionThe probability of an event A occurring is 0.5 and B occurring is 0.3. If A and B are mutually exclusive events, then find the probability of
(i) P(A∪B) (ii) P(A∩¯¯¯B) (iii) P(¯¯¯A∩B).02:09View SolutionIf P(A∪B)=0.8andP(A∩B)=0.3 then P(¯¯¯A)+P(¯¯¯B)=
01:45View SolutionIf A and B are mutually exclusive events P(A)=38andP(B)=18, then find
(i) P(¯¯¯A) (ii) P(A∪B) (iii) P(¯¯¯A∩B)03:03View SolutionIf A and B are events of a random experiment such that
P(A∪B)=45,P(¯¯¯A∪¯¯¯B)=P(¯¯¯A∩¯¯¯B)710 and P(B)=25 , then P(A) equal07:21View SolutionIf ¯¯¯Aand¯¯¯B are the events complementary to the events A and B respectively, prove that,
P(¯¯¯Aor¯¯¯B)=1−P(A)P(B/A).02:36View SolutionIf A and B are two events such that P(A∪B)=0.65,P(A∩B)=0.15 then P(¯¯¯A)+P(¯¯¯B)=
02:03View SolutionIf A, B are two mutually exclusive events such that P(A)=0.5 ,P(B)=0.3 then P(¯¯¯A∩¯¯¯B)=
View SolutionGiven P(A)=0.5P(B)=0.6andP(A∩B)=0.24. Find (i)P(A∪B)(ii)P(¯¯¯A∩B).(iii)P(A∩¯¯¯B),(iv)P(¯¯¯A∪¯¯¯B),(v)P(¯¯¯A∩¯¯¯B)
01:21View SolutionP(A)=0.3P(B)=0.6andP(A∩B)=0.25. Find (i)n P(A∪B),(ii)P(A/B),(iii)P(B/¯¯¯A),(iv)P(¯¯¯A/B),(v)P(¯¯¯A/¯¯¯B)
02:24View Solution