Question

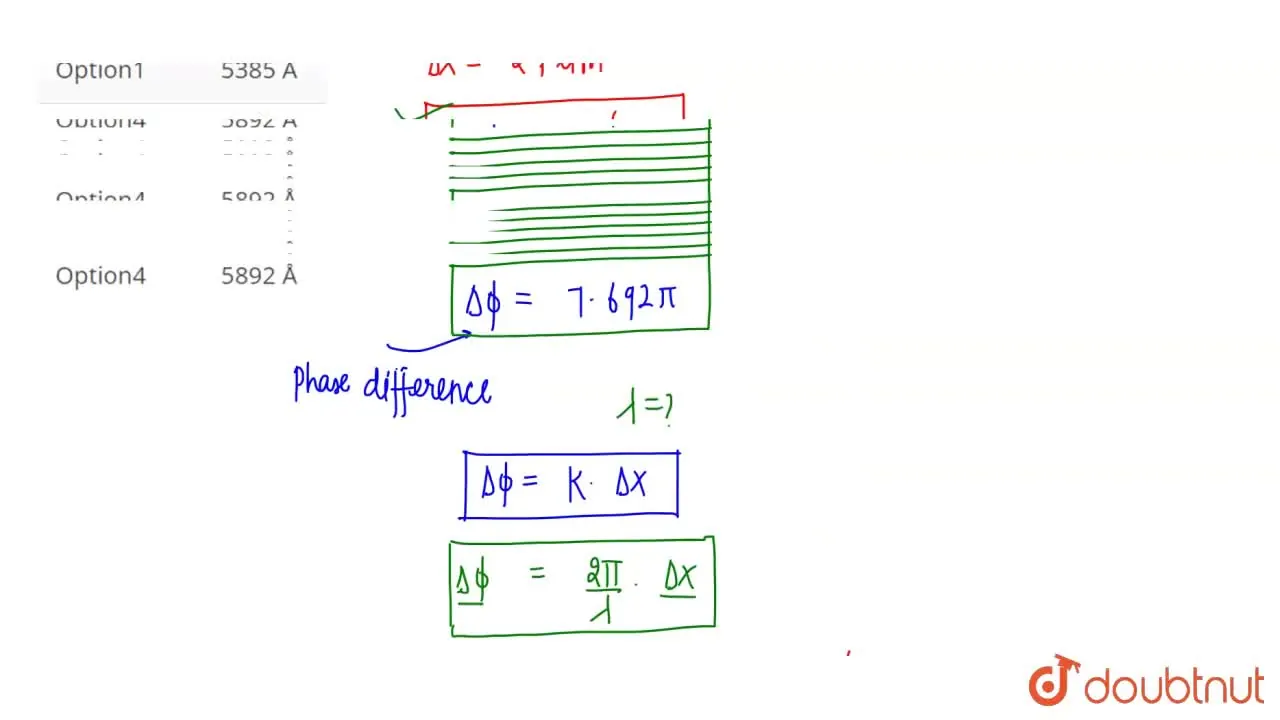
Path difference between two wavefronts emitted from coherent sources is $2.1\mu m$. Phase difference between the wavefronts at that point is 7.692 $\pi$the wavelength of light emitted by source will be

Options

A. 5385 Å
B. 5600 Å
C. 5460Å
D. 5892 Å

Answer

C

Explanation

To find the wavelength of light emitted by the source, we can use the relationship between path difference and phase difference. Here are the steps to solve the problem:

Step 1: Understand the given values
- Path difference (\( \Delta x \)) = 2.1 µm = \( 2.1 \times 10^{-6} \) m
- Phase difference (\( \Delta \phi \)) = \( 7.692 \pi \)

Step 2: Use the relationship between phase difference and path difference
The relationship between phase difference and path difference is given by the formula:
\( \Delta \phi = \frac{2\pi}{\lambda} \Delta x \)
where:
- \( \Delta \phi \) is the phase difference,
- \( \Delta x \) is the path difference,
- \( \lambda \) is the wavelength.

Step 3: Substitute the known values into the equation
Substituting the known values into the equation:
\( 7.692 \pi = \frac{2\pi}{\lambda} (2.1 \times 10^{-6}) \)

Step 4: Simplify the equation
We can cancel \( \pi \) from both sides:
\( 7.692 = \frac{2}{\lambda} (2.1 \times 10^{-6}) \)

Step 5: Rearrange to solve for \( \lambda \)
Rearranging the equation to solve for \( \lambda \):
\( \lambda = \frac{2 \times (2.1 \times 10^{-6})}{7.692} \)

Step 6: Calculate the wavelength
Now, calculate the value:
\( \lambda = \frac{4.2 \times 10^{-6}}{7.692} \)
Calculating this gives:
\( \lambda \approx 5.46 \times 10^{-7} \text{ m} = 546 \text{ nm} \)

Final Answer
The wavelength of the light emitted by the source is approximately 546 nm.

---