The scores given below were earned by 10 students on a recent biological test. What is the median score?
71, 94, 86, 77, 88, 94, 88, 80, 78,94
85
86
87
88
The correct Answer is:D
To find the median score from the given data of 10 students, we will follow these steps:
Step 1: Arrange the data in ascending order
The scores given are:
71, 94, 86, 77, 88, 94, 88, 80, 78, 94
Arranging these scores in ascending order gives us:
71, 77, 78, 80, 86, 88, 88, 94, 94, 94
Step 2: Determine the number of data points
We have a total of 10 scores (n = 10), which is an even number.
Step 3: Identify the median position
For an even number of data points, the median is calculated as the average of the two middle numbers. The positions of these middle numbers can be found using the formula:
- n/2 = 10/2 = 5 (5th term)
- n/2 + 1 = 10/2 + 1 = 6 (6th term)
Step 4: Find the 5th and 6th terms in the ordered list
From our ordered list:
- The 5th term is 86
- The 6th term is 88
Step 5: Calculate the median
Now, we find the average of the 5th and 6th terms:
Median = (5th term + 6th term) / 2
Median = (86 + 88) / 2
Median = 174 / 2
Median = 87
Final Answer
The median score of the given data is 87.
---
The scores given are:
71, 94, 86, 77, 88, 94, 88, 80, 78, 94
71, 77, 78, 80, 86, 88, 88, 94, 94, 94
We have a total of 10 scores (n = 10), which is an even number.
For an even number of data points, the median is calculated as the average of the two middle numbers. The positions of these middle numbers can be found using the formula:
- n/2 = 10/2 = 5 (5th term)
- n/2 + 1 = 10/2 + 1 = 6 (6th term)
From our ordered list:
- The 5th term is 86
- The 6th term is 88
Now, we find the average of the 5th and 6th terms:
Median = (5th term + 6th term) / 2
Median = (86 + 88) / 2
Median = 174 / 2
Median = 87
The median score of the given data is 87.
Topper's Solved these Questions
Similar Questions
The ages of ten students of a group are given below: The ages have been recorded in years and months: 8-6,9-0,8-4,9-3,7-8,8-11,8-7,9-2,7-10,8-8 What is the lowest age? What is the highest age? Determine the range?
View SolutionThe marks of 10 students of a class in an examination arranged in ascending order are as follows :
13, 35, 43, 46, x, x + 4, 55, 61, 71, 80
If the median marks is 48, find the value of x. Hence find the mode of the given data.
View Solution
Knowledge Check
The marks scored by 10 students in a monthly test are:
9, 13, 17, 6, 8, 13, 11, 10, 5, 9
The median marks areA9B10C9.5D10.5In a particular college, the test scores of the most recent test given for a particular Physics class and a particular Literature class were studied. Both tests were scored from 0 to 100 and had a total of 20 question, which were equally weighted with no partial credit. The physics class had 128 students and the Literature class had 75 students . The test results are shown in the two graphs below.
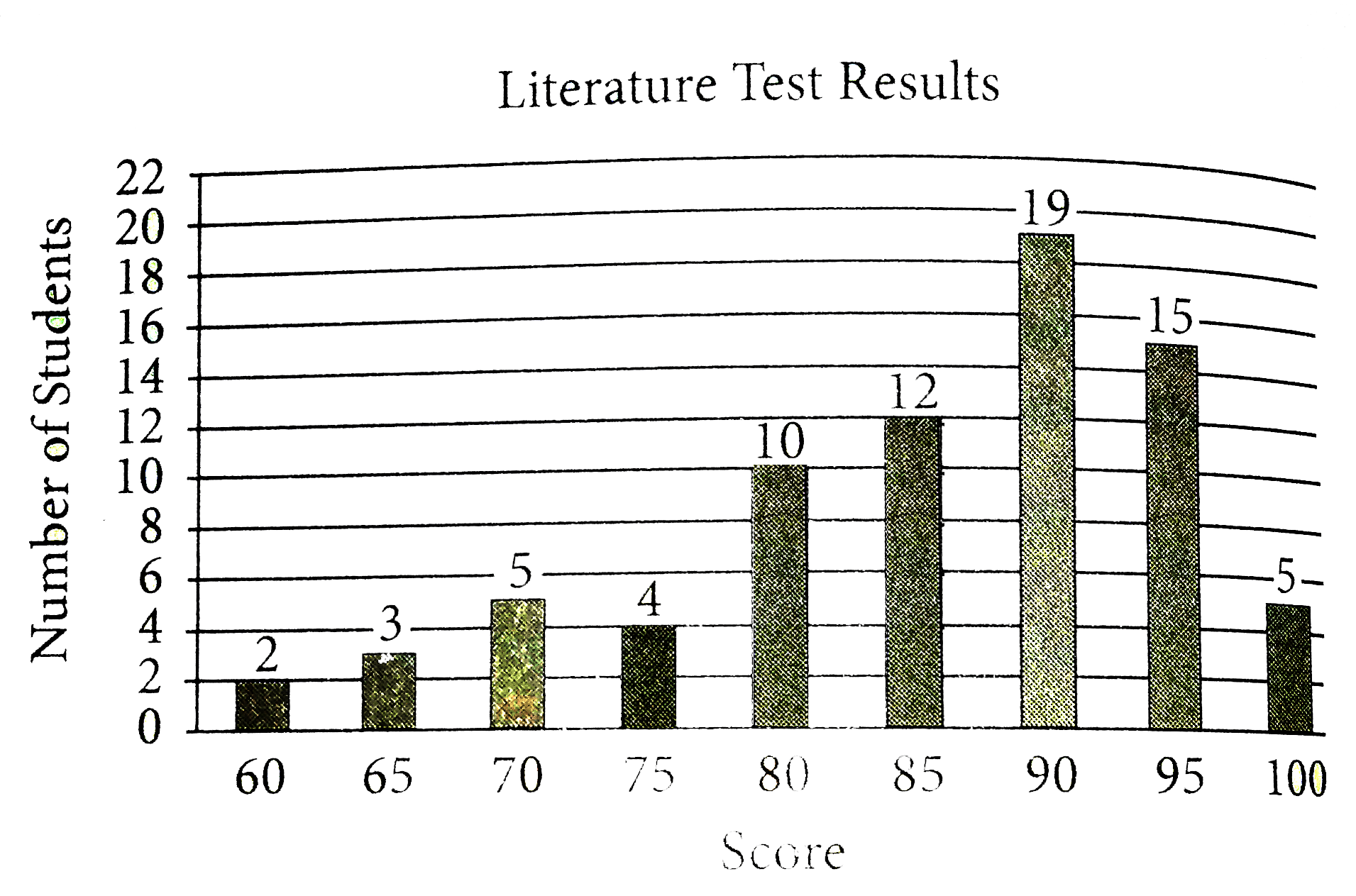
The dean of the college is comparing the scores from the two classes and calculates the median for each class. If the dean labels the median score of the Physics class P and the median score of the Literature class L, what is the sum of P and L?A175B170C85D80
At a shooting competition the scores of a competitor were as given below: (i) What was his modal score?
(ii) What was his median score?
(iii) What was his total score? (iv) What was his mean score?
View SolutionThe scores of 48 children in an intelligence test are shown in the following frequency table .
Score 7176798386899297101103107110114Frequency 4345654433322
Calculate σ2 and find the percentage of students whose score lie between ¯x−σand¯x+σ
View SolutionFind the mean of the marks obtained by 30 students of Class IX of aschool, given in Example 2.10 20 36 92 95 40 50 56 60 7092 88 80 70 72 70 36 40 36 40 92 40 50 50 56 60 70 60 60 88
View SolutionThe marks scored by 40 students of class VIII in mathematics are given below: 81,55,68,79,85,43,29,68,54,73,47,35,72,64,95,44,50,77,64, 35,79,52,45,54,70,83,62,64,72,92,84,76,63,43,54,38,73,68,52,54. Prepare a frequency distribution with class size of 10 marks.
View SolutionMarks scored by 100 students in a 25 marks unit test of Mathematics is given below. Their median is
Marks 0−55−1010−1515−2020−25Students 10 18 42 23 7
View SolutionThe scores in mathematics test (out of 25) of 15 students is as follows:19, 25, 23, 20, 9, 20, 15, 10, 5, 16, 25, 20, 24, 12, 20Find the mode and median of this data. Are they same?
View Solution
