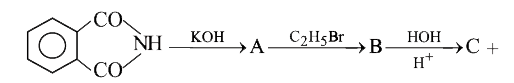In the sequence x,x+d, x+2d,x+3d , assume that x and d are positive integers . What is the difference between the arithmetic mean and the median of the numbers in the sequence ?
2x + 3d
2x+3d2
x+d2
0
The correct Answer is:D
To solve the problem, we need to find the difference between the arithmetic mean and the median of the sequence x,x+d,x+2d,x+3d.
Step 1: Identify the terms of the sequence
The sequence consists of four terms:
1. x
2. x+d
3. x+2d
4. x+3d
Step 2: Calculate the Arithmetic Mean
The arithmetic mean (AM) is calculated by taking the sum of all terms and dividing by the number of terms.
AM=x+(x+d)+(x+2d)+(x+3d)4
Calculating the sum:
x+(x+d)+(x+2d)+(x+3d)=4x+(0+d+2d+3d)=4x+6d
Now, divide by the number of terms (which is 4):
AM=4x+6d4=x+3d2
Step 3: Calculate the Median
Since there are four terms (an even number), the median is the average of the two middle terms. The middle terms in the sequence are x+d and x+2d.
Calculating the median:
Median=(x+d)+(x+2d)2=2x+3d2=x+3d2
Step 4: Find the Difference Between the Arithmetic Mean and the Median
Now, we need to find the difference between the arithmetic mean and the median:
Difference=AM−Median
Substituting the values we found:
Difference=(x+3d2)−(x+3d2)=0
Final Answer
The difference between the arithmetic mean and the median of the numbers in the sequence is 0.
---
The sequence consists of four terms:
1. x
2. x+d
3. x+2d
4. x+3d
The arithmetic mean (AM) is calculated by taking the sum of all terms and dividing by the number of terms.
Since there are four terms (an even number), the median is the average of the two middle terms. The middle terms in the sequence are x+d and x+2d.
Now, we need to find the difference between the arithmetic mean and the median:
The difference between the arithmetic mean and the median of the numbers in the sequence is 0.
Similar Questions
Knowledge Check
- Question 1 - Select One
A finite arithmetic sequence has 7 terms, and the first term is 34. What is the difference between the mean and the median of the 7 terms?
A0B34C43D3 - Question 2 - Select One
Five numbers are given by expressions x, 2x−3,2x+1,3x−4and3x+1. If the average (arithmetic mean) of these number is 10, what is the mode of the numbers?
A10B11C12DThere is not enough information to determine the value of the mode.
If x is a positive integer such that the distance between points P(x, 2) and Q(3, −6) is 10 units, then x= (a) 3 (b) −3 (c) 9 (d) −9
View SolutionIf d is the minimum distance between the curves f(x)=exandg(x)=(log)ex, then the value of d6 is
View SolutionIf d is the minimum distance between the curves f(x)=exandg(x)=(log)ex, then the value of d6 is
View SolutionThe coefficient of the quadratic equation ax2+(a+d)x+(a+2d)=0 are consecutive terms of a positively valued, increasing arithmetic sequence. Then the least integral value of d/a such that the equation has real solutions is __________.
View SolutionIf D is the set of all real x such that 1−e1x−1 is positive , then D is equal to
View SolutionDifferentiate x2 with respect to x3 .
View Solution