A wire of length l is bent in the form a circular coil of some turns. A current I flows through the coil. The coil is placed in a uniform magnetic field B. The maximum torqur on the coil can be

A

$l B l^{2}$

B

$4 π l B l^{2}$

C

$\frac{l l^{2} B}{4 π}$

D

zero

Video Solution

Text Solution

Verified by Experts

The correct Answer is:c

$τ = N I A B = N π r^{2} B$
But, $N \times 2 π r = I$
$∴ r = \frac{I}{2 π N}$
$∴ R a u = N I π {(\frac{I}{2 π N})}^{2} B = N I π \frac{I^{2}}{4 π^{2} N^{2}} B = \frac{I I^{2} B}{4 π N}$
$∴ τ_{max} = \frac{I I^{2} B}{4 π}$ $[N_{min} = 1]$

|

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A constant current $I$ is flowing through a circular coil placed in uniform magnetic field $\to B$ as shown. Then:

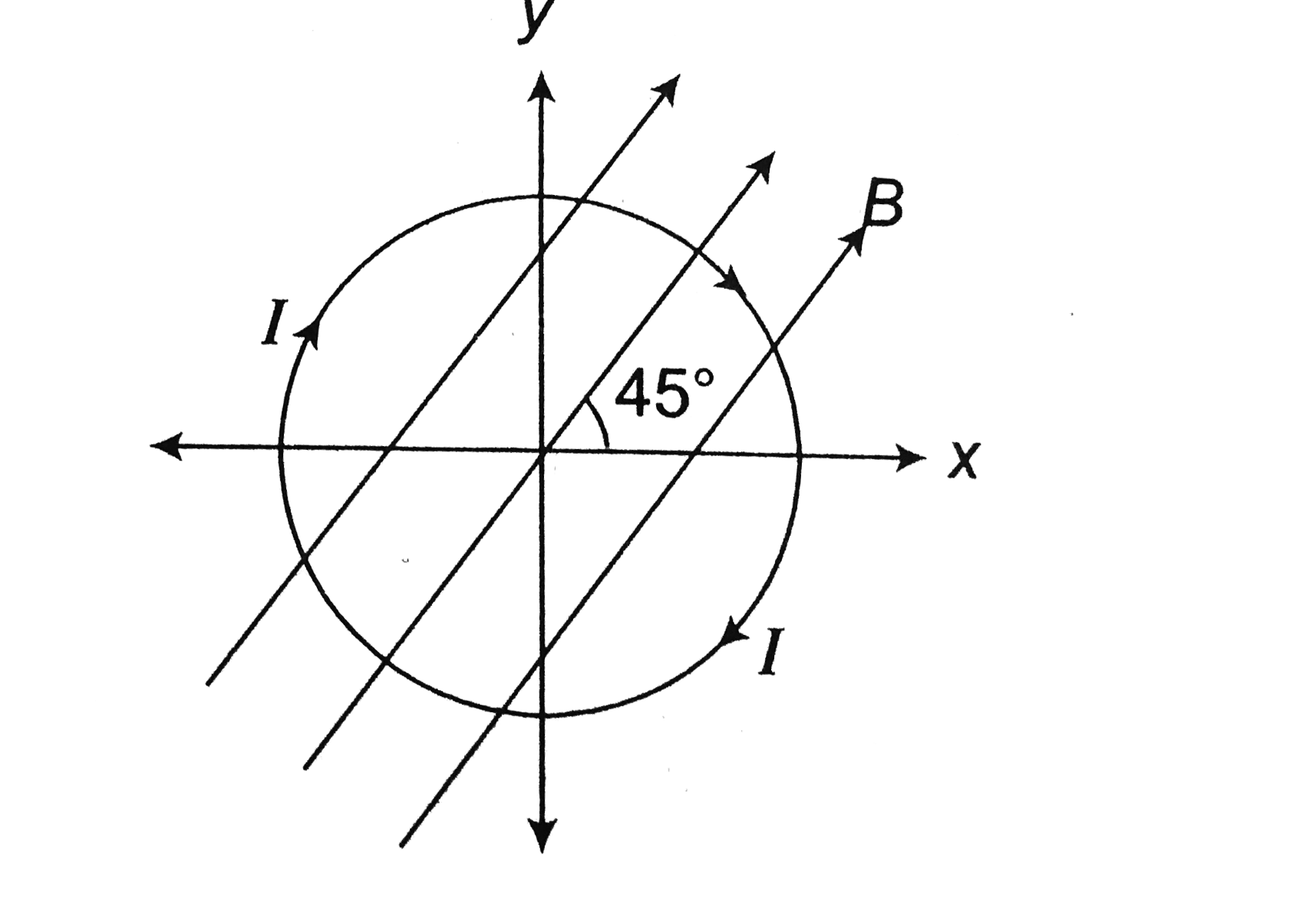AThe loop is in stable equilibrium
BThe loop is in unstable equilibrium
CThe torque acting on the loop is maximum
DThe torque acting on the loop is $\frac{1}{\sqrt{2}}$ times the maximum torque
Question 2 - Select One
A wire of length L is bent to form a circular loop of number of turns n. The coil is placed in a magnetic field of induction B and a current is passed through the coil. What will be the maximum torque acting on the coil?
A $I b l^{2}$
B $z e r o$
C $\frac{I b l^{2}}{4 π n}$
D $\frac{4 π n^{2}}{I b l^{2}}$
Question 3 - Select One
A wire length $6.28 m$ is bent into a circular coil of 2 turns. If a current of $0.5 A$ exists in the coil, the magnetic moment of the coil is, in $A m^{2} :$
A $\frac{π}{4}$
B $\frac{1}{4}$
C $π$
D $4 π$

BITSAT GUIDE-MAGNETIC FIELD-All Questions

Home
Ask Doubt
Profile