If the displacement (x) and velocity (v) of a particle executing SHM are related through the expression 3v2=30−x2. If the time period of the particle is T=π√n, then what is the value of n?
The correct Answer is:12.00
Step by step video, text & image solution for If the displacement (x) and velocity (v) of a particle executing SHM are related through the expression 3v^(2)=30-x^(2). If the time period of the particle is T=pisqrt(n), then what is the value of n? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
The plot of velocity (v) versus displacement (x) of a particle executing simple harmonic motion is shown in figure. The time period of oscillation of particle is :-

View SolutionIf the displacement (y in m) and velocity (v in ms−1) of a particle executing SHM are related by the equation 4v2=16−y2, then the path length of the motion and time period of oscillation respectively are
View Solution
Knowledge Check
If the displacement (x) and velocity (v) of a particle executing simple harmonic motion are related through the expression 4v2=25−x2, then its time period is given by
AπB2πC4πD6πDispalcement (x) and velocity (v) of a particle exectuting S.H.M are related as 2v2=9−x2. The time period of particle is
A4πB2πC4√2πD2√2πIf the displacement x and velocity v of a aprticle executing SHM are related as 4v2=25−x2. Then its maximum displacement in metre (x,v are in SI) is
A1B2C5D6
The equation of motion of a particle executing SHM is (d2xdt2)+kx=0. The time period of the particle will be :
View SolutionThe displacement (x) is plotted with time (t) for a particle executing SHM as shown below. The correct equation of SHM is
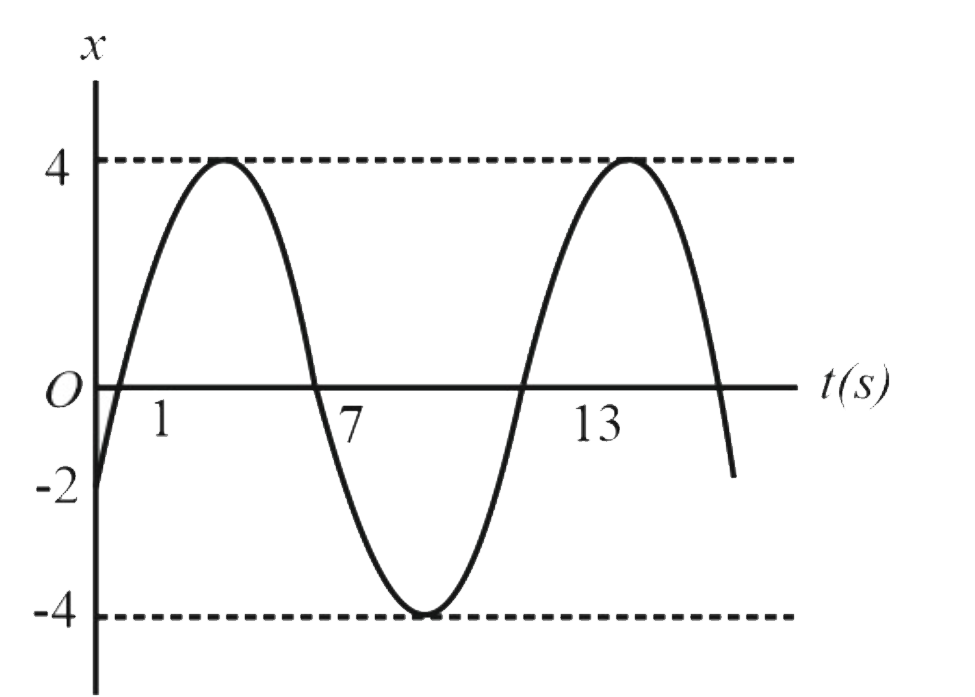
View SolutionIf the maximum velocity and acceleration of a particle executing SHM are equal in magnitude the time period will be
View SolutionIf the maximum velocity and acceleration of a particle executing SHM are equal in magnitude, the time period will be
View SolutionThe disperod of a particle varies according to the relation x=4(cosπt+sinπt). The amplitude of the particle is.
View Solution
