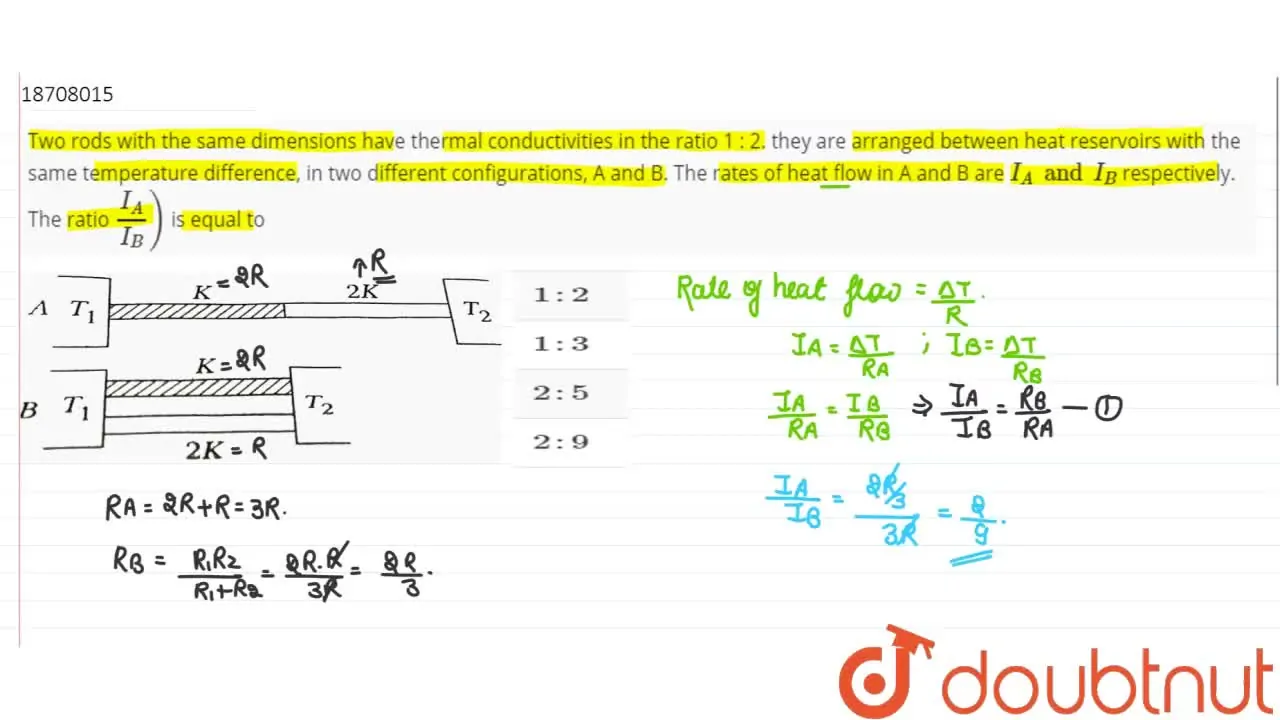
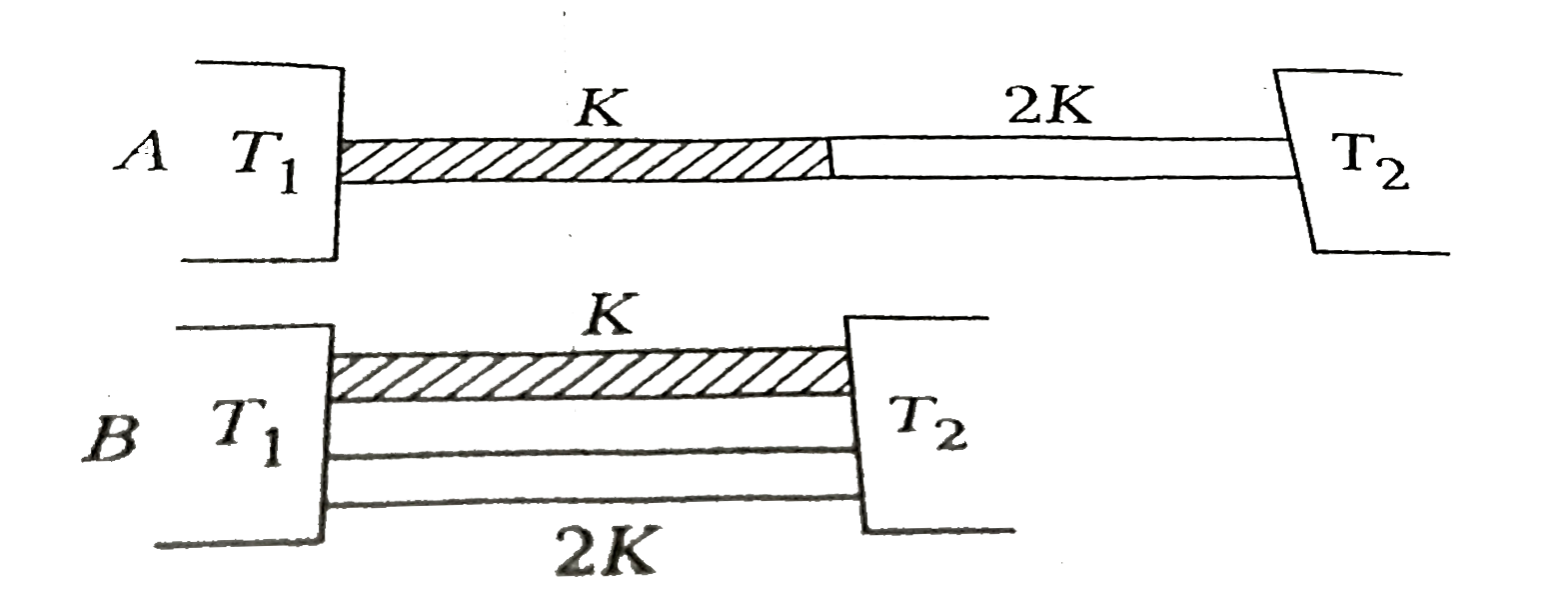
Two rods with the same dimensions have thermal conductivities in the r

Two identical rods are made of different materials whose thermal conductivities are $K_{1} and K_{2}$ . They are placed end to end between two heat reservoirs at temperatures $θ_{1} and θ_{2}$ . The temperature of the junction of the rod is

A $\frac{θ_{1} + θ_{2}}{2}$
B $\frac{K_{1} θ_{1} + K_{2} θ_{2}}{K_{1} + K_{2}}$
C $\frac{K_{1} θ_{2} + K_{2} θ_{1}}{K_{1} + K_{2}}$
D $\frac{K_{1} θ_{1} + K_{2} θ_{2}}{[K_{1} - K_{2}]}$
Two cylindrical conductors A and B of same metallic material have their diameters in the ratio 1:2 and lengths in the ratio 2:1. If the temperature difference between their ends is same, the ratio of heats conducted respectively by A and B per second is,
A $1 : 2$
B $1 : 4$
C $1 : 16$
D $1 : 8$
The ends of the two rods of different materials with their lengths, diameters of cross-section and thermal conductivities all in the ratio 1 : 2 are maintained at the same temperature difference. The rate of flow of heat in the shorter rod is $1 c a l s^{- 1}$ . What is the rate of flow of heat in the larger rod:
A $1 c a l s^{- 1}$
B $4 c a l s^{- 1}$
C $8 c a l s^{- 1}$
D $16 c a l s^{- 1}$

The correct Answer is:D