Jeremy will fill a rectangular crate that has inside dimensions of 18 inches by 15 inches by 9 inches with cubical tiles, each with edge lengths of 3 inches. If the tiles are packaged in sets of 8, how many packages will jeremy needs to completely fill the crate?
11
12
90
101
The correct Answer is:B
To solve the problem step by step, we need to calculate the volume of the rectangular crate, the volume of one cubical tile, and then determine how many packages of tiles Jeremy will need to fill the crate completely.
Step 1: Calculate the Volume of the Rectangular Crate
The volume V of a rectangular crate can be calculated using the formula:
V=length×width×height
Given the dimensions of the crate are 18 inches, 15 inches, and 9 inches, we can substitute these values into the formula:
V=18inches×15inches×9inches
Calculating this:
V=18×15=270square inches
V=270×9=2430cubic inches
Step 2: Calculate the Volume of One Cubical Tile
The volume Vt of a cube is calculated using the formula:
Vt=edge length3
Given that the edge length of the cubical tile is 3 inches:
Vt=3inches×3inches×3inches=33=27cubic inches
Step 3: Calculate the Total Number of Cubical Tiles Needed
To find the total number of tiles N needed to fill the crate, we divide the volume of the crate by the volume of one tile:
N=VVt=2430cubic inches27cubic inches
Calculating this:
N=90
So, Jeremy needs 90 tiles to fill the crate.
Step 4: Calculate the Number of Packages Required
Since the tiles are packaged in sets of 8, we need to determine how many packages P are required:
P=N8=908=11.25
Since Jeremy cannot buy a fraction of a package, he will need to round up to the nearest whole number:
P=12
Final Answer
Jeremy needs 12 packages of tiles to completely fill the crate.
---
The volume V of a rectangular crate can be calculated using the formula:
V=length×width×height
Given the dimensions of the crate are 18 inches, 15 inches, and 9 inches, we can substitute these values into the formula:
V=18inches×15inches×9inches
Calculating this:
V=18×15=270square inches
V=270×9=2430cubic inches
The volume Vt of a cube is calculated using the formula:
Vt=edge length3
Given that the edge length of the cubical tile is 3 inches:
Vt=3inches×3inches×3inches=33=27cubic inches
To find the total number of tiles N needed to fill the crate, we divide the volume of the crate by the volume of one tile:
N=VVt=2430cubic inches27cubic inches
Calculating this:
N=90
So, Jeremy needs 90 tiles to fill the crate.
Since the tiles are packaged in sets of 8, we need to determine how many packages P are required:
P=N8=908=11.25
Since Jeremy cannot buy a fraction of a package, he will need to round up to the nearest whole number:
P=12
Jeremy needs 12 packages of tiles to completely fill the crate.
Topper's Solved these Questions
- PROBLEM SETSBOOK - PRINCETONCHAPTER - PROBLEM SETSEXERCISE - PROBLEM SET 13: ADVANCED GEOMETRY
11 Videos
- PROBLEM SETSBOOK - PRINCETONCHAPTER - PROBLEM SETSEXERCISE - PROBLEM SET 14: FUNCTIONS
11 Videos
- PROBLEM SETSBOOK - PRINCETONCHAPTER - PROBLEM SETSEXERCISE - PROBLEM SET 11: TRIANGLES
11 Videos
- PRACTICE TEST 4BOOK - PRINCETONCHAPTER - PRACTICE TEST 4EXERCISE - Math Test-Calculator
38 Videos
- SAT MATH: THE BIG PICTUREBOOK - PRINCETONCHAPTER - SAT MATH: THE BIG PICTUREEXERCISE - Example
4 Videos
Similar Questions
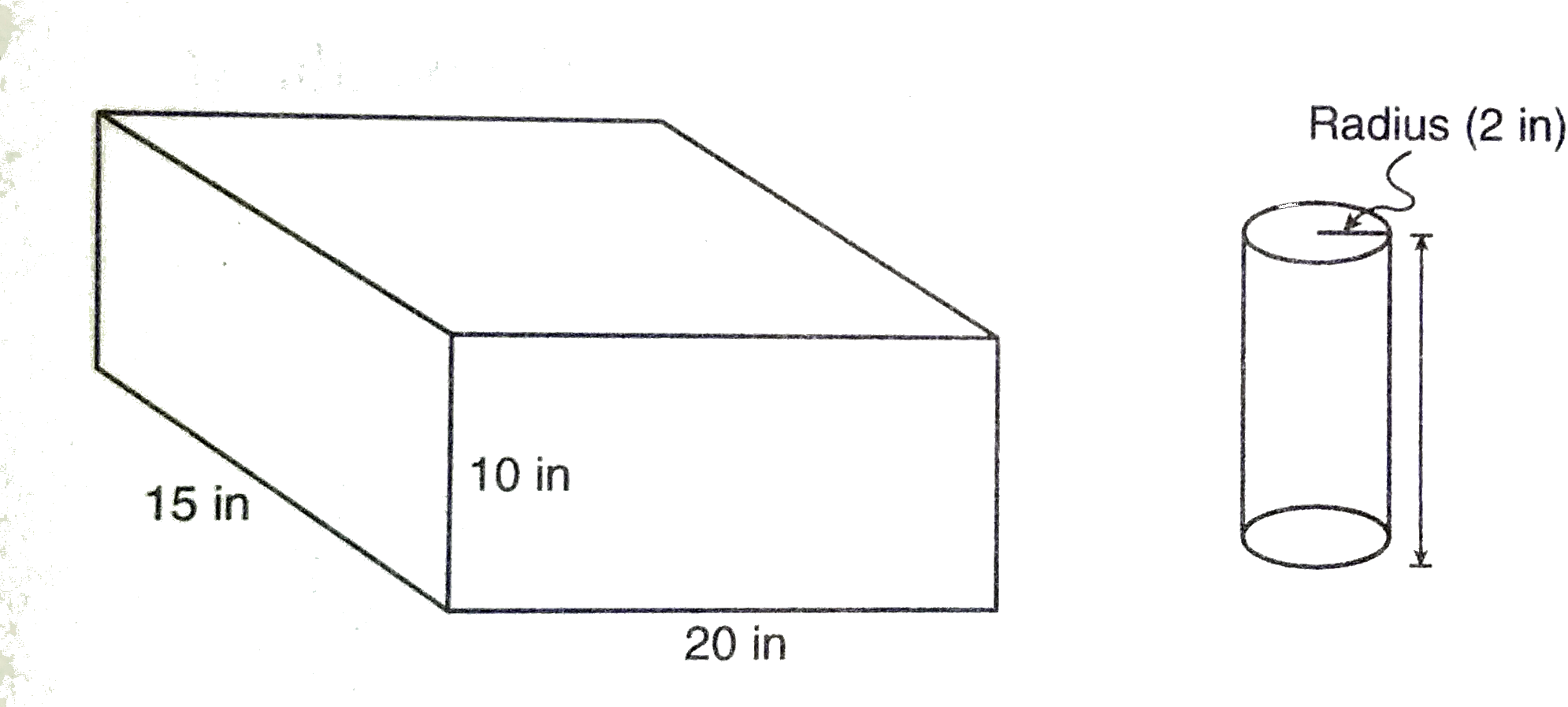
In the figure above, a rectangular container with the dimensions 10 inches by 15 inches by 20 inches is to be filled with water, using a cylindrical cup whose radius is 2 inches and whose height is 5 inches. What is the maximum number of full cups of water that can be placed into the container without the water overflowing the container?
View SolutionA mason has to fit a bathroom with square marble tiles of the largest possible size. The size of the bathroom is 10 ft. by 8 ft. What would be the size in inches of the tile required that has to be cut and how many such tiles are required?
View Solution
Knowledge Check
Milk is poured from a full rectangular container with dimensions 4 inches by 9 inches by 10 inches into cylindrical container with a diameter of 6 inches. Assuming all the milk is transferred without spilage, how many inches high will the milk reach in the cylindrical container?
A40πB60πC24D30A company ships notepads in rectangular boxes that each have inside dimensions measuring 9 inches long, 9 inches wide, and 12 inches tall. Each notepad is in the shape of a cube with an edge length of 3 inches. What is the maximum number of natepads that will fit in 1 closed box?
A10B11C12D36A rectangular fish tank fish has a base 2 feet wide and 2 feet long. When the tank is partially filled with water, a solid cube with an edge length of 1 foot is placed in the tank. If no overflow of water from the tank is assumed, by how many inches will be level of the water in the tank rise when the cube becomes completely submerged?
A16B12C2D3
A can of soda pop has the shape of a right circular cylinder with an inside height of 6 inches and an inside diameter of 2 inches. When you pour the soda pop from the full can into a cylindrical glas with an inside diameter of 3 inches, about how many inches high is the soda pop in the glass?
(Note: The volume of a right circular cylinder is πr2h)
View SolutionThe Northampton Volunteer Association has built a rectangular sandbox for a local elementary school and is ready to fill it with sand. The sandbox is 60 inches wide, 72 inches long, and will be filled 18 inches deep. Under the assumption that 1 bag of sand can fill 3,600 cubic inches of the sandbox, what is the minimum number of bags of sand they will need in order to fill the sandbox ?
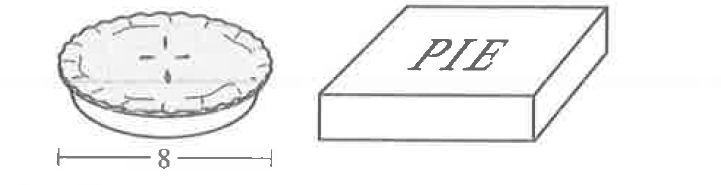
View SolutionA 3-inch-tall rectangular box with a square base is constructed to hold a circular pie that has a diameter of 8 inches. Both are shown below. What is the volume, in cubic inches, of the smallest such box that can hold this pie?
View SolutionAlma pours water into a small cylindrical glass with a height of 6 inches and a diameter of 3 inches. The water fills the glass to the very top, so she decides to pour it into a bigger glass that is 8 inches tall and 4 inches in diamter. Assuming alma doesn't spill any when she pours, how many inches high will the water reach in he bigger glass ?
View SolutionA crate in the shape of a right rectangular prism can hold 8 feet by 4 feet by 3 feet worth of material. At a particular hardware store, the price of brick is $1.20 per cubic foot. How much would it cost to completely fill the crate with bricks, such that there is no space remaining in the crate?
View Solution
