Of all the houses in a certain neighorhood, 80% have garages. Of those houses with garages, 60% have two car garages. If there are 56 houses with garages that are not two car garages, how many houses are there in the neighborhood?
93
117
156
175
The correct Answer is:D
To solve the problem, we will follow these steps:
1. Define the total number of houses: Let the total number of houses in the neighborhood be x.
2. Calculate the number of houses with garages: Since 80% of the houses have garages, the number of houses with garages can be calculated as:
Number of houses with garages=0.8x
3. Determine the percentage of houses with two-car garages: It is given that 60% of the houses with garages have two-car garages. Therefore, the number of houses with two-car garages is:
Number of houses with two-car garages=0.6×(0.8x)=0.48x
4. Calculate the percentage of houses with one-car garages: The remaining houses with garages must have one-car garages. Since 60% have two-car garages, 40% must have one-car garages:
Number of houses with one-car garages=0.4×(0.8x)=0.32x
5. Set up the equation based on the given information: We know that there are 56 houses with one-car garages. Therefore, we can set up the equation:
0.32x=56
6. Solve for x: To find the total number of houses, we divide both sides of the equation by 0.32:
x=560.32
7. Calculate the value of x:
x=175
Thus, the total number of houses in the neighborhood is 175.
Number of houses with garages=0.8x
Number of houses with two-car garages=0.6×(0.8x)=0.48x
Number of houses with one-car garages=0.4×(0.8x)=0.32x
0.32x=56
x=560.32
x=175
Topper's Solved these Questions
- ADVANCED ARITHMETICBOOK - PRINCETONCHAPTER - ADVANCED ARITHMETICEXERCISE - Examples
25 Videos
- ADVANCED ARITHMETICBOOK - PRINCETONCHAPTER - ADVANCED ARITHMETICEXERCISE - Examples
25 Videos
- ADDITIONAL TOPICSBOOK - PRINCETONCHAPTER - ADDITIONAL TOPICSEXERCISE - QUICK QUIZ #9
3 Videos
- ALEGBRABOOK - PRINCETONCHAPTER - ALEGBRAEXERCISE - QUICK QUIZ #6
3 Videos
Similar Questions
An acidic solution is mixed so that the mass ratio of nitric acid to water is 2:7, IF a total of 270 grams of acid solution is mixed, how many more grams of water are there than the nitric acid?
View SolutionAn acidic solution is mixed so that the mass ratio of nitric acid to water is 2:7, IF a total of 270 grams of acid solution is mixed, how many more grams of water are there than the nitric acid?
View Solution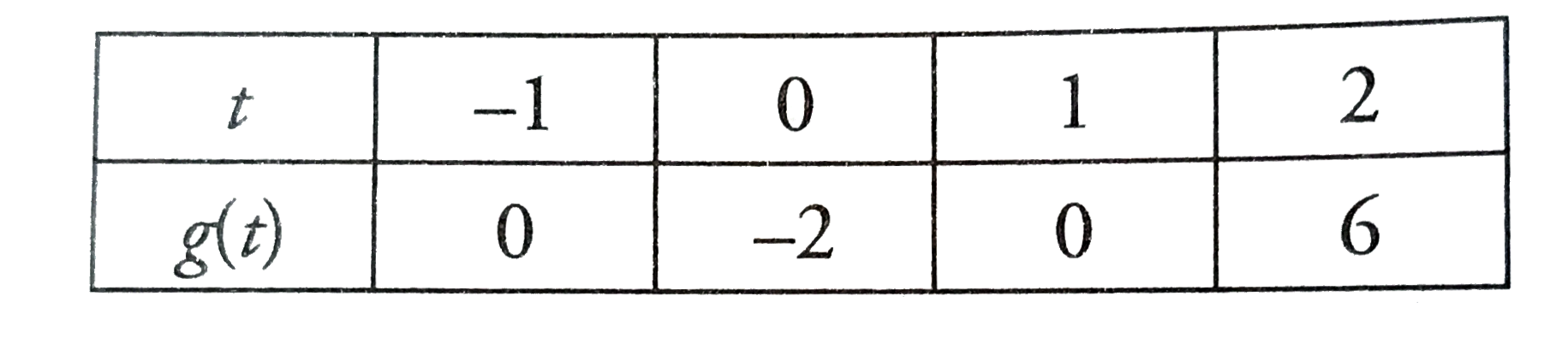
The table above provides values for the function g for selected values of t. Which of the following defines the function g?
View SolutionIf (3x)2=81, which of the following is a possible value of x?
View SolutionTwo car garages have a common gate which needs to open automatically when a car enters either of the garages or cars enter both. Devise a circuit that resembles this situation using diodes for the situation .
View SolutionWhat garages each charge a fixed amount, plus an hourly rate, to service a car. The garage on Main street charged one customer $153 for a 2 hour service appointment, and it charged a second customer $315 for a 5 hour service appointment. The garage on 2nd Street charges $5 lee per hour than the garages on Main Street and $10 more for the fixed amount. How much would the garage on 2nd Street charges for a 3 hour service appointment?
View SolutionDNA गाइरेज एन्जाइम, DNA के……...में सहायता करता है।
View SolutionWhat was the largest garage sale in history?
View SolutionMy dad ________ smoking in the garage.
View SolutionCar: Garage :: Aeroplane : ?
View SolutionChoose the correct alternative.
Car : Garage : : Aeroplane : ?
View SolutionTwo car garages have a common gate which needs to open automatically when a car enters either of the garages or cars enter both. Devise a circuit that resembles this situation using diodes for this situation.
View SolutionTwo car garages have a common gate which needs to open automatically when a car enters either of the garages or cars enter both. Devise a circuit that resembles this situation using diodes for the situation .
View SolutionThere is a common automatic entrance of two car garages which open automatically when a car is entered in one of the garages or cars are entered in both garages. Create circuits for this type of situation using diodes.
View SolutionTwo car garages have a common gate which needs to open automatically when a car enters either of the garages or cars enter both. Devise a circuit that resembles this situation using diodes for the situation .
View Solution
