x<4−2y
y≤−2x+1
Which of the following orfdered pairs satisfies both of the inequalities above ?
A
(−1,3)
B
(1,1)
C
(2,−3)
D
(4,4)
Video Solution
Text Solution
The correct Answer is:C
You could plot the two lines and identify the solution set on a graph, but testing the choices to see if they satisfy both inequalities is a more efficient approach, particularly since one inequality is "less than" and the other is "less than or equal to." If you take this approach, you don't even need to realrange the inequalities to isolate y on one side.
(A): Substtuting these values for x and y in x<4−2y gives you (−1)<4−2(3), whihc is −1<=−2 (not a true statemetn). You don't need to evaluate the other inequality since this ordered pair is not in the solution set for x<4−2y. Eliminate (A).
(B): Substituting these values for x and y in x<4−2y gives you (1)<4−2(1), which is 1<2. The ordered pair is in the solution set for this inequality, so plug the values into y≤−1. Thus, this ordered pair is not in the solution set for the second inequality. Eliminate (B).
(C ): Substituting these values for x and y in x<4−2y gives you (2)<4−2(−3), whihc is 2<10. The ordered pair is in thte solution set for this inequality, so plug the values into y≤−2x+1 to get (−3)≤−2(2)+1. which simplifies to −3≤−3. Since the sign for this inequality is "less than or equal to," this ordered pair is in the solution set. (C ) is correct.
(D): Since you already identified the correct choice, you do not need to check this pair.
|
Topper's Solved these Questions
- INEQUALITIESBOOK - KAPLANCHAPTER - INEQUALITIESEXERCISE - LINEAR INEQUALITIES
1 Video
- INEQUALITIESBOOK - KAPLANCHAPTER - INEQUALITIESEXERCISE - SYSTEM OF INEQUALITIES
1 Video
- INEQUALITIESBOOK - KAPLANCHAPTER - INEQUALITIESEXERCISE - MODELING REAL-LIFE SITUATIONS WITH INEQUALITIES
1 Video
- IMAGINARY NUMBERS BOOK - KAPLANCHAPTER - IMAGINARY NUMBERS EXERCISE - ARITHMETIC OPERATIONS WITH COMPLEX NUMBERS
1 Video
- LINEAR EQUATIONS AND GRAPHSBOOK - KAPLANCHAPTER - LINEAR EQUATIONS AND GRAPHSEXERCISE - LINEAR GRAPH
1 Video
Similar Questions
Knowledge Check
x+y=0
3x−2y=10
Which of the following ordered pairs (x y) satisfies the system of equations above?A(3, −2)B(2, −2)C(−2, 2)D(−2, −2)13x−16y=7
15y−15x=8
Which of the following ordered pairs (x, y) satisfies the system of equations above?A(−36,−57)B(12,43)C(1015,3074)D(82,122)−2y≤8
y−3≤x
−13y+1≥x
Which of the following graphs shows the solutions to the system of inequalities above?A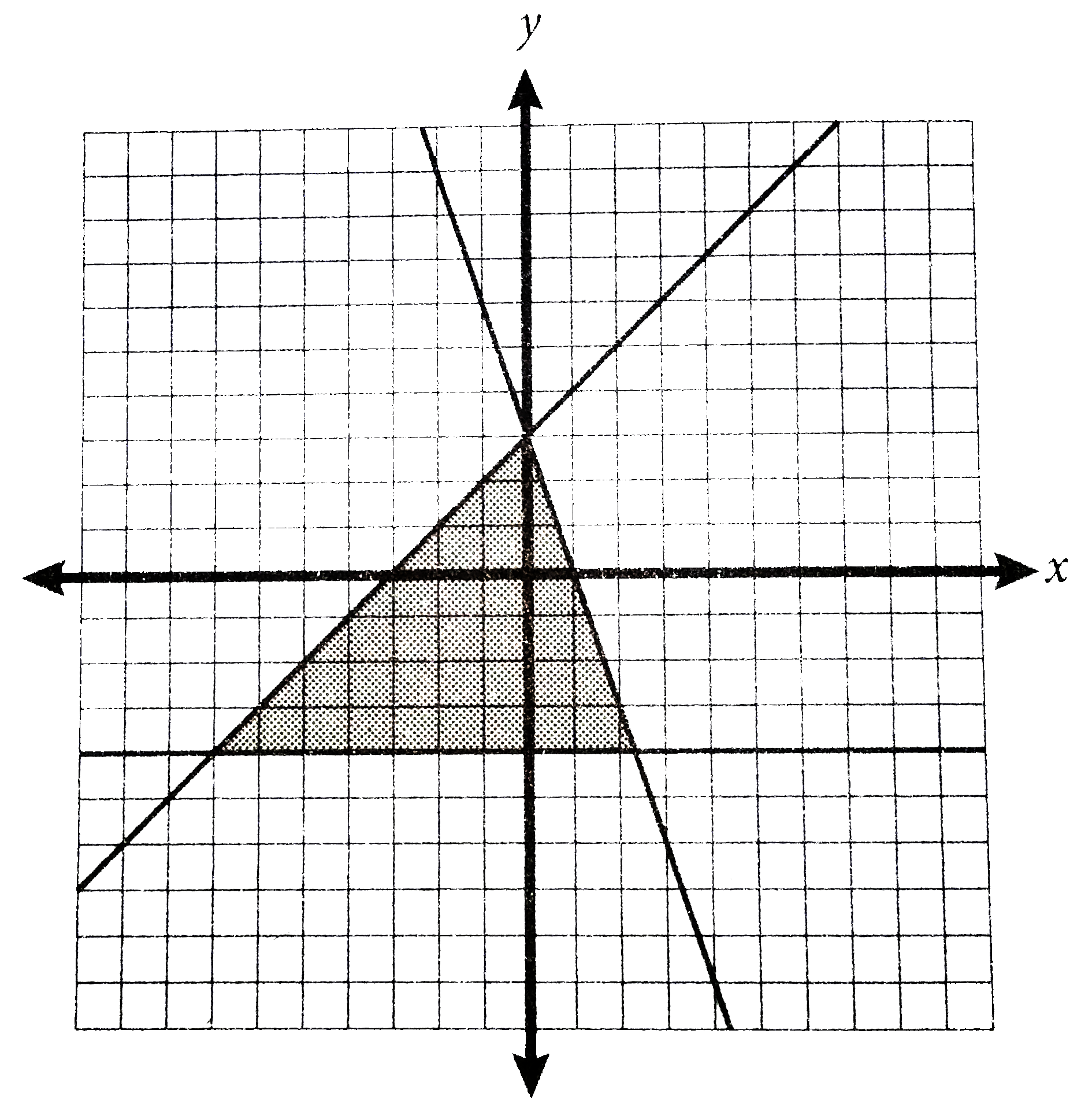 B
B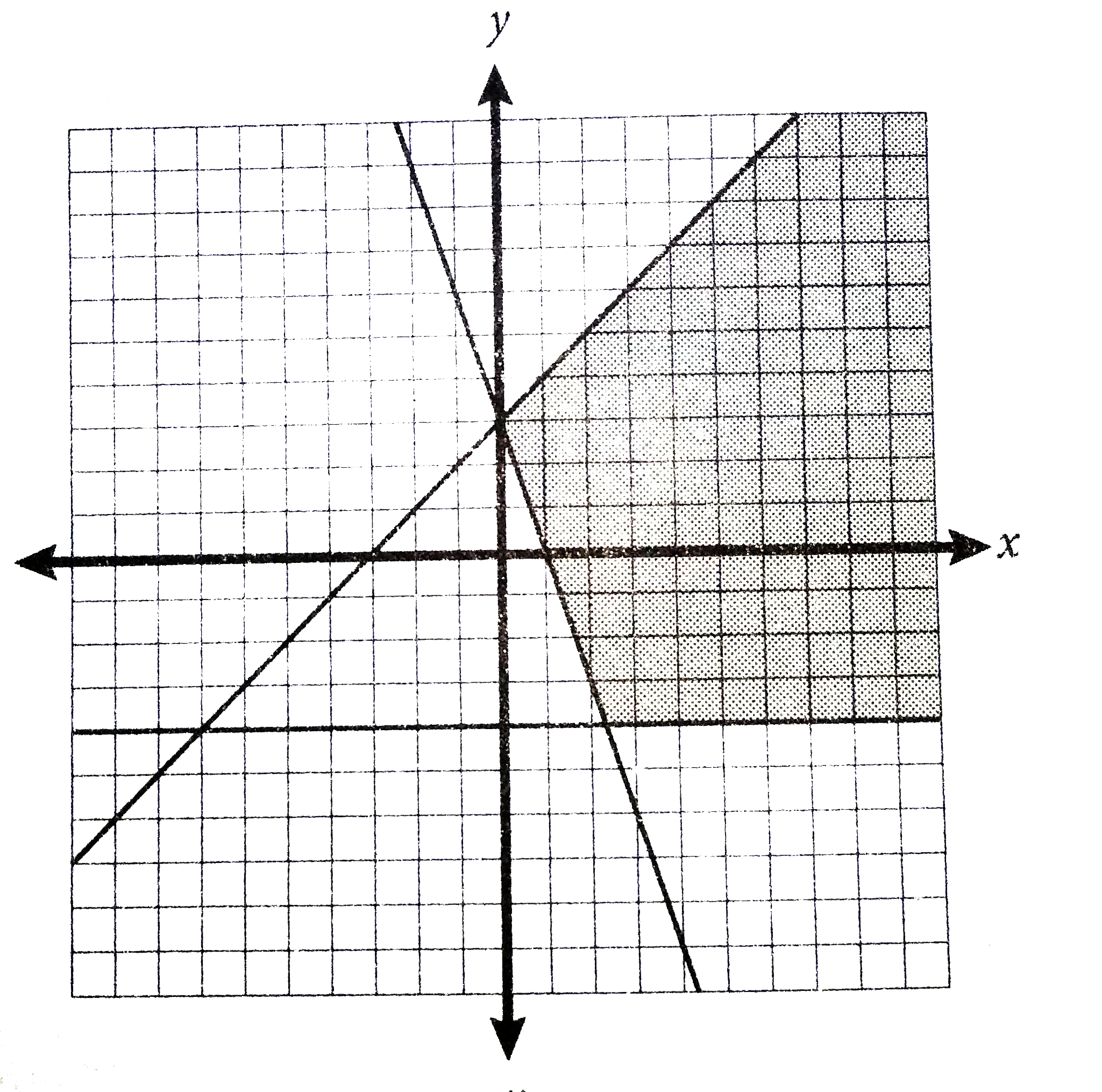 C
C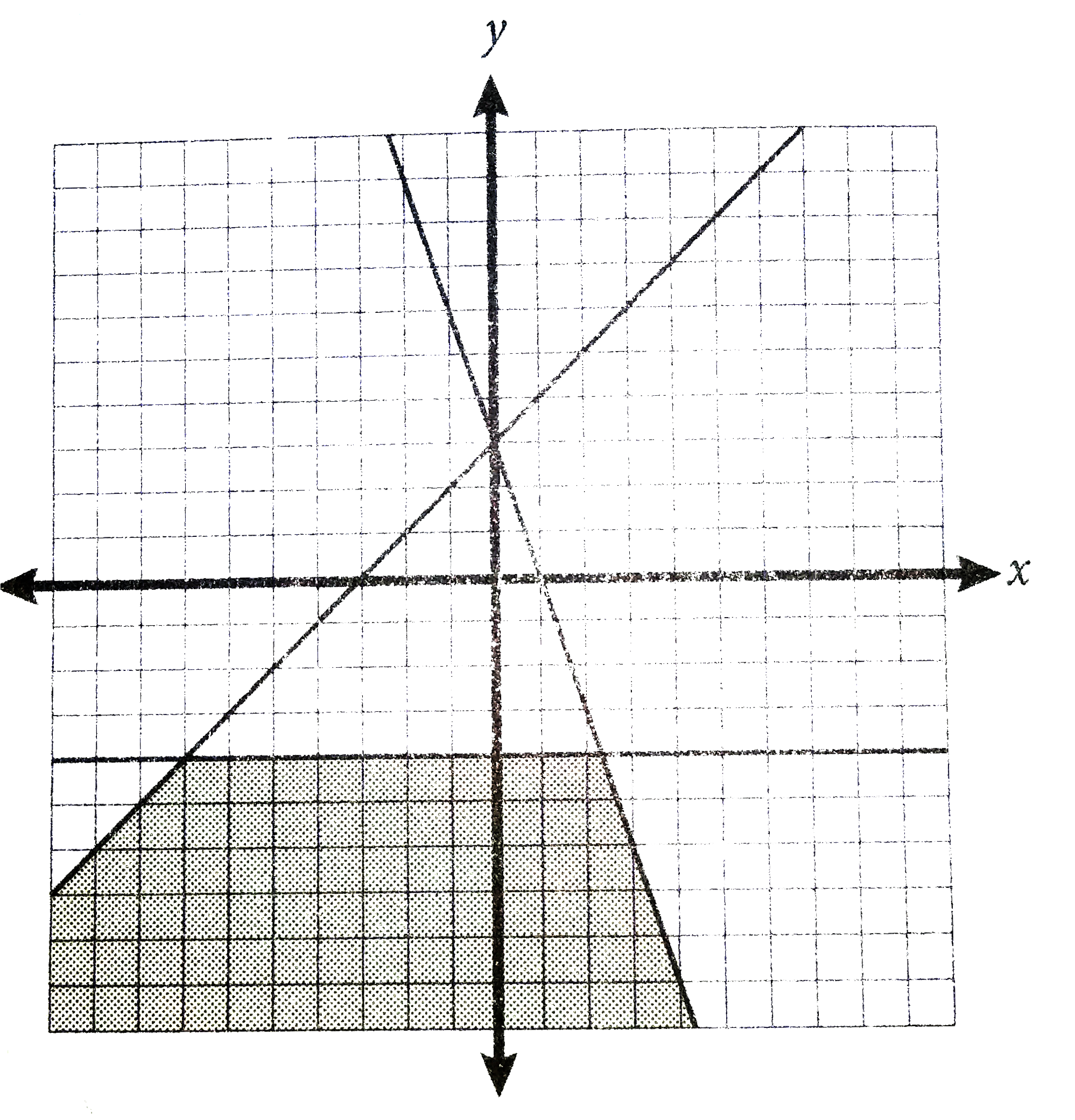 D
D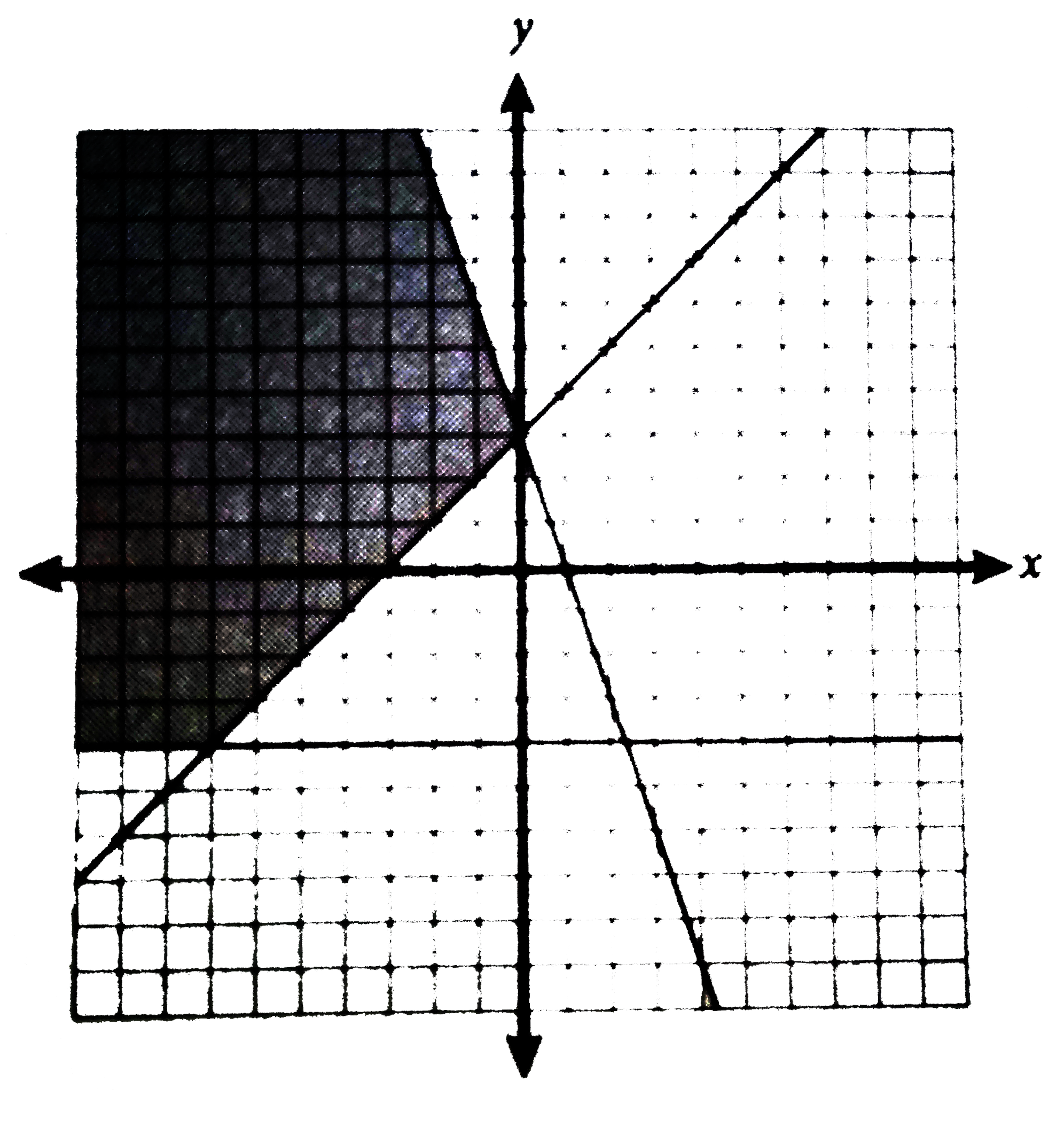
x=y-3
x2+2y=6
Which ordered pair (x, y) satisfies the system of equations shown above?
View SolutionWhich of the following ordered pairs (x, y) satisfies the inequality 5x − 3y lt 4 ?
I.(1,1)
II.(2,5)
III.(3,2)
View Solutiony≤3x+1
x−y>1
View SolutionWhich of the following ordered pairs in the standard (x,y) coordinate plane satisfies the system of inequalities below?
x>2
y>0
x+y<5
View Solutionx=2y+5
y=(2x−3)(x+9)
How many ordered pairs (x,y) satisfy the system of equations shown above ?
View Solution

