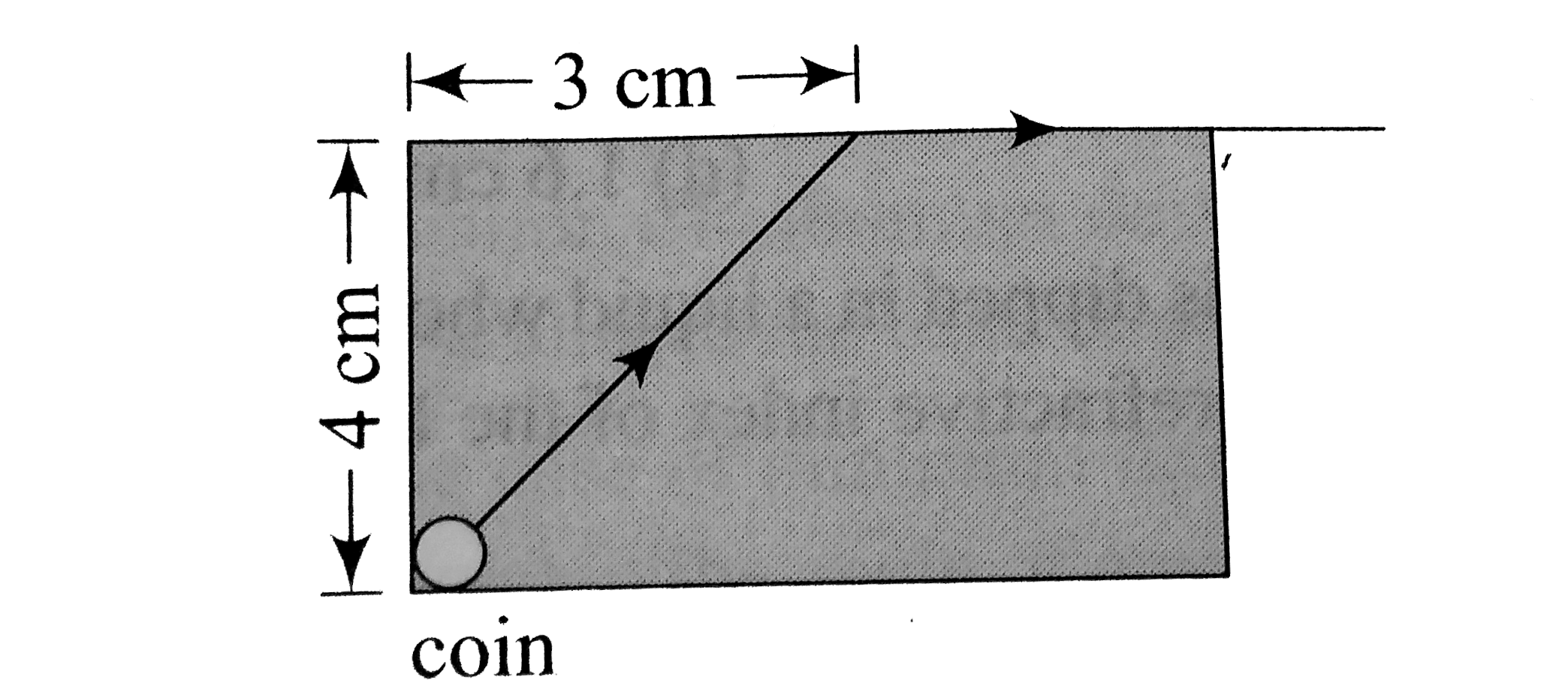
A small coin is resting on the bottom of a beaker filled with a liquid. A ray of light from the coin travels up to the surface of the liquid and moves along its surface (see figure ).
How fast is the light travelling in the liquid ?
1.8×108ms−1
2.4×108ms−1
3.0×108ms−1
1.2×108ms−1
(a) As shown in figure, a light ray from the the coin will not emerge out of liquied if i>C.
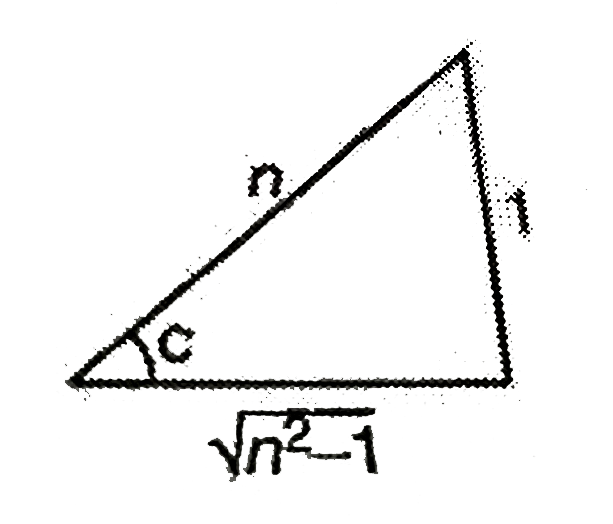
Therefore, minimum radius R corresponds to i=C. In ΔSAB,
Rh=tanC or R=htanC
or R=h√n2−1

[∵sinC=1n and tanC=sinC√1−sin2C]
Given R=3cm,h=4cm
Hence 34=1√n2−1
or n2=259 or n=53
But n=cv or v=cn=3×1085/3=1.8×108ms−1
Topper's Solved these Questions
Similar Questions
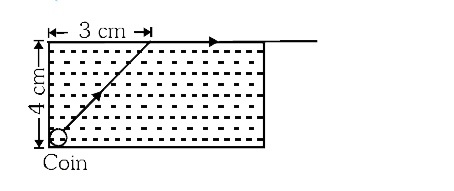
A small coin is resting on the bottom of a beaker filled with a liquid. A ray of light from the coin travels upto the surface of the liquid and moves along its surface How fast is the light travelling the liquid ?
View SolutionA wooden ball of density σ is released from the bottom of a tank which is filled with a liquid of density ρ(ρ>σ)up to a height h1. The ball rises in the liquid, emerges from its surface and attains a height h2 in air.If viscous effects are neglected,the ratio h2h1 is
View Solution
Knowledge Check
A small coin is resting on the bottom of a beaker filled with liquid. A ray of light from the coin travels upto the surface of the liquid and moves along its surface. How fast is the light travelling in the liquid?
 A2.4×108ms−1B3.0×108ms−1C1.2×108ms−1D1.8×108ms−1
A2.4×108ms−1B3.0×108ms−1C1.2×108ms−1D1.8×108ms−1A small coin is resting on the bottom of a beaker filled with a liquid. A ray of light from the coin travels upto the surface of the liquid and moves along its surface How fast is the light travelling the liquid ?

A1.8×108ms−1B2.4×108ms−1C3.0×108ms−1D1.2×108ms−1A ray of light travelling in air falls obliquely on the surface of a clam poun. It will
Ago into the water without deviating from its pathBdeviate away from the normalCdeviate towards the normalDTurn back on its original path
A metal coin at the bottom of a beaker filled with a liquid of refractive index 4/3 to height of 6cm. To an observer looking from above the surface of the liquid, coin will appear at a depth of (consider paraxial rays).
View SolutionA container filled with a liquid A is floating on the surface of another liquid 'B' as shown in the figure, then volume of the liquid displaced by the container
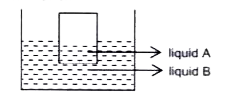
View SolutionA container filled with a liquid A is floating on the surface of another liquid 'B' as shown in the figure, then volume of the liquid displaced by the container

View SolutionWhen a ray of light travels perpendicular to the surface of separation of two mediums, it
View SolutionA beaker of radius 15 cm is filled with a liquid of surface tension 0.075 N/m. Force across an imaginary diameter on the surface of the liquid is
View Solution