An electron is released from the bottom plate A as shown in the figure (E=104N/C). The velocity of the electron when it reaches plate B will be nearly equal to

0.85×107ms−1
1.0×107ms−1
1.25×107ms−1
1.65×107ms−1
The correct Answer is:A
Step by step video, text & image solution for An electron is released from the bottom plate A as shown in the figure (E = 10^(4) N//C). The velocity of the electron when it reaches plate B will be nearly equal to by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Assertion and Reason
15 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Match the columns
5 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Check point 1.5
20 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEYCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - All Questions
120 Videos
Similar Questions
A block of A is released from rest from the top of wedge block of height h shown in figure-4.55. If velocity of block when it reaches the bottom of inclive is v0, find the time os sliding.
View SolutionAn electron is released from rest in a uniform electric field of magnitude 2.00×104N/C . (a) Calculate the acceleration of the electron. (Ignore gravitational) (b) How much time does the electron take to reach 1.00 % of the speed of light ?
View Solution
Knowledge Check
The wavelength of an electron , moving with the velocity 3×103 m/s , is nearly equal to
A2×10−12mB6×10−13 mC2.5×10−7 mD8×10−10mTwo charged metal plates in vacuum are 10cm apart. A uniform electric field of intensity (45/16)×103NC−1 is applied between the plates. An electron is released between the plates from rest at a point just outside the negative plate.
(i) Calculate how long (t) will the electron take to reach the other plate?
(ii) At what velocity (v) will it be electron take to hits the other plate?A(i) 2×10−6s (ii) 105ms−1B(i) 3×10−8s (ii) 106ms−1C(i) 2×10−8s (ii) 107ms−1D(i) 3×10−6s (ii) 107ms−1In the uniform electric field of E=1×104NC−1, an electron is accelerated from rest. The velocity of the electron when it has travelled a distance of 2×10−2 m is nearly
(em of electron =1.8×1011Ckg−1)A1.6×106ms−1B0.85×106ms−1C0.425×106ms−1D8.5×106ms−1
A uniform magnetic field (B) is applied between the plates of a capacitor in the outward direction as shown in the figure. Surface charge density on the capacitor plates is σ.
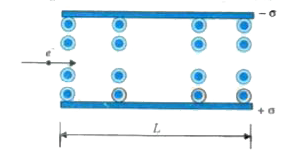
An electron is fired in between the plates, parallel to them, as shown in the figure. The electron is found to move straight in-between the plates of capacitor. Neglect the gravity. If L is the length of the plates then caleulate the time taken by the electron to cross the plates.
View SolutionThere exists a uniform and constant magnetic field of strength B in the space betweent he plates of a charged parallel plate capacitor. The charge density on the plate is σ and length of the plate is l. An electron is projected in the space between the plates along the length of the plate. It is foudn that velcoity of the electron does not change. Find the time taken by the electron to come out of the capacitor. The figure describes the situtation. Ignore the gravity.

View SolutionThe potential difference between two large parallel plates is varied as v = at, a is a positive constant and t is time. An electron starts from rest at t=0 from the plate which is at lower potential. If the distance between the plates is L, mass of electron m and charge on electron -e then find the velocity of the electron when it reaches the other plate.
View SolutionAn electron enters the region between the plates of a parallel plate capacitor at a point equidistance from either plate. The capacitor plates are 2×10−2m apart and 10−1m long. A potential difference of 300 V is kept across the plates. Assuming that the initial velocity of the electron is parallel to the capacitor plates, calculate the largest value of the velocity of the electrons so that they do not fly out of the capacitor at the other end.
View SolutionAt some instant the velocity components of an electron moving between two charged parallel plates are vx=1.5×105m/s and vy=3.0×106m/s.Suppose that the electric field between the plates is given by E=(120N/C)ˆj
. (a) What is the acceleration of the electron?
(b) What will be the velocity of the electron after its x-coordinate has changed by 2.0 cm?
View Solution
