Two identical charged spheres of material density ρ, suspended from the same point by inextensible strings of equal length make an angle θ between the string. When suspended in a liquid of density σ the angle θ remains the same. The dielectric constant K of the liquid is
ρρ−σ
ρ−σρ
ρρ+σ
ρ+σρ
The correct Answer is:A
To solve the problem, we need to analyze the forces acting on the charged spheres when they are suspended in air and then in a liquid.
Step 1: Analyze the forces acting on the spheres in air
When the two charged spheres are suspended in air, they experience:
- Gravitational force acting downwards: Fg=mg=Vρg
- Electrostatic force acting horizontally due to repulsion between the two charged spheres: Fe=k⋅q2r2
Where:
- m is the mass of the sphere,
- V is the volume of the sphere,
- ρ is the density of the sphere,
- g is the acceleration due to gravity,
- q is the charge on each sphere,
- r is the distance between the centers of the spheres.
Step 2: Set up the equilibrium condition in air
In equilibrium, the horizontal and vertical forces must balance. The vertical component of the tension T in the strings must balance the weight of the spheres, and the horizontal component must balance the electrostatic force.
Let T be the tension in the strings. The angle θ gives us:
- Vertical component: Tcos(θ)=mg
- Horizontal component: Tsin(θ)=Fe
Step 3: Analyze the forces acting on the spheres in the liquid
When the spheres are submerged in a liquid of density σ, they experience:
- Gravitational force acting downwards: F′g=mg−Vσg (the buoyant force reduces the effective weight)
- The electrostatic force remains the same as in air.
Step 4: Set up the equilibrium condition in the liquid
In the liquid, the equilibrium conditions are similar:
- Vertical component: T′cos(θ)=mg−Vσg
- Horizontal component: T′sin(θ)=Fe
Step 5: Relate the two cases
Since the angle θ remains the same in both cases, we can equate the ratios of the forces:
Tsin(θ)Tcos(θ)=Femg(in air)
T′sin(θ)T′cos(θ)=Femg−Vσg(in liquid)
Step 6: Simplify the equations
From the above equations, we can derive:
Femg=Femg−Vσg
Step 7: Solve for the dielectric constant K
From the balance of forces, we can express the dielectric constant K as:
K=ρρ−σ
Final Answer
Thus, the dielectric constant K of the liquid is given by:
K=ρρ−σ
When the two charged spheres are suspended in air, they experience:
- Gravitational force acting downwards: Fg=mg=Vρg
- Electrostatic force acting horizontally due to repulsion between the two charged spheres: Fe=k⋅q2r2
- m is the mass of the sphere,
- V is the volume of the sphere,
- ρ is the density of the sphere,
- g is the acceleration due to gravity,
- q is the charge on each sphere,
- r is the distance between the centers of the spheres.
In equilibrium, the horizontal and vertical forces must balance. The vertical component of the tension T in the strings must balance the weight of the spheres, and the horizontal component must balance the electrostatic force.
- Vertical component: Tcos(θ)=mg
- Horizontal component: Tsin(θ)=Fe
When the spheres are submerged in a liquid of density σ, they experience:
- Gravitational force acting downwards: F′g=mg−Vσg (the buoyant force reduces the effective weight)
- The electrostatic force remains the same as in air.
In the liquid, the equilibrium conditions are similar:
- Vertical component: T′cos(θ)=mg−Vσg
- Horizontal component: T′sin(θ)=Fe
Since the angle θ remains the same in both cases, we can equate the ratios of the forces:
Tsin(θ)Tcos(θ)=Femg(in air)
T′sin(θ)T′cos(θ)=Femg−Vσg(in liquid)
From the above equations, we can derive:
Femg=Femg−Vσg
From the balance of forces, we can express the dielectric constant K as:
K=ρρ−σ
Thus, the dielectric constant K of the liquid is given by:
K=ρρ−σ
Topper's Solved these Questions
- ELECTROSTATICSBook:DC PANDEYChapter:ELECTROSTATICSExercise:Assertion and Reason
Explore 15 Videos
- ELECTROSTATICSBook:DC PANDEYChapter:ELECTROSTATICSExercise:Match the columns
Explore 5 Videos
- ELECTROSTATICSBook:DC PANDEYChapter:ELECTROSTATICSExercise:Check point 1.5
Explore 20 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBook:DC PANDEYChapter:ELECTROSTATIC POTENTIAL AND CAPACITORSExercise:(C) Chapter exercises
Explore 50 Videos
- GRAVITATIONBook:DC PANDEYChapter:GRAVITATIONExercise:All Questions
Explore 120 Videos
Similar Questions
Two identically charged spheres are suspended by strings of equal length. The strings make an angle of 30∘ with each other. When suspended in a liquid of density 0.8g/cc the angle remains same. What is the dielectric constant of liquid. Density of sphere=1.6g/cc
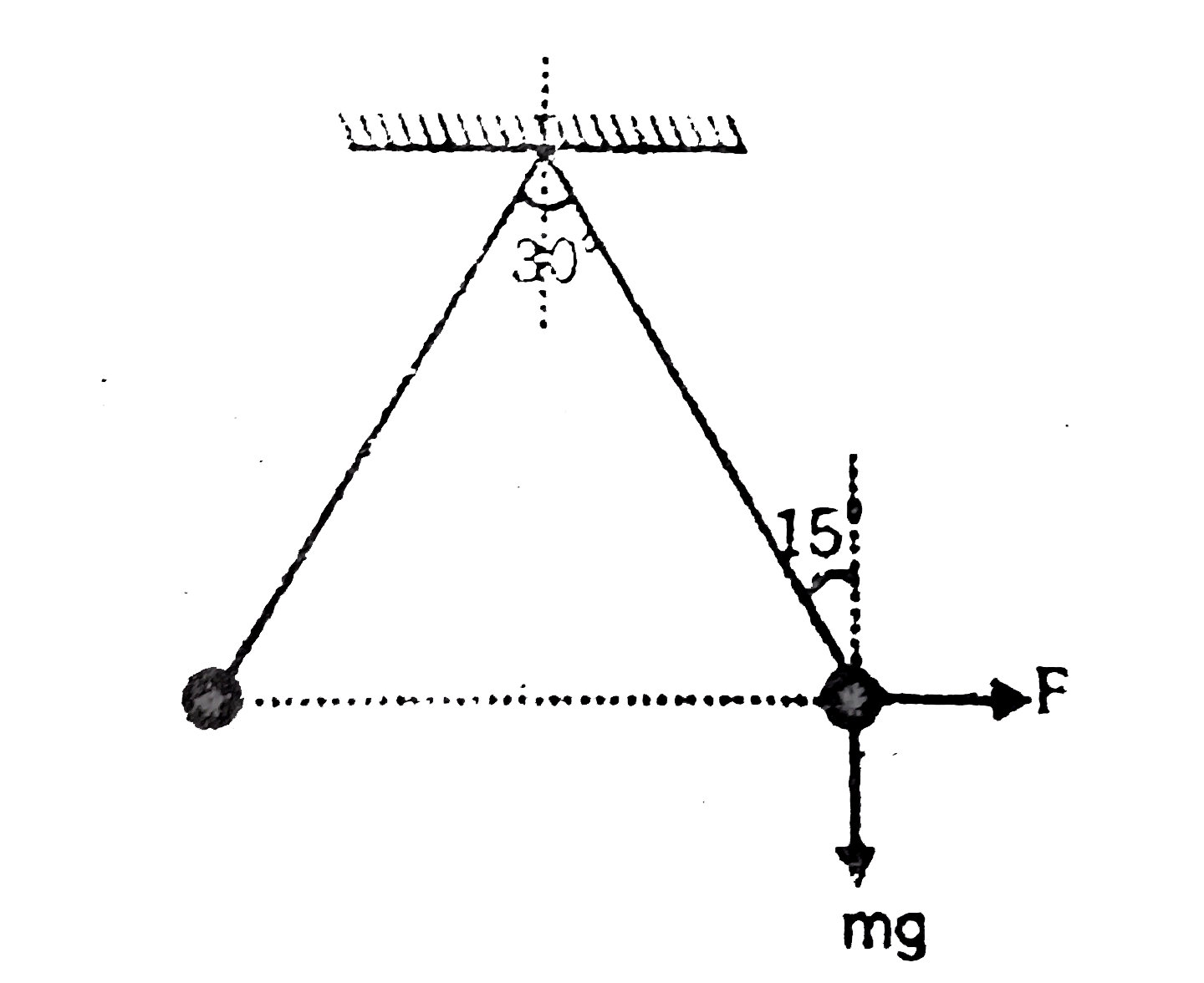
View SolutionTwo indentical pith balls, each carrying charge q, are suspended from a common point by two strings of equal length l. Find the mass of each ball if the angle between the strings is 2θ in equilibrium.
View Solution
Knowledge Check
Two identical charged spheres are suspended by strings of equal lengths. The strings make an angle of 30∘ with each other. When suspended in a liquid of density 0.8gcm−3, the angle remains the same. If density of the material of the sphere is 1.6gcm−3, the dielectric constant of the liquid is
A(a) 4B(b) 3C(c) 2D(d) 1Two identical non - conducting solid spheres of same and charge are suspended in air form a common point by two non - conducing massless string of same length . At equilibrium the angle between the strings is α. The spheres are now immersed in a dielectric liquid of density 800kgm−3 and dielectric constant 21. If the angle between the spheres remains the same after the immersion , then
Aelectric force between the spheres reamins unchangedBelectic force between the sphere reduceCmass density of the spheres is 840kgm−3Dthe tension in the string holding the spheres remains unchangedIn a liquid medium of dielectric constant K and of spherical gravity 2 two identically charged spheres are suspended from a fixed point by threads of equal length .The angle between them is 90∘ .In another medium of unknown dielectric constant K1 and specific gravity 4 the angle between them become 120∘ if density of material of spheres is 8gm/cc thenK1 is
AK2B√3KC√32KDK√3
Two identical ball having mass density ρ , mass m and charge q are suspended from a common point by two insulating massless strings of equal length. At equilibrium each string makes an angle theta with the vertical. Now both the balls are immersed in a fluid. At equilibrium, theta remains same. If the mass density of liquid is σ then dielectric constant of liquid will be
View SolutionTwo small identical balls carrying the charges of the same sign are suspended from the same point by insulating threads of equal length. When the surrounding space was filled with kerosene the divergence angle between the threads remained constant, What is the density of the material of which the balls are made ?
View SolutionTwo identical spheres of mass m and charge q are suspended from a common point by two threads of the same length l and it is found that threads make an angle θ with the vertical when the spheres are in equilibrium . Calculate the value of q.
View SolutionTwo identically charged spherical objects are suspended from a common point , using two different threads of equal length. Due to repulsion between charges both strings are maintaining a constant angle with the vertical, and both the objects are in equilibrium. Use m as mass , L as length of each thread. The angle made by each thread with the vertical is θ. Calculate the charge on objects.
View SolutionIn a liquid medium of dielectric constant K and specific gravity 2 two identically charged spheres are suspended from a fixed point by threads of equal length .The angle between them is 90∘ .In another medium of unknown dielectric constant K1 and specific gravity 4 the angle between them become 120∘ if density of material of spheres is 8gm/cc thenK1 is
View Solution