Three concentric metallic spherical shells of radii R, 2R, 3R are given charges Q1Q2Q3, respectively. It is found that the surface charge densities on the outer surface of the shells are equal. Then, the ratio of the charges given to the shells Q1:Q2:Q3 is
1:2:3
1:3:5
1:4:9
1:8:18
The correct Answer is:B
To solve the problem, we need to find the ratio of the charges Q1:Q2:Q3 given to three concentric metallic spherical shells with radii R,2R, and 3R respectively, under the condition that the surface charge densities on their outer surfaces are equal.
1. Understanding Surface Charge Density:
The surface charge density σ on a spherical shell is defined as:
σ=QA
where Q is the charge on the shell and A is the surface area of the shell. For a sphere, the surface area A is given by 4πr2.
2. Surface Charge Densities for Each Shell:
- For the inner shell (radius R):
σ1=Q14πR2
- For the middle shell (radius 2R):
σ2=Q24π(2R)2=Q216πR2
- For the outer shell (radius 3R):
σ3=Q34π(3R)2=Q336πR2
3. Setting the Surface Charge Densities Equal:
Since it is given that the surface charge densities on the outer surfaces are equal, we can set:
σ1=σ2=σ3
This gives us the following equations:
Q14πR2=Q216πR2
Q216πR2=Q336πR2
4. Solving for Ratios:
From the first equation:
Q1=14Q2(1)
From the second equation:
Q2=1636Q3=49Q3(2)
5. Substituting Equation (2) into Equation (1):
Substitute Q2 from equation (2) into equation (1):
Q1=14(49Q3)=19Q3
6. Expressing All Charges in Terms of Q3:
Now we can express Q1 and Q2 in terms of Q3:
- From Q1=19Q3
- From Q2=49Q3
7. Finding the Ratio:
The charges can now be expressed as:
Q1:Q2:Q3=19Q3:49Q3:Q3
Simplifying this gives:
Q1:Q2:Q3=1:4:9
Final Answer:
The ratio of the charges given to the shells is:
Q1:Q2:Q3=1:4:9
The surface charge density σ on a spherical shell is defined as:
σ=QA
where Q is the charge on the shell and A is the surface area of the shell. For a sphere, the surface area A is given by 4πr2.
- For the inner shell (radius R):
σ1=Q14πR2
- For the middle shell (radius 2R):
σ2=Q24π(2R)2=Q216πR2
- For the outer shell (radius 3R):
σ3=Q34π(3R)2=Q336πR2
Since it is given that the surface charge densities on the outer surfaces are equal, we can set:
σ1=σ2=σ3
This gives us the following equations:
Q14πR2=Q216πR2
Q216πR2=Q336πR2
From the first equation:
Q1=14Q2(1)
From the second equation:
Q2=1636Q3=49Q3(2)
Substitute Q2 from equation (2) into equation (1):
Q1=14(49Q3)=19Q3
Now we can express Q1 and Q2 in terms of Q3:
- From Q1=19Q3
- From Q2=49Q3
The charges can now be expressed as:
Q1:Q2:Q3=19Q3:49Q3:Q3
Simplifying this gives:
Q1:Q2:Q3=1:4:9
The ratio of the charges given to the shells is:
Q1:Q2:Q3=1:4:9
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Assertion and Reason
15 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Match the columns
5 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Check point 1.5
20 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEYCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - All Questions
120 Videos
Similar Questions
There are three conecentric hollow spheres of radii a, b and c and they are charged with charges q1,q2 and q3 respectively. Calculate the potential of the spheres.
View SolutionThere are two concentric metallic shells of radii R and 2R, if outer shell is given charge q1 , then charge on surface of inner shell will be
View Solution
Knowledge Check
Three concentric metallic spherical shells of radii R, 2R, 3R are given charges Q1Q2Q3, respectively. It is found that the surface charge densities on the outer surface of the shells are equal. Then, the ratio of the charges given to the shells Q1:Q2:Q3 is
A1:2:3B1:3:5C1:4:9D1:8:18Three concentric metallic spherical shells of radii R, 2R, 3R, are given charges Q1, Q2, Q3, respectively. It is found that the surface charge denisties on the outer surfaces of the shells are equal. Then, the ratio of the charges given to the shells, Q1:Q2:Q3, is
A(a) 1:2:3B(b) 1:3:5C(c) 1:4:9D(d) 1:8:18Two concentric spherical shells of radii R and 2R are given charges Q1andQ2 respectively.
The surfaces charge densities of the outer surfaces are equal. Determine the ratio Q1:Q2.A1:2B4:1C1:4D2:1
Two concentric spherical shells of radius R1 and R2(R2>R1) are having uniformly distributed charges Q1 and Q2 respectively. Find out total energy of the system.

View SolutionTwo uniformly charged concentric spherical shells of radius R and r, are given charges Q1 and Q2 respectively. If their potential difference is V, then it will not depends upon:

View SolutionThe insulated spheres of radii R1 and R2 having charges Q1 and Q2 respectively are connected to each other. There is
View SolutionThere are three concentric metallic spherical shells of radii R, 2R and 3q, respectively. The middle spherical shell is connected to earth. Let q1 be the charge appearing on the inner surface of the middle sphere and q2 be the charge on outer surface of the middle sphere. Similarly q3 is the charge appearing on inner surface of outermost sphere and q4 is the charge appearing on outer surface of outermost sphere.
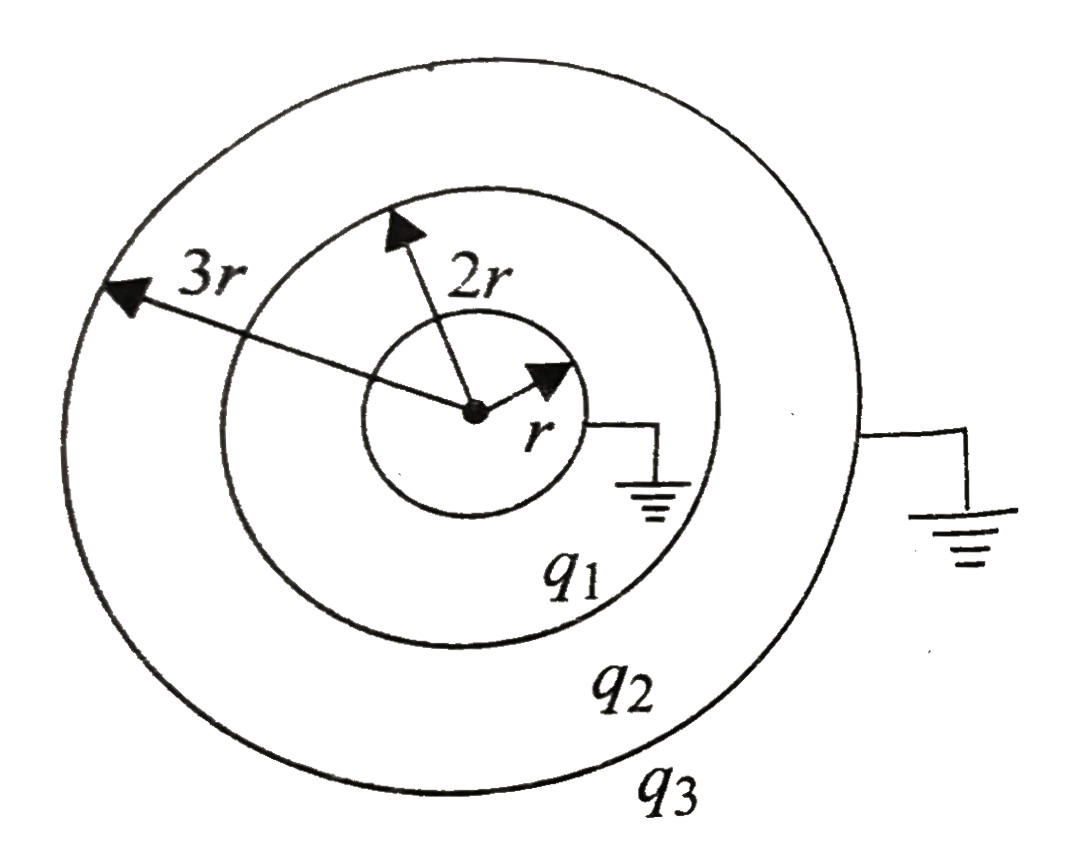
View SolutionThree concentric conducting spherical shells have radii r, 2r, and 3r and charges q1,q2andq3, respectively. Innermost and outermost shells are earthed as shown in figure. The charges shown are after earthing. Select the correct alternative.
View Solution
