Density of a planet is two times the density of earth. Radius of this planet is half. Match the following (as compared to earth)
Column-IColumn-II(A)Accleration due to gravity on this planet's surface(p)Half(B)Gravitational potential on the surface(q)Same(C)Gravitational potential at centre(r)Two times(D)Gravitational field strength at centre(s)Four times
The correct Answer is:(A→q,B→p,C→p,D→q)
To solve the problem, we need to analyze the properties of the planet in comparison to Earth based on the given information: the density of the planet is two times that of Earth, and the radius of the planet is half that of Earth.
1. Acceleration due to Gravity (g') on the Surface of the Planet:
The formula for acceleration due to gravity at the surface of a planet is given by:
g′=GMR2
where M is the mass of the planet and R is its radius. The mass M can be expressed in terms of density (ρ) and volume (V):
M=ρV=ρ(43πR3)
Substituting this into the equation for g′:
g′=Gρ(43πR3)R2=43GρR
Given that the density of the planet is 2ρE (where ρE is the density of Earth) and the radius is 12RE:
g′=43G(2ρE)(12RE)=43GρERE=gE
Thus, the acceleration due to gravity on the planet's surface is the same as that of Earth.
2. Gravitational Potential (V') on the Surface:
The gravitational potential at the surface is given by:
V′=−GMR
Substituting for M:
V′=−G(2ρE⋅43π(12RE)3)12RE
Simplifying:
V′=−G⋅2ρE⋅43π⋅18R3E12RE=−G⋅2ρE⋅43π⋅18R2E12=−G⋅2ρE⋅43π⋅14R2E1=−G⋅ρE⋅43πR2E1
This shows that the gravitational potential at the surface is the same as that of Earth.
3. Gravitational Potential at the Center:
The gravitational potential at the center of a solid sphere is given by:
Vc=−32GMR
For our planet:
V′c=−32G(2ρE⋅43π(12RE)3)12RE=−32⋅G⋅2ρE⋅43π⋅18R3E12RE
This simplifies to show that the gravitational potential at the center of the planet is two times that of Earth.
4. Gravitational Field Strength (E) at the Center:
The gravitational field strength inside a solid sphere is zero at the center. Therefore, the gravitational field strength at the center of the planet is also zero, just like on Earth.
Final Matching:
- (A) Acceleration due to gravity on this planet's surface → (q) Same
- (B) Gravitational potential on the surface → (q) Same
- (C) Gravitational potential at center → (r) Two times
- (D) Gravitational field strength at center → (s) Zero
The formula for acceleration due to gravity at the surface of a planet is given by:
g′=GMR2
where M is the mass of the planet and R is its radius. The mass M can be expressed in terms of density (ρ) and volume (V):
M=ρV=ρ(43πR3)
Substituting this into the equation for g′:
g′=Gρ(43πR3)R2=43GρR
Given that the density of the planet is 2ρE (where ρE is the density of Earth) and the radius is 12RE:
g′=43G(2ρE)(12RE)=43GρERE=gE
Thus, the acceleration due to gravity on the planet's surface is the same as that of Earth.
The gravitational potential at the surface is given by:
V′=−GMR
Substituting for M:
V′=−G(2ρE⋅43π(12RE)3)12RE
Simplifying:
V′=−G⋅2ρE⋅43π⋅18R3E12RE=−G⋅2ρE⋅43π⋅18R2E12=−G⋅2ρE⋅43π⋅14R2E1=−G⋅ρE⋅43πR2E1
This shows that the gravitational potential at the surface is the same as that of Earth.
The gravitational potential at the center of a solid sphere is given by:
Vc=−32GMR
For our planet:
V′c=−32G(2ρE⋅43π(12RE)3)12RE=−32⋅G⋅2ρE⋅43π⋅18R3E12RE
This simplifies to show that the gravitational potential at the center of the planet is two times that of Earth.
The gravitational field strength inside a solid sphere is zero at the center. Therefore, the gravitational field strength at the center of the planet is also zero, just like on Earth.
- (A) Acceleration due to gravity on this planet's surface → (q) Same
- (B) Gravitational potential on the surface → (q) Same
- (C) Gravitational potential at center → (r) Two times
- (D) Gravitational field strength at center → (s) Zero
Topper's Solved these Questions
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises
45 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - (A) Chapter Exercises
68 Videos
- GENERAL PHYSICSBOOK - DC PANDEYCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
- KINEMATICSBOOK - DC PANDEYCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
11 Videos
Similar Questions
Match the following :
Column -IColumn -II(A)Acceleration due to gravity(p)Kepler's law(B)Law of periods(q)Vector quantity(C)Orbital speed(r)Uniform near earth surface(D)Gravitational potential(s)Is nearly zero at infinity
View SolutionTwo concentric spherical shells are as shown in figure. Match the following columns
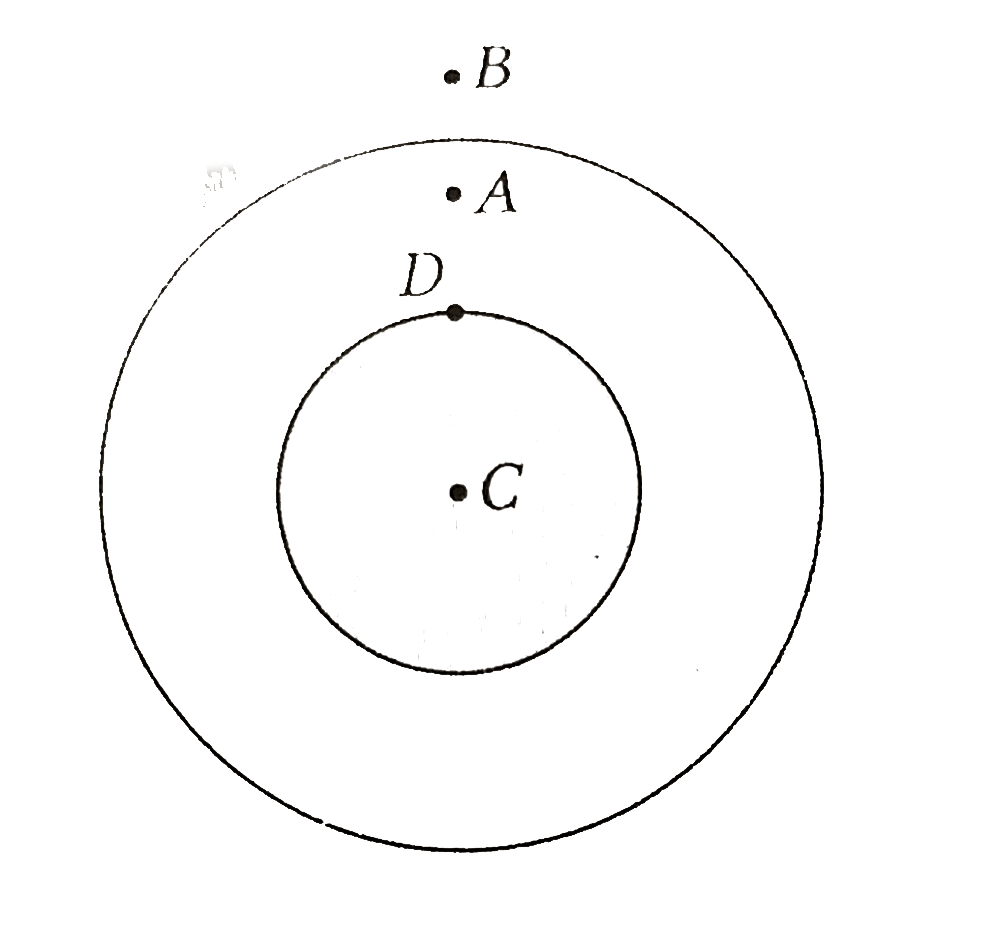
Column-IColumn-II(A)Potential at A(p)greater than B(B)Gravitational field at A(q)less than B(C)As one moves from C to D(r)Potential remains constant(D)As one moves from D to A(s)Gravitational field decreases(t)None
View Solution
Knowledge Check
If the density of the planet is double that of the earth and the radius 1.5 times that of the earth, the acceleration due to gravity on the planet is
A4/3 times that on the surface of the earthB3 times that on the surface of the earthC3/4 times that on the surface of the earthD6 times that on the surface of the earthThe escape velocity of a body at the surface of planet P is 1√5 times than that at surface of earth. The radius of planet is 124 times the radius of earth. The acceleration due to gravity for planet is
A2.4geB2.8geC4.8geD3.0geThe radius of a planet is n times the radius of earth (R). A satellite revolves around it in a circle of radius 4nR with angular velocity ω. The acceleration due to gravity on planet's surface is
ARω2B16 Rω2C32nRω2D64nRω2
In elliptical orbit of a planet, as the planet moves from apogee position to perigee position to perigee position, match the following columns
Column-IColumn-II(A)Speed of planet(p)Remains same(B)Distance of planet from centre of sun(q)Decreases(C)Potential energy(r)Increase(D)Angular momentum about centre of sun(s)Cannot say
View SolutionIf radius if earth is reduced to half without changing its mass, then match the following columns
Column-IColumn-II(A)Angular momentum of earth(p)Will become two times(B)Time period of rotation of earth(q)Will become four times(C)Rotational kinetic energy of earth(r)Will remain constant(D)(s)None
View SolutionMass and radius of a planet are two times the value of earth. What is the value of escape velocity from the surface of this planet?
View SolutionIf the dentist of a small planet is the same as that of earth, while the radius of the planet is 0.2 times that of the earth, the gravitational acceleration of the surface of that planet is :
View SolutionIf the dentist of a small planet is the same as that of earth, while the radius of the planet is 0.2 times that of the earth, the gravitational acceleration of the surface of that planet is :
View Solution
