y=x2−6x+8
The equation above represents a parabola in the xy-plane. Which of the following equivalent forms of the equation displays the x-intercepts of the parabola as constants or coefficients?

A

y−8=x2−6x

B

y+1=(x−3)2

C

y=x(x−6)+8

D

y=(x−2)(x−4)

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

Choice D is correct. The x-intercepts of the parabola represented by y=x2−6x+8 in the xy-plane are the values of x for which y is equal to 0. The factored form of the equation, y = (x − 2)(x − 4), shows that y equals 0 if and only if x = 2 or x = 4. Thus, the factored form, y = (x − 2)(x − 4), displays the x-intercepts of the parabola as the constants 2 and 4.
Choices A, B, and C are incorrect because none of these forms shows the x-intercepts 2 and 4 as constants or coefficients.

Updated on:21/07/2023
|
Class 12MATHSQUESTION BANK

Similar Questions

Knowledge Check

  • y=x2+16x+28
    The equation above represents the graph of a parabola in the xy-plane. Which of the following represents an equivalent form of the equation that includes the minimum value of y as a constant?

    Ay−28=x(x+16)
    By=x2+2(8x+14)
    Cy=x(x+16)+28
    Dy=(x+8)2−36

  • Which of the following is the graph of the equation y = 2x − 5 in the xy-plane?

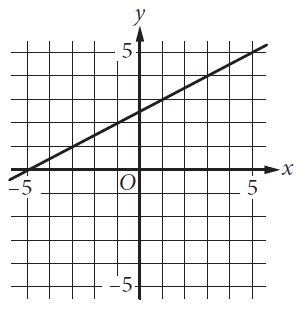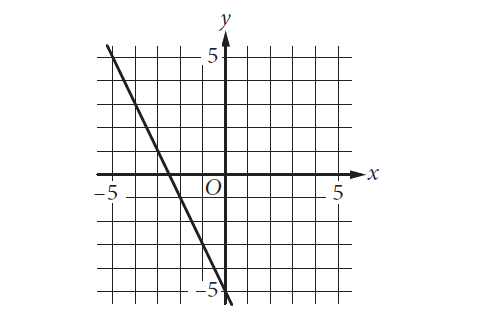    Aocr_image
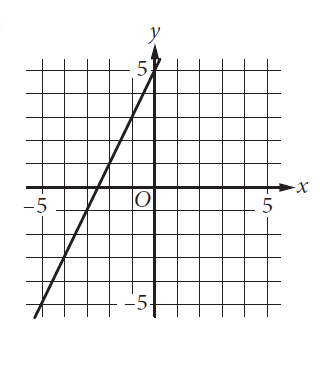    Bocr_image
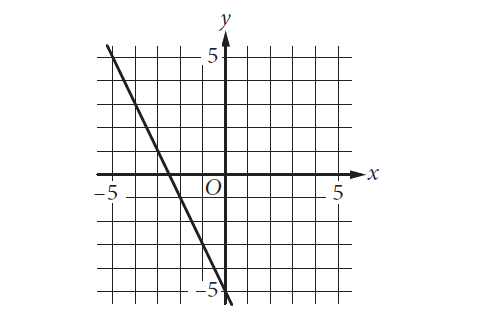
    Cocr_image
    Docr_image

  • f(x)=(x+6)(x−4)
    Which of the following is an equivalent form of the function f above in which the minimum value of f appears as a constant or coefficient?

    Af(x)=x2−24
    Bf(x)=x2+2x−24
    Cf(x)=(X−1)2−21
    Df(x)=(x+1)2−25