Question

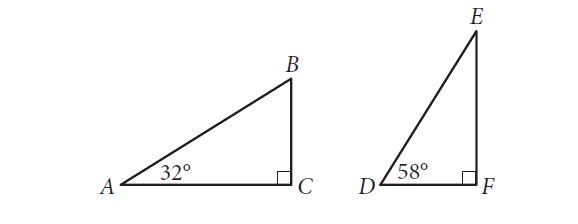
Triangles ABC and DEF are shown above. Which of the following is equal to the ratio $\frac{BC}{AB}$ ?

Options

A. `(DE)/(DF)`
B. `(DF)/(DE)`
C. `(DF)/(EF)`
D. `(EF)/(DE)`

Answer

B

Explanation

In right triangle ABC, the measure of angle B must be `58^@` because the sum of the measure of angle A, which is `32^@`, and the measure of angle B is `90^@`. Angle D in the right triangle DEF has measure `58^@`. Hence, triangles ABC and DEF are similar. Since BC is the side opposite to the angle with measure `32^@` and AB is the hypotenuse in right triangle ABC, the ratio `(BC)/(AB)` is equal to `(DF)/(DE)` .
Alternate approach: The trigonometric ratios can be used to answer this question. In right triangle ABC, the ratio `(BC)/(AB)=sin (32^@)`. The angle E is triangle DEF has measure `32^@` because `m(angleD)+m(angleE)=90^@`. In triangle DEF , the ratio `(DF)/(DE)=sin(32^@)`. Therefore , `(DF)/(DE)=(BC)/(AB)`. Choice A is incorrect because `(DE)/(DF)` is the inverse of the ratio `(BC)/(AB)`. Choice C is incorrect because `(DF)/(EF)=(BC)/(AC)`, not `(BC)/(AB)`. Choice D is incorrect because `(EF)/(DE)=(AC)/(AB)` , not `(BC)/(AB)`.