Question

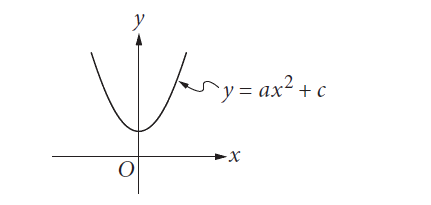
The vertex of the parabola in the xy plane above is (0, c). Which of the following is true about the parabola with the equation $y=-a{(x-b)}^2+c$ ?

Options

A. The vertex is (b, c) and the graph opens upward.
B. The vertex is (b, c) and the graph opens downward
C. The vertex is (−b, c) and the graph opens upward.
D. The vertex is (−b, c) and the graph opens downward.

Answer

B

Explanation

Since the shown parabola opens upward, the coefficient of `x^2` in the equation `y = ax^2 + c` must be positive. Given that a is positive, –a is negative, and therefore the graph of the equation `y = −a(x − b)^2 + c` will be a parabola that opens downward. The vertex of this parabola is (b, c), because the maximum value of y, c, is reached when x = b. Therefore, the answer must be choice B.
Choices A and C are incorrect. The coefficient of `x^2` in the equation`y = −a(x − b)^2 + c` is negative. Therefore, the parabola with this equation opens downward, not upward. Choice D is incorrect because the vertex of this parabola is (b, c), not (−b, c), because the maximum value of y, c, is reached when x = b.