Question

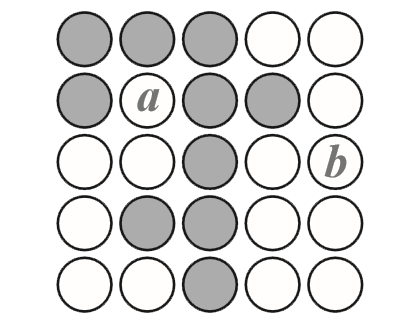
After leaving his island, Tintin seeked the help of the great scientist Dr. Calculus who stayed in his research island. When he landed on the island, he notices a map of 25 labs, aligned in a 5x5 pattern, of which a few labs were burned(Coloured in figure). Only 15 labs numbered from $\#1$ to $\#15$ are not burnt. He noticed the map, but realised that the lab numbers were missing from it. Instead, an instruction read “The distance from lab #1 to lab #2 will be less than the distance from lab $\#2$ to lab$\#3$, which is less than the distance from lab #3 to lab $\#4$, and so on”. Also, only the intact labs were numbered. Find out the value of a+b (where a and b are the lab numbers). Note : Distance is calculated from the centre of one lab to the centre of another.

Options

A. 10
B. 12
C. 14
D. 16

Explanation

If we see from the centre then the the maximum distance horizontally and vertically  can be 4 and minimum can be 0.  Possibilities A^2 + B^2 with  for finding the order :   X,Y Distances^2  0,1 01  1,1 02  0,2 04  1,2 05  2,2 08  0,3 09  1,3 10  2,3 13 3,3 18  0,4 16  1,4 17  2,4 20  3,4 25  4,4 32   
which are 14 possibilities and that means all will be used in this puzzle as no two distance  can be equal. 
So there will be 4,4 difference in 14,15... 3,4 in 13 ,14 and so on...
    Now we start from the sides and write variable for which positions are possible, then we  see different positions possible and continue till we reach one solution. WE go back from it  to fill all the remaining squares and we will get a=6 and b=10  Answer will be 16