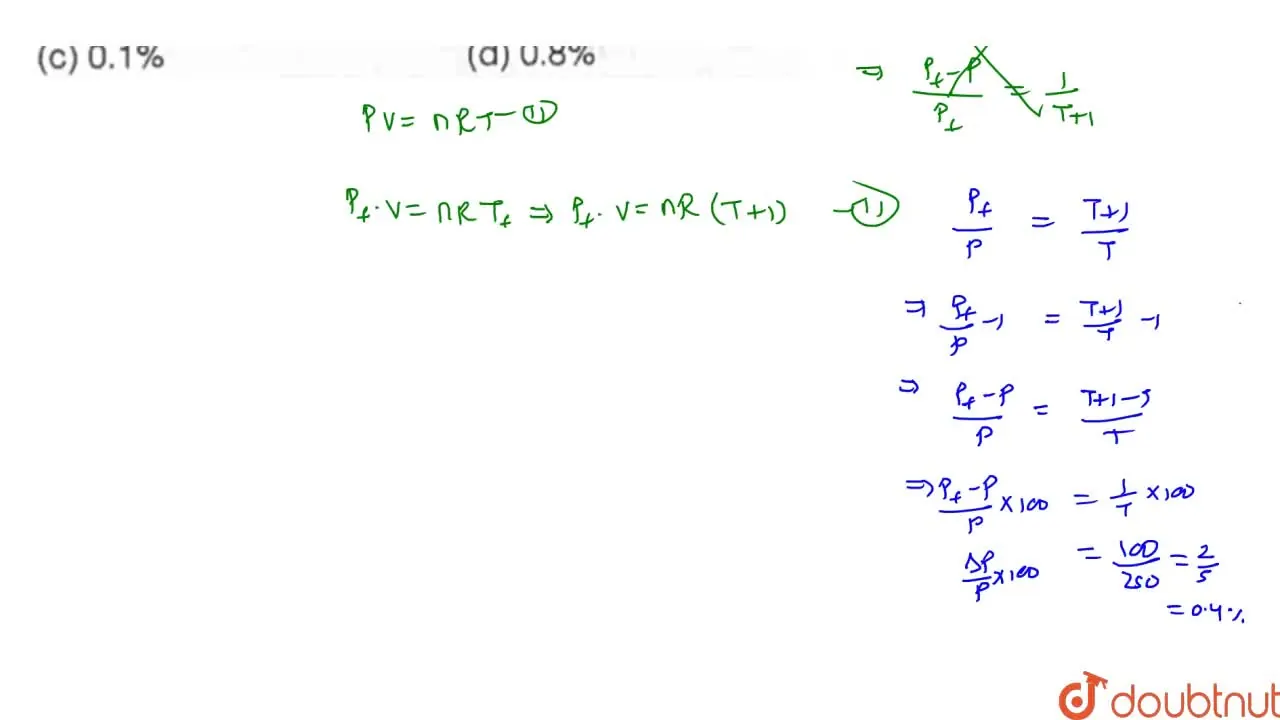A gas at the temperature 250 K is contained in a closed vessel. If the gas is heated through 1K, then the percentage increase in its pressure will be
0.4%
0.2%
0.1%
0.8%
The correct Answer is:A
Step by step video, text & image solution for A gas at the temperature 250 K is contained in a closed vessel. If the gas is heated through 1K, then the percentage increase in its pressure will be by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
Similar Questions
A gas at a temperature of 250K is contained in a closed vessel. If the gas is heated through 5.46° C, the percentage Increase in its pressure is nearly
View SolutionA gas at a temperature of 250K is contained in a closed vessel.If the gas is heated through 5.46∘C ,the percentage increase in its pressure is nearly
View SolutionIn a closed vessel, a gas is heated from 300 K to 600 K the kinetic energy becomes/remain
View SolutionIn a closed vessel, a gas is heated from 300K to 600K the kinetic energy becomes/remains
View SolutionThe temperature of a gas contain in a closed vessel increased by 20C when the pressure is increased by 2% the intial temperature of the gas is
View SolutionIf the pressure of a gas contained in a closed vessel increases by X% when heated bu 10C, it initial temperature is
View SolutionThe temperature of a gas contain in a closed vessel increased by 20C when the pressure is increased by 2% the intial temperature of the gas is
View SolutionWhen a gas filled in a closed vessel is heated through 1∘C , its pressure is increased by 0.4 % . The initial temperature of the gas was
View SolutionThe initial temperature of a gas is 100∘C . The gas is contained in a closed vessel . If the pressure of the gas is increased by 5% , calculate the increase in temperature of the gas :
View SolutionThe initial temperature of a gas is 100∘C. The gas is contained in closed vessel. If the pressure on the gas is increased by 5% calculate the increase in temperature of the gas
View Solution