In the $s - t$ equations $(s = 10 + 20 t - 5 t^{2})$ match the following

Video Solution

Text Solution

Verified by Experts

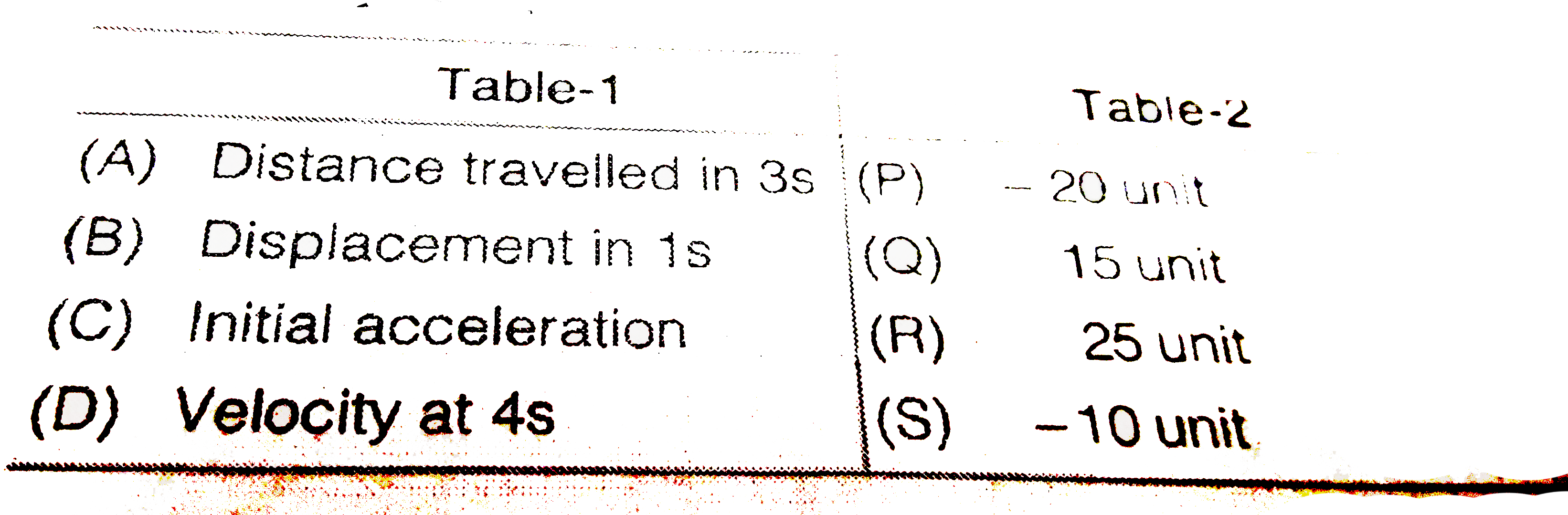
The correct Answer is:A, B, C, D

Updated on:21/07/2023

|

Answer

Step by step video, text & image solution for In the s-t equations (s=10+20t-5t^(2)) match the following by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 PHYSICS RECTILINEAR MOTION

Topper's Solved these Questions

KINEMATICS 1BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
KINEMATICS 1BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - COMPREHENSION_TYPE
19 Videos
KINEMATICSBOOK - DC PANDEYCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
11 Videos
LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Medical entrances gallery
39 Videos

Similar Questions

Knowledge Check

The maximum height is reached is $5 s$ by a stone thrown vertically upwards and moving under the equation $10 s = 10 u t - 49 t^{2}$ , where $s$ is in metre and $t$ is in second. The value of $u$ is
A $4.9 m s^{- 1}$
B $49 m s^{- 1}$
C $98 m s^{- 1}$
DNone of these
Factorise:
$- 5 - 10 t + 20 t^{2}$
A $- 5 (5 + 2 t - 4 t^{2})$
B $- 10 (1 + 2 t - 4 t^{2})$
C $- 5 (1 + 2 t - 4 t^{2})$
D $- 5 (1 + 2 t - 10 t^{2})$
Displacements is related with timet in the equation $s = 5 t^{2} + 4 t + 3$ . The acceleration is equal to
A10 units
B5 units
C4 units
D3 units

DC PANDEY-KINEMATICS 1-MATCH THE COLUMN

Home
Ask Doubt
Profile